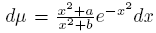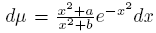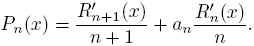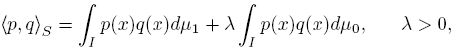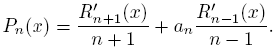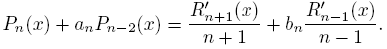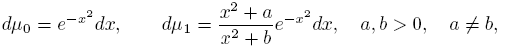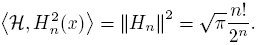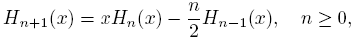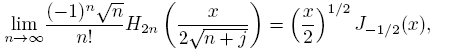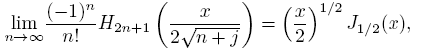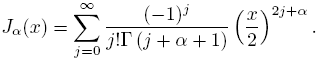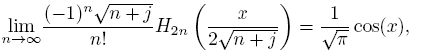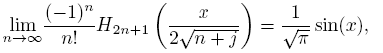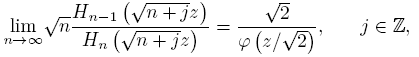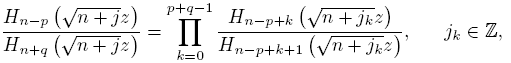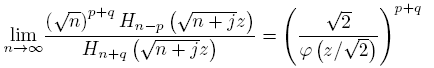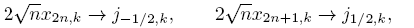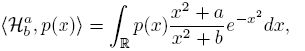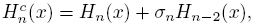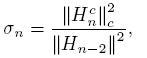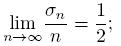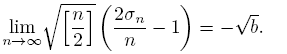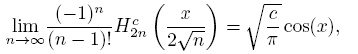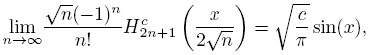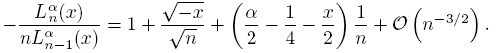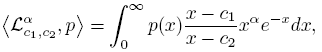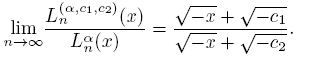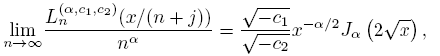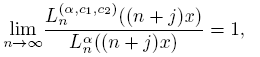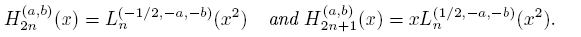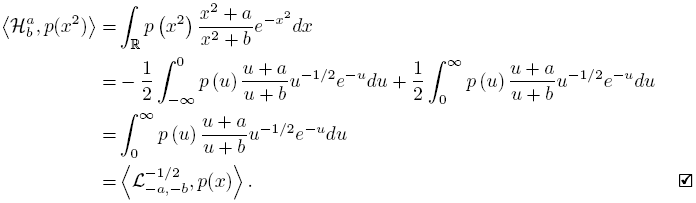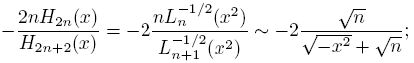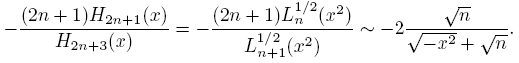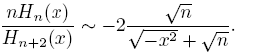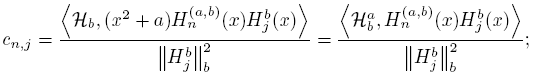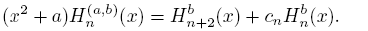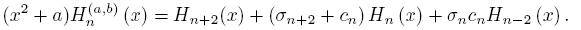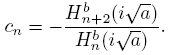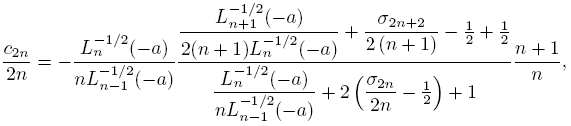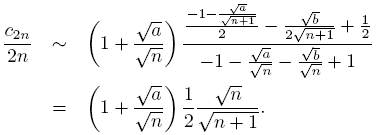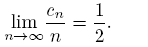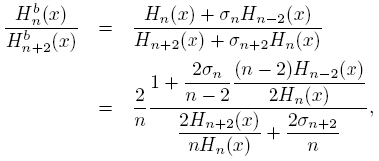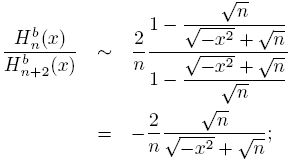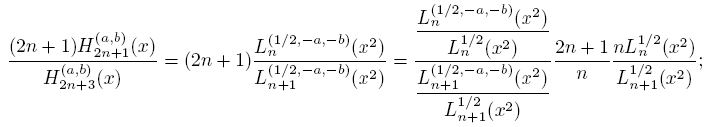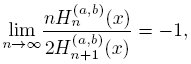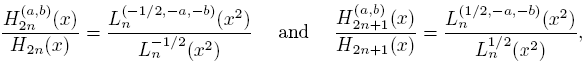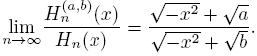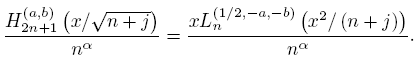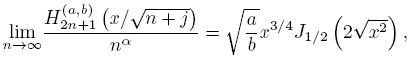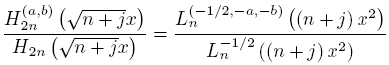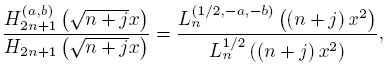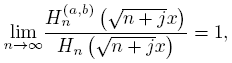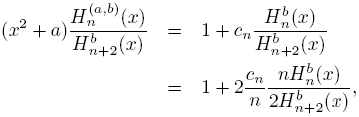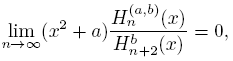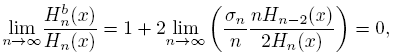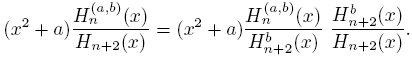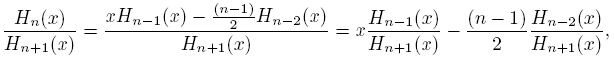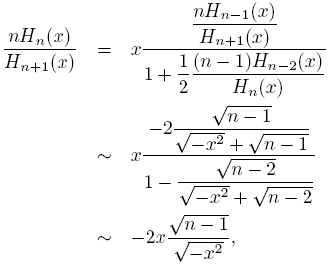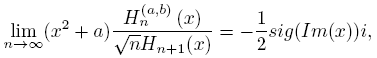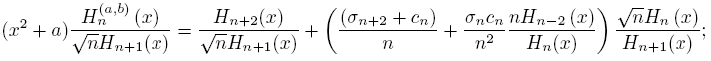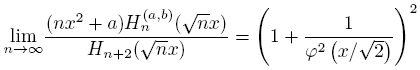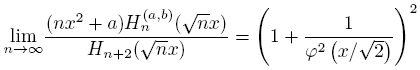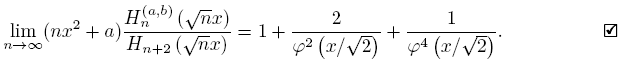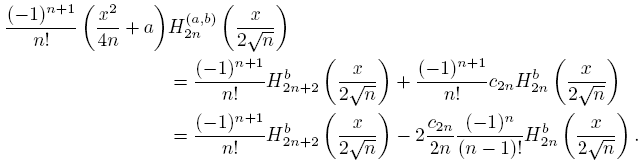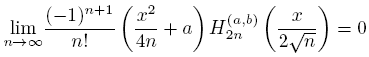1. Introduction

Let U and V be quasi-definite linear functionals and let {P_n } _n∈N and {R_n } _n∈N be their sequences of monic orthogonal polynomials (SMOP), respectively. (U, V ) is a coherent pair if there exists a sequence (a_n ), a_n ≠ 0, such that

This concept is introduced in [⁷] where it is studied its connection with polynomials orthogonal with respect to Sobolev inner products like

where μ ₀ and μ ₁ are positive Borel measures supported on an infinite subset I ⊆ R, with U and V as the associated functionals, respectively. In this way, among others, it is studied an algebraic connection between the Sobolev polynomials and the sequence {R_n } _n∈N , in such a way that the coefficients of connection are independent of the degree; an algorithm is presented for to compute Fourier coefficients using as basis the Sobolev polynomials. Likewise, if U and V are symmetric, (U, V ) is a symmetric coherent pair if there exists a sequence (a_n ) , a_n ≠ 0, such that

In [⁸] all coherent pairs and symmetric coherent pairs are determined and it is shown that at least one of the functionals has to be classical (Hermite or Gegenbauer in the symmetric case); moreover, if ξ > 0, the symmetric coherent pair dμ ₀ = e ^−x2 dx, 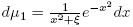is obtained. In connection with this particular case, in [²] the outer relative asymptotics of Sobolev polynomials orthogonal with respect to (1) is found; besides, in [⁹] Mehler-Heine type formulas are established with respect to rational modification of the Hermite polynomials. Under the same assumptions, (U, V ) is a symmetric (1, 1)−coherent pair if there exist sequences (a_n ) _n∈N and (b_n ) _n∈N , b_n ≠ 0, such that the respective SPOM satisfies

About this subject, in [⁴] is presented the algebraic relation between the Sobolev polynomials and the polynomials {R_n } _n∈N ,; besides, the particular case where V is classical is studied, and the respective symmetric (1, 1)−coherent companion is found. In particular, we focus on the symmetric (1, 1)−coherent pair

and we will study the asymptotic behavior of the orthogonal polynomials associated with dμ ₁. Thus, the structure of this manuscript is as follows: In the section 2 we present some basic facts about of asymptotic behavior of Hermite orthogonal polynomials. In section 3 we present an algebraic connection between the Hermite polynomials and the SMOP associated with dμ ₁, as well as the asymptotic behavior of the respective connection coefficients. Finally, in section 4 some asymptotics properties are studied.

2. Preliminaries

From now on, and as it is usual, {H_n } _n∈N will represent the sequence of monic Hermite polynomials, orthogonal with respect to the weight e ^−x² on (−∞,∞). The classical Hermite linear functional will be denoted by H and 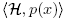will be the application of H on any polynomial p. The norm of the monic Hermite polynomials is defined as

On the other hand the sequence {H_n } _n∈N is defined via the three terms recurrence relation

with the initial conditions H ₀(x) = 1 and H ₋₁(x) = 0. With respect to the asymptotic behavior of Hermite polynomials we present the next results.

Theorem 2.1 (See [¹⁰]).

uniformly on compact sets of C\R.

Theorem 2.2 (Mehler-Heine). (See [¹]). For j ∈ Z fixed,

and

uniformly on compact sets of the complex plane, where J_α represents Bessel’s function of the first kind defined by

Theorem 2.3 (Mehler-Heine). (See [¹⁰]).

and

uniformly on compact subsets of C and uniformly on j ∈ N ∪ {0} .

Theorem 2.4 (See [¹¹]). For j ∈ Z fixed,

holds uniformly on compact subsets of C\ [−√2,√2]. Here, φ (z) = z + √z ² − 1 is the conformal mapping of C\ [−1, 1] onto the exterior of the closed unit disk.

If i = 0 and if p and q are non-negative integers such that n > p − 1, then

and as a consequence,

The above proves the next

Corollary 2.5. For j ∈ Z fixed, and non-negative integers p and q such that n > p − 1,

holds uniformly on compact subsets of C\ [−√2,√2].

The zeros of H_n are real, simples and symmetric; that is, for every n, Hn(t) = 0 is equivalent to H_n (−t) = 0. Let {x_{n ,k} }^[n/2] _n=1 be the positive zeros of H_n in increasing order. It is well known that the zeros of H_n and H_{n −1} are interlaced and for k fixed, x_{n ,k} → 0 when n → ∞. Besides, given that J_α has a countably infinite set of real and positive zeros if α > −1, as a consequence of Mehler-Heine formulas and the Hurwitz’s theorem, if n → ∞ and k ≥ 1 then

and 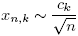, where c_k > 0 and {j_{α ,k} } _n∈N are the zeros of J_α when α > −1.

On the other hand, let {H_n ^(a,b)} _n∈N be the sequence of monic polynomials orthogonal with respect to the positive definite linear functional H^a _b , defined as

where a, b > 0, and a ≠ b. As it is usual, let ║.║_(a,b) be the induced norm. If c > 0, then {H^c _n } will be the sequence of monic polynomials orthogonal with respect to the positive definite functional H_c defined by 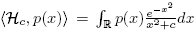, and ║.║ _c the respective induced norm. On the algebraic connection between the sequence {H^c _n } and the classical Hermite polynomials we get the next result.

Lemma 2.6 (See [²]). There exists a sequence of real numbers (σ_n ) such that

with

and

moreover,

With respect to asymptotic behavior and Mehler-Heine type formulas for the sequence {H^c _n } _n∈N , we get the next

Theorem 2.7 (See [⁹]).

and

both uniformly on compact subsets of C.

Let L^α be the classical Laguerre functional, α > −1, and let {L^α _n (x)} be the respective SMOP. We present the next result about asymptotics behavior of ratios of Laguerre polynomials that will be necessary in our work. The proof can be see in [⁵].

Lemma 2.8. For x ∈ C\R⁺,

In this paper also will be important to deal with rational perturbations of the L^α and the asymptotic behavior of the associated SPOM. About this topic, in [⁶] is made an exhaustive study of asymptotic behavior of orthogonal polynomials associated to this kind of perturbations. Indeed, given c ₁, c ₂ < 0, let {L_n ^{(α,c₁,c₂)} (x)} be the SMOP associated to positive definite linear functional L^α _{c ₁,c₂} defined on the space of polynomials as

and about the asymptotic behavior of the sequence {L_n ^{(α,c₁,c₂)} (x)},we get the next

Theorem 2.9 (See [⁶]).

a). Uniformly on compact subsets of C\[0,∞),

b). Uniformly on compact subsets of C,

where j ∈ N ∪ {0} .

c). (Plancherel-Rotach type exterior asymptotics).

uniformly on compact subsets of C\[0, 4], and uniformly on j ∈ N ∪ {0} .

3. Some basic results

Given that H is a symmetric linear functional, it is well known that there is a relation between the classical Laguerre and Hermite polynomials, namely

In this way, the next result is an extension of the above relations.

Lemma 3.1. For every n ∈ N,

Proof. Given that H^a _b is symmetric, there exists an unique quasi-definite linear functional v, with {P_n } as the associated SPOM and such that H _2n ^(a,b) (x) = P_n (x ²) and H ^(a,b) _2n+1(x) = xP_n (x ²) (see [³]), moreover, 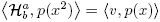.We will see that v = L ^−1/2 _−a,−b . Indeed, with the change of variable u = x ² we get

On the other hand, from (22) we have 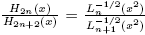, and given that (see (18)) 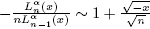, we get

in the same way, in the odd case we get

Then we have deduced the next

Lemma 3.2. For x ∈ C\R we get

By using of {H^b _n } _n∈N as a basis we get

where

then, c_{n ,j} = 0, for j = 0, ..., n − 1, and 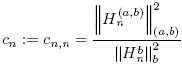. So we get

As a consequence of (12), we have

In order to obtain the behavior limit of the sequence (c_n ), we choose x = i√a in (25), and then for every n

By using (12) we get

In the even case,

From (12) we obtain

and by using of (18) we get

Given that H _2n+1(x) = xL_n ^1/2 (x ²), and following the same arguments in the odd case for the subsequence 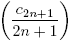, we get the next

Lemma 3.3.

4. Asymptotic Properties

We want to obtain formulas of the kind (4) associated to the ratios 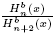and 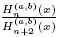. First, we will deduce the next

Lemma 4.1.

uniformly on compact sets of C\R.

Proof. From (12), we get that

and by using of (24) we get

then the result follows.

In the above result we have obtained a formula of the type (4) for the sequence {H^b _n } _n∈N . Now we will get the same one for the sequence {H_n ^(a,b)} _n∈N. Indeed, in the odd case and from (23) we get

as before, the even case is similar. Then, as a consequence of (18) and (19) we get the next

Proposition 4.2.

uniformly on compact subsets of C\R.

Moreover, from (23) we get

and as a consequence of (19) we obtain

Lemma 4.3. Uniformly on compact subsets of C\R,

On the other hand, scaling the variable, and by (23), we get

and

And from (20) we deduce

Lemma 4.4. Uniformly on compact subsets of C,

and

where j ∈ N ∪ {0} .

Finally, given that

and

as a consequence of (21) we have

Lemma 4.5 (Plancherel-Rotach type exterior asymptotics).

uniformly on compact subsets of C − [−2, 2], and uniformly on j ∈ N ∪ {0} .

In our search of information about of asymptotic behavior of the sequence {H_n ^(a,b)} _n∈N, it is very useful to consider the sequence {(x ² + a)H_n ^(a,b)} and the results obtained in the above lemmas. In this way, using (25) we obtain

then, the next proposition is a consequence of (27) and (28).

Proposition 4.6. The sequence {H_n ^(a,b)} satisfy

uniformly on compact sets of C\R.

Naturally, it is possible to obtain a similar result for the ratio 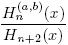. It is enough to consider (4) and (12) to obtain

uniformly on compact sets of C\R, and to use the above theorem for the expression

On the other hand, using recurrence relation (3) we get

and as a consequence, 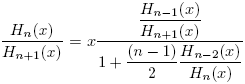. As before, for x ∈ C\R, and from (24), it follows

and as a consequence, 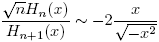. Then we get the next

Lemma 4.7.

uniformly on compact subsets of C\R.

Now, we will see the importance of above lemma in the following theorems.

Theorem 4.8.

uniformly on subsets compact of C\R.

Proof. From (26) we get

then, using (4) and (29) the result holds.

Theorem 4.9 (Scaled Relative Asymptotics).

holds uniformly on compact subsets of C\ [−√2,√2].

Proof. Making the scaling x → √nx in (26), we have

Then, using (9) and (14) we obtain

Now we will deduce Mehler-Heine type formulas for the polynomials {H_n ^(a,b)}. Making 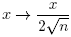in (25), for the even case we get

Then, using (16) and following a similar procedure in the odd case, we get the next

Proposition 4.10 (Mehler-Heine type formulas).

and

uniformly on compact subsets of the complex plane.