Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Cited by Google
Cited by Google -
 Similars in
SciELO
Similars in
SciELO -
 Similars in Google
Similars in Google
Share
DYNA
Print version ISSN 0012-7353On-line version ISSN 2346-2183
Dyna rev.fac.nac.minas vol.78 no.166 Medellín Apr./June 2011
GENERALIZATION OF ZAD STRATEGY: AN APPLICATION TO A DC-DC BUCK CONVERTER
GENERALIZACIÓN DE LA ESTRATEGIA ZAD: APLICACIÓN A UN CONVERTIDOR REDUCTOR DC-DC
FABIOLA ANGULO GARCIA
Departamento de Ingeniería Eléctrica, Electrónica y Computación, Universidad Nacional de Colombia Sede Manizales fangulog@unal.edu.co
GERARD OLIVAR TOST
Departamento de Ingeniería Eléctrica, Electrónica y Computación, Universidad Nacional de Colombia Sede Manizales golivart@unal.edu.co
GUSTAVO ADOLFO OSORIO LONDOÑO
Departamento de Ingeniería Eléctrica, Electrónica y Computación, Universidad Nacional de Colombia Sede Manizales gaosoriol@unal.edu.co
Received for review January 29th, 2010, accepted October 13th, 2010, final version October, 23th, 2010
ABSTRACT: The Zero Average Dynamics (ZAD) strategy has been reported in the last decade as an alternative for controlling power converters. This technique has the advantage of guaranteeing fixed frequency switching. However, the stability of the controller is highly dependent on the load value, and when the stability is lost, the fixed frequency switching is lost too. In this paper we generalize ZAD strategy using the probabilities framework through the expectation operator. Thus, we recover classical sliding mode control classical ZAD strategy, and new control methods can be defined, which are more stable than the others previously used. For this reason, this technique is entitled Generalized Zero Average Dynamics (GZAD). We will show several simulations regarding an application to a DC-DC Buck converter within the generalized ZAD strategy, which cannot be deduced from the classical ZAD. Numerical simulations show good regulation features and a wide range of stability.
KEY WORDS: ZAD control, GZAD control, bifurcation analysis, power electronic circuits, power converters, control of power converters.
RESUMEN: En la última década se ha reportado la estrategia de control Zero Average Dynamics (ZAD) como una alternativa al control de los convertidores de potencia, garantizando frecuencia fija de conmutación y bajo error. Sin embargo la estabilidad del sistema depende fuertemente de la carga, por lo cual no es robusto. Cuando se pierde la estabilidad la frecuencia fija de conmutación se pierde también. En este artículo se presenta la generalización de la técnica ZAD dentro del marco de la teoría de probabilidades. Usando este marco es posible recuperar el ZAD tal como ha sido analizado hasta ahora y se pueden generar nuevas estrategias de control, las cuales son más estables que las usadas en el ZAD. Por este motivo a esta técnica se le ha llamado Generalized Zero Average Dynamics (GZAD). Presentamos algunas simulaciones del GZAD aplicado a un convertidor de potencia reductor. Esta nueva estrategia no puede ser obtenida con el ZAD clásico. Las simulaciones numéricas muestran buen desempeño del controlador con bajo error de regulación y robustez ante cambio en la carga.
PALABRAS CLAVE: Control ZAD, Control GZAD, Análisis de bifurcaciones, convertidores de potencia, control de convertidores.
1. INTRODUCTION
This paper is devoted to the analysis of the regulation problem in ZAD controlled buck power converters. Power converters have a lot of applications in industrial and commercial environments, and in electrical household appliances (see, for example [1]). There are several basic configurations such as buck (or step down), boost (or step up), buck-boost, and so on. Besides, these configurations can be used for tracking or regulations tasks. A complete introduction to power converters can be found in [2] and a more concise overview including control, in [3]. Nonlinear phenomena in power electronics, bifurcations, chaos, and control of chaos are widely reported in [4], and more recently in [5-6]. Two-parameter bifurcation diagrams of DC/DC converter topologies have been recently reported in [7-10].
In the last decade, a new control strategy, the so-called ZAD control, has been widely applied and analyzed for regulation problems in a step down power converter, that is, to keep the output voltage of a converter close to a desired reference voltage in buck converters. This control scheme (called classical ZAD strategy in this paper) proposed in [11], conjugates the advantages of fixed frequency implementations and the inherent robustness of sliding control modes. It is based on an appropriate design of the duty cycle in such a way that, in each sampling period, the sliding surface average is zero, and at the same time the output of the system tracks the reference value with a very low voltage error. Numerical [12,13], analytical [12-14], and experimental results [15-17] have shown the effectiveness of this technique. However, due to the switching control, the converter displays a plethora of nonlinear behavior after the system loses stability and fixed frequency. In [13-14,18-21] a wide study of nonlinear phenomena has been reported. Other strategies with PWM and Sliding Mode can be found in [22].
The continuous version of the classical ZAD strategy is based on sliding modes, and it forces a dynamical error to be zero in each sampling time. With this technique there are two problems: the first problem is manifested due to a kind of rigidity of the method. At each sampling interval, and when the control variable takes one of its values (+1), the strategy proposed in [11] gives the same weight all along the period. This will become clearer in the corresponding section. The second problem occurs from a practical point of view: the computation of the duty cycle may be slow. The simplified version in [11-12] considers the dynamical expression in the error as a piece-wise linear function with the aim of simplifying the computations [12]. However, this simplified new version (or discrete version) of the classical ZAD strategy gives also the same weight to the measured values for computing the duty cycle. Thus, we propose a generalization of this technique using the probabilities framework through the expectation operator. Our main aim is to show that a simple idea like introducing the appropriate mathematical framework can lead to a generalization of the ZAD control strategy that can produce better results than classical ZAD. Through this method we can recover the known classical continuous and discrete ZAD techniques, and furthermore, we can state new ones which enhance some methods in the literature. Thus we will first state the mathematical framework and, later, we will apply this set of techniques to a case of study: the regulation problem for a DC-DC Buck converter.
In this paper we consider different weights for the variables in the dynamical expression, which take discrete values, and we generalize the ZAD technique in such a way that we can recover the classical sliding mode control, the classical continuous ZAD and, the classical discrete ZAD strategies. Even though the probabilities mathematical framework looks a bit unusual in the analysis of DC-DC converters, we propose to use the expression of the duty cycle given by our methods instead of the already classical ZAD reported procedures. The new proposed controller is more stable than the classical ZAD controller and with it the fixed frequency switching is not lost. On the other hand, the practical implementation requires the same technological development as the reported classical ZAD strategy.
This paper is organized as follows: In Section 2, we develop the generalization of ZAD strategy for continuous and discrete cases. In Section 3 we analyze the design of the control strategy for the continuous case. Section 4 is devoted to showing the design of the control strategy for the discrete case. Both sections 3 and 4 are illustrated with a DC-DC Buck power converter. Section 5 includes a detailed design for controller based on GZAD technique, and finally, in Section 6 we state some conclusions and future work.
2. GENERALIZED ZERO AVERAGE DYNAMICS (GZAD)
Zero Average Dynamics (ZAD) has been recently proposed in the literature [11-12,15-16,23], and it has been applied to several DC-DC converters [15,17,24]. In this section, we generalize this technique and several different control strategies are obtained as particular cases.
2.1 Probabilities mathematical framework
PWM methods are very much related to sliding mode control [25] and, concretely, the ZAD strategy is related to it as well, as follows. Given a control system
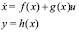 (1)
(1)
where x is the vector of state variables, and u is the control signal, a sliding surface is defined as
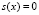 (2)
(2)
and a general control strategy is to find u in such a way that the system evolves on the surface. This is basically the so-called sliding-mode control. Generally speaking, the ZAD technique is a control strategy which is softer than the sliding mode control, in the sense that only
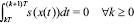 (3)
(3)
is imposed for every interval of fixed length T.
The main idea of the new GZAD is to assume that there is a density function defined on [0,T] (which is equivalent of saying that x(t) is a random variable with a certain distribution, or {x(t)}t≥0 a stochastic process), and forcing the expectation of s(x(t)) to be zero.
Let q(t) be a density on the real line. Then one can easily define a density on [0,T] by
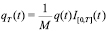 (4)
(4)
where I is the indicator function (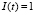 if
if 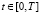 , and
, and 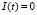 otherwise) and
otherwise) and
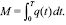 (5)
(5)
Then, the expectation of s(x(t)) is computed as
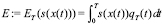 (6)
(6)
and 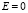 (7)
(7)
is imposed in the GZAD strategy. One can recover the classical ZAD strategy with a 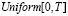 distribution. Considering different distributions, like a
distribution. Considering different distributions, like a 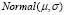 or
or  , one obtains different control strategies, depending on the importance given to some sets in the interval [0,T]. In the following section, we explain the continuous and discrete cases.
, one obtains different control strategies, depending on the importance given to some sets in the interval [0,T]. In the following section, we explain the continuous and discrete cases.
2.2 Continuous case: normal and exponential strategies
The density of a Normal distribution with µ mean and 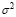 variance is given by
variance is given by
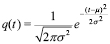 (8)
(8)
In this case we should force
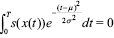 (9)
(9)
In practical cases, where x(t) contains exponential and trigonometric functions, it is clear that (9) can only be solved numerically and the computations can be heavy for experimental prototypes. Thus although this distribution can be thought of as an academic example, from a practical point of view this distribution function may not be good.
Instead, if we consider the Exponential distribution with parameter b, whose density is
 (10)
(10)
then we have to force
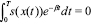 (11)
(11)
For several applications, this integral will not be solved analytically, but with a good selection of parameter b it is possible to reduce the complexity of the expression for the solution. In such a case, better stability results are obtained, as is shown in the bifurcation diagrams (Figs. 1 and 2). As the ZAD technique can be used in practical applications such as those reported in [15, 17], the main idea is to find a simple form to compute the duty cycle, which guarantees stability and does not require much computational effort. In this section, we relax the condition of zero value of the integral on the interval [0,T] through a selection of some points, and we force the sum (instead of the integral) to be zero.
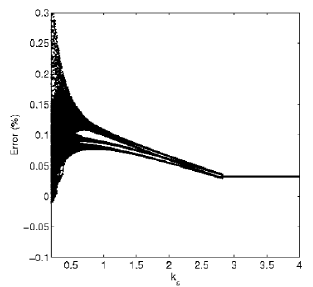
Figure 1. Bifurcation diagram of the voltage error using ks as bifurcation parameter. Classical ZAD strategy: continuous uniform density
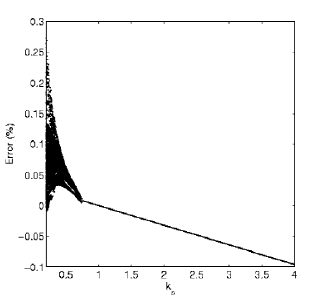
Figure 2. Bifurcation diagram of the voltage error using ks as bifurcation parameter. GZAD strategy: exponential density
2.3 The case of discrete random variables
When we have a discrete random variable, some interesting new strategies appear. Let us write the density as a finite combination of Delta functions,
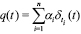 (12)
(12)
where all 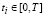 ,
, 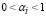 , for
, for 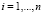 , and
, and
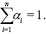 (13)
(13)
Then the expectation is
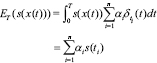 (14)
(14)
and it is zero when
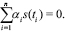 (15)
(15)
When n = 1, this is reduced to s(t1) = 0 for a chosen 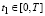 . This law is simpler than ZAD and, moreover,
. This law is simpler than ZAD and, moreover, 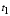 can be chosen in such a way that it minimizes some additional error function, improving the control design. In the case n = 2, we have for some
can be chosen in such a way that it minimizes some additional error function, improving the control design. In the case n = 2, we have for some 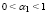 ,
,
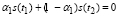 (16)
(16)
and thus
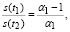 (17)
(17)
and, in general, for  we have
we have
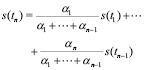 (18)
(18)
for 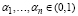 , such that
, such that 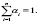 If we choose
If we choose 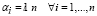 (a discrete uniform distribution), we recover the discrete version of the classical ZAD technique. Also, in some cases, it can be useful to apply GZAD to several surfaces
(a discrete uniform distribution), we recover the discrete version of the classical ZAD technique. Also, in some cases, it can be useful to apply GZAD to several surfaces 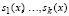 if we need the system, for design purposes, to evolve close to the intersection of the surfaces. This will be studied in a further paper. In Sections 3, 4, and 5, we will use our knowledge of the classical ZAD strategy and we will improve its performance.
if we need the system, for design purposes, to evolve close to the intersection of the surfaces. This will be studied in a further paper. In Sections 3, 4, and 5, we will use our knowledge of the classical ZAD strategy and we will improve its performance.
3. THE DESIGN OF THE CONTROL STRATEGY: UNIFORM (ZAD) AND EXPONENTIAL (GZAD) DISTRIBUTIONS.
With the aim of generalizing the ZAD technique, we consider a system defined by (19), with γ > 0, which corresponds to any two-order stable linear system with relative degree two and unitary gain (a complete procedure for this transformation can be found in [26]).
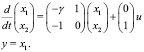 (19)
(19)
The control variable is 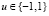 . A centered PWM u is given by
. A centered PWM u is given by
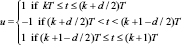 (20)
(20)
Where d is the normalized duty cycle. The objective is to find a control law in such a way that the output variable (y) remains close to a fixed desired value with low voltage error (regulation task) and the system is robust. To satisfy these requirements, and using the main ideas of sliding control, the following function is defined [27]:
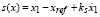 (21)
(21)
where x1 is the variable to be controlled and ks is the time-constant associated with the error dynamics. Because of the similarity with sliding modes, s(x) is also called sliding surface.
The proposed ZAD strategy is based on computing a time instant d, such that in each period T, the following equation is satisfied
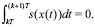 (22)
(22)
Thus s(x(t)) is forced to have zero average in each period. As we stated previously, the reported ZAD strategy is based on a continuous uniform distribution, in such a way that (22) is satisfied in each period. Using an exponential distribution, we force the system to satisfy
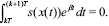 (23)
(23)
With the aim of testing our generalization of the ZAD strategy, we compute the duty cycle with continuous uniform (classical ZAD strategy) and exponential densities (GZAD) using b = 1. Figures 1 and 2 show the bifurcation diagram of both strategies for γ = 0.35. The fixed point loses stability for ks » 2.8 with the classical ZAD strategy, while the point loses stability for ks » 0.743 with GZAD. Thus the generalized method shows that the stability range has been enlarged. The voltage error, shown at the sampling times  is very low in both methods.
is very low in both methods.
It is worth noting that quantitative agreement with the voltage error is hard to obtain in practical applications mainly due to problems related to the sensitivity of sensors, delays, and so on. Therefore, the advantage of GZAD strategy is the widening of the stability range. However, the main problem of this technique, as it is also in the case of the classical continuous ZAD strategy, is that it is still necessary to solve a transcendental equation for finding the duty cycle in each period. For solving this situation, we proceeded to define the generalized ZAD discrete version.
4. THE DESIGN OF THE CONTROL STRATEGY FOR DISCRETE RANDOM VARIABLES: DISCRETE ZAD AND DISCRETE GZAD
For the classical ZAD strategy a practical handicap for experimental validation is the heavy computation of the duty cycle at each sampling interval. This is due to the fact that, at each sampling time 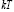 , a transcendental equation must be solved. In order to avoid this problem, in [11, 12] a piece-wise linear function
, a transcendental equation must be solved. In order to avoid this problem, in [11, 12] a piece-wise linear function  was introduced (which approximates quite well the original
was introduced (which approximates quite well the original  ), and instead of solving
), and instead of solving 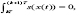 it was forced that
it was forced that 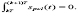
Function  was defined by three linear pieces and the slopes of these pieces where
was defined by three linear pieces and the slopes of these pieces where 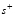 was in the first piece,
was in the first piece, 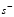 in the second one, and
in the second one, and 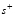 again in the third one. So,
again in the third one. So, 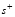 is computed as the slope of the function
is computed as the slope of the function  at the beginning of the sampling interval assuming that u = +1, while
at the beginning of the sampling interval assuming that u = +1, while 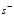 is computed as the slope of the function
is computed as the slope of the function 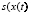 at the beginning of the sampling interval, assuming that
at the beginning of the sampling interval, assuming that 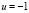 . Concretely,
. Concretely,
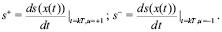
Then, we assume that the first piece is defined in 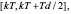 the second piece in
the second piece in 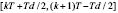 and the third one in
and the third one in 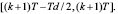 Thus we compute the normalized duty cycle
Thus we compute the normalized duty cycle 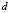 in such a way that the following equation is satisfied:
in such a way that the following equation is satisfied:
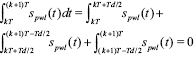 (24)
(24)
Then the duty cycle obtained is [12]:
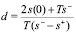 (25)
(25)
where 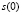 is the value of
is the value of 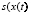 in
in 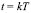 .
.
Since d must be in the interval  , we saturate the expression in (25) by 1 if it exceeds 1, or we saturate it to 0 if it is below 0. This is the simplest case, and it is called classical discrete ZAD.
, we saturate the expression in (25) by 1 if it exceeds 1, or we saturate it to 0 if it is below 0. This is the simplest case, and it is called classical discrete ZAD.
In [12, 13] it was proven that considering 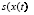 as a piece-wise linear function, for γ = 0.35 and
as a piece-wise linear function, for γ = 0.35 and  , the system defined by (19), (20) and (25) has a stable T-periodic orbit for ks » > 3.25. For this value the system has a period-doubling bifurcation followed by a corner collision bifurcation (at ks » 3.24), followed by a second period-doubling bifurcation (for ks » 3) and a second corner collision (for ks » 2.999). After these bifurcations, chaotic bands appear and the process continues until the system enters in 1-band chaos (for ks » 0.5). Details of this bifurcation process can be found in [13, 18].
, the system defined by (19), (20) and (25) has a stable T-periodic orbit for ks » > 3.25. For this value the system has a period-doubling bifurcation followed by a corner collision bifurcation (at ks » 3.24), followed by a second period-doubling bifurcation (for ks » 3) and a second corner collision (for ks » 2.999). After these bifurcations, chaotic bands appear and the process continues until the system enters in 1-band chaos (for ks » 0.5). Details of this bifurcation process can be found in [13, 18].
As far as we know, the system losses stability since it is unable to hold zero average, and many saturated duty cycles appear. This is basically because the control pulse u(t) is symmetric, and 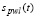 is not. We assume that the first and the third slopes of the piece-wise linear function
is not. We assume that the first and the third slopes of the piece-wise linear function 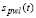 have the same value (
have the same value (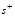 ) and they should be assumed to be different.
) and they should be assumed to be different.
Now, we propose a new general version of discrete ZAD (called discrete GZAD) given by
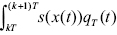 (26)
(26)
where  corresponds to a density of a discrete distribution, and thus it involves Delta functions. Then, as the previous continuous case, we force the expectation of
corresponds to a density of a discrete distribution, and thus it involves Delta functions. Then, as the previous continuous case, we force the expectation of 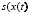 to be zero in each sampling period
to be zero in each sampling period 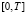 . Thus the integral in (26) can be computed as
. Thus the integral in (26) can be computed as
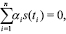 (27)
(27)
with 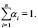 Depending on the number n of points, we have different expressions for the normalized duty cycle d. Also, as in classical discrete ZAD, we will assume that the function
Depending on the number n of points, we have different expressions for the normalized duty cycle d. Also, as in classical discrete ZAD, we will assume that the function  is close to a piece-wise linear function
is close to a piece-wise linear function 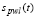 with 3 linear pieces in the sampling interval
with 3 linear pieces in the sampling interval 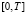 (1 piece if we have saturation in the duty cycle). We will show with detail the case for n = 2, being a good compromise between simplicity in control and good bounds for the voltage error, and also, because it completely exemplifies our method.
(1 piece if we have saturation in the duty cycle). We will show with detail the case for n = 2, being a good compromise between simplicity in control and good bounds for the voltage error, and also, because it completely exemplifies our method.
We have 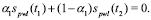 After some algebra, it is easy to obtain the following equivalent expression
After some algebra, it is easy to obtain the following equivalent expression
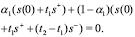 (28)
(28)
We still have many choices for 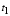 and
and  , and for α1; but there are several natural ways to choose them, according to the symmetry of the control variable u. One of the possible natural choices is t1 = d/2 and t2 = 1-d/2. Then the normalized duty cycle is found as
, and for α1; but there are several natural ways to choose them, according to the symmetry of the control variable u. One of the possible natural choices is t1 = d/2 and t2 = 1-d/2. Then the normalized duty cycle is found as
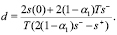 (29)
(29)
Of course, we also saturate d to 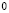 or
or 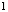 to obtain a value in the admissible interval
to obtain a value in the admissible interval 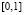 . In particular, taking into account our knowledge of the ZAD strategy, we apply this new version for improving the performance of the controller and for widening the stability range. It is worth noting that for α1 = 1/2, we reproduce the classical discrete ZAD strategy. However the duty cycle expression for the generalized ZAD (GZAD) is more powerful than the one for the classical ZAD strategy. This is because the system losses the stability mainly at the end of the sampling period. The system tries to preserve simultaneously zero average and the symmetry of the pulse, and both things together are hard to satisfy.
. In particular, taking into account our knowledge of the ZAD strategy, we apply this new version for improving the performance of the controller and for widening the stability range. It is worth noting that for α1 = 1/2, we reproduce the classical discrete ZAD strategy. However the duty cycle expression for the generalized ZAD (GZAD) is more powerful than the one for the classical ZAD strategy. This is because the system losses the stability mainly at the end of the sampling period. The system tries to preserve simultaneously zero average and the symmetry of the pulse, and both things together are hard to satisfy.
With the new expression for the duty cycle in (29) we can give more weight to the last part of the sampling interval and force the system to evolve better. In particular, we want to emphasize that even very small differences between duty cycles, produce completely different behaviors [14,19,20]. Figure 3 show the bifurcation diagram of the duty cycle using α1 as bifurcation parameter and fixing ks = 4.5. Taking into account that α1 is the weight of the value of 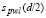 , at the sampling time, the minimum regulation error was obtained when α1 = 0.4967, which agrees with
, at the sampling time, the minimum regulation error was obtained when α1 = 0.4967, which agrees with 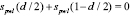 . This balances the difference between the real and expected values of
. This balances the difference between the real and expected values of 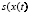 at
at 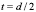 and at
and at 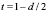 and now the system has almost the same behavior as the classical ZAD strategy without approximation (but with less computation effort) (see Fig. 1).
and now the system has almost the same behavior as the classical ZAD strategy without approximation (but with less computation effort) (see Fig. 1).
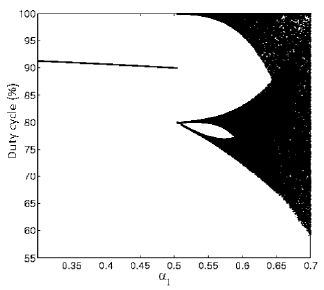
Figure 3. Bifurcation diagram of the duty cycle using α1 as bifurcation parameter. ks = 4.5
Figure 4 shows the bifurcation diagram for the maximum of the absolute value of the voltage error in each interval 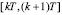 when α1 is varied (this is
when α1 is varied (this is 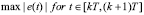 versus α1).
versus α1).
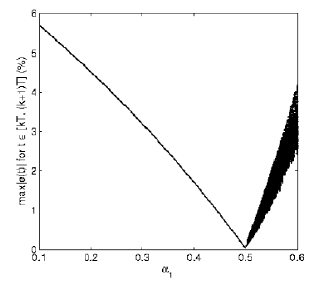
Figure 4. Bifurcation diagram of the maximum absolute voltage error in each period, using α1 as bifurcation parameter and fixing ks = 4.5Then, from the previous results we conclude that:
- The value of α1 for which the minimum voltage error in steady state is obtained is α1 = 0.4989.
- Higher values of α1 imply higher values of ks. However, previous analysis have shown that ks cannot be very high since although the system is still stable, it losses the regulation capability [12].
- From Fig. 4 we can choose α1 so that the stability is improved and the maximum voltage error is lower with a certain threshold (say, for example, with regulation error lower than 1
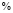 ).
).
Thus, this new technique can be used to design a PWM control technique in such a way that the compromise among accuracy, steady state voltage error, and the stability of the 1-periodic orbit can be properly handled. Taking this idea into account, we present the following section.
5. DESIGN OF AN IMPROVED CONTROLLER
Taking into account that now the main parameters are ks and α1, we need to state an optimal link between them. Since analytical results are very difficult to obtain, we proceed to relate ks and α1 through a two-dimensional numerical plot. Figure 5 shows the absolute value of the voltage error at the sampling time using α1 and ks as variables.
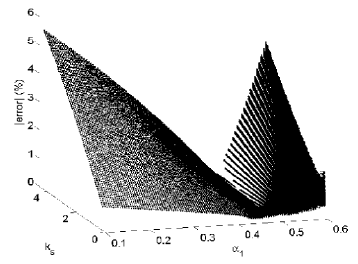
Figure 5. Plot of the absolute voltage error at the sampling time using α1 and ks as variables (the right part of the surface is unstable, while the left part is stable).
It is worth noting that even though the bifurcation diagrams shown in Figs. 4 and 5 can induce one to think that the best strategy is to select values close to classical discrete ZAD, these values give too much weight to the derivative part of 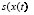 (
(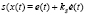 ) and the system hardly follows the ZAD strategy (that is, it has many saturated cycles which do not fit the ZAD strategy). Using the diagram shown in Fig. 5, we can observe that as we decrease the value of α1 it is possible to reduce the value of ks for obtaining a regulated 1-periodic orbit. Analyzing the same figure, we conclude that the weight (1- α1) applied to the second point of the discrete ZAD strategy has a significant effect on the stability. Even more, for α1 > 0.52, the system is unstable for ks < 5, and the technique cannot be applied. In fact, ks < 5 is required for regulation purposes. Specifically for our case, previous works have reported that the classical ZAD strategy is not robust enough to load changes. In [12] it was proven that for ks = 4.5 and varying γ, the system lost the stability of the one-periodic orbit close to γ = 0.26.
) and the system hardly follows the ZAD strategy (that is, it has many saturated cycles which do not fit the ZAD strategy). Using the diagram shown in Fig. 5, we can observe that as we decrease the value of α1 it is possible to reduce the value of ks for obtaining a regulated 1-periodic orbit. Analyzing the same figure, we conclude that the weight (1- α1) applied to the second point of the discrete ZAD strategy has a significant effect on the stability. Even more, for α1 > 0.52, the system is unstable for ks < 5, and the technique cannot be applied. In fact, ks < 5 is required for regulation purposes. Specifically for our case, previous works have reported that the classical ZAD strategy is not robust enough to load changes. In [12] it was proven that for ks = 4.5 and varying γ, the system lost the stability of the one-periodic orbit close to γ = 0.26.
On the other hand, using the proposed GZAD technique in this work and, taking ks = 0.3 and α1 = 0.3, the system preserves the stability of the 1-periodic orbit even if γ is very low 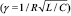 . The comparison between both strategies is presented in Figs. 6-7. The figures are devoted to showing the behavior of the regulation error. Although both have very similar values for the regulation error, the ZAD strategy has a lower voltage error than the GZAD strategy for γ > 0.1. However, very low voltage error is difficult to obtain in practical implementations due to problems related to the sensitivity of the sensors, noise, delays, etc. So, from a practical point of view, the global performance of the GZAD strategy is better than the classical ZAD strategy due to the fact that GZAD is more robust and shows no bifurcations (see Figs. 6 and 7).
. The comparison between both strategies is presented in Figs. 6-7. The figures are devoted to showing the behavior of the regulation error. Although both have very similar values for the regulation error, the ZAD strategy has a lower voltage error than the GZAD strategy for γ > 0.1. However, very low voltage error is difficult to obtain in practical implementations due to problems related to the sensitivity of the sensors, noise, delays, etc. So, from a practical point of view, the global performance of the GZAD strategy is better than the classical ZAD strategy due to the fact that GZAD is more robust and shows no bifurcations (see Figs. 6 and 7).
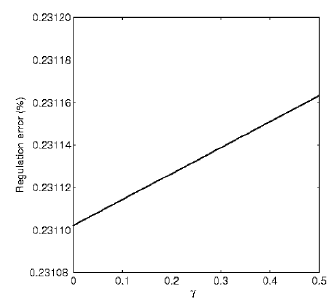
Figure 6. GZAD: bifurcation diagram of the voltage error, using γ as bifurcation parameter (ks = 0.3, α1 =0.3)
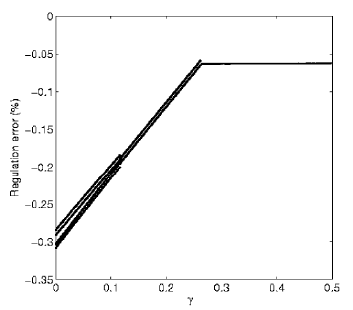
Figure 7. ZAD: Bifurcation diagram of the voltage error, using γ as bifurcation parameter (ks = 4.5)
Therefore we conclude that, for practical purposes, this new GZAD strategy with the improved method can have more applications than the classical ZAD strategy previously reported in the literature.
6. CONCLUSIONS AND FUTURE WORK
We can summarize our main results about the GZAD strategy as follows:
The main advantage of the GZAD strategy is related to the stability of the 1-periodic orbit. Taking into account the idea behind the ZAD strategy (fixed frequency switching), this new and generalized technique solves a lot of problems related to chaos, period doubling and, of course, the loss of fixed frequency switching.
GZAD strategy can be used in a wide range of situations and purposes. One of them is fixing ks and finding the best value of α1 assuming steady state conditions. Conversely, another purpose is fixing α1 and finding the best value for ks. However, results have shown that probably the best use is to keep ks and α1 in low values, as we did in Section 5 (ks = 0.3 and α1 = 0.3), using Fig. 5. The ks value must be low because, as the parameter ks increases, the regulation ability of the system is lost and the voltage error increases (see Fig. 5).
Despite the fact that the probabilities framework may look a bit unusual when dealing with DC-DC converters, we propose using the expression of the duty cycle given by (29), replacing α1 with 0.3 with ks = 0.3, instead of the duty cycle given by (25) which has two main disadvantages: on the one hand, it is more unstable than our proposed technique and, on the other hand, it exhibits a lot of nonlinear behavior, which makes that allows for the fixed frequency switching to be easily lost.
With our proposed procedure we are able to reproduce continuous and discrete classical ZAD strategies.
Our future work will be devoted to testing this technique on a prototype developed in our laboratory. Finally, we want to emphasize that the system with classical ZAD is highly sensitive to the duty cycle computation, as it has been previously observed and reported [14, 19]. This is why the experimental results for the classical ZAD strategy alone do not totally fit with the obtained numerical results. However, using the ideas of this GZAD strategy and, adding other control techniques such as the FPIC, we have obtained very good performance [28] and very good agreement between experimental and numerical results [29].
REFERENCES
[1] MOHAN N., UNDELAND T.M. AND ROBBINS W.P. Power electronics: converters, applications and design. New York, Wiley, second edition, 1995. [ Links ]
[2] SEVERNS R.P. AND BLOOM G. Modern DC to DC Switch-mode Power Converter Circuits. Van Nostrand - Reinhold, New York. 1985. [ Links ]
[3] SIRA-RAMREZ H. Sliding Motions in Bilinear Switched Networks IEEE Transactions on Circuits and Systems I, Vol. 34, No. 8, pp. 919-933, August 1987. [ Links ]
[4] BANERJEE S. AND VERGHESE G.C. Nonlinear phenomena in power electronics, IEEE Press, Piscataway. 2001. [ Links ]
[5] MAITY S., TRIPATHY D., BHATTACHARYA T. K. AND BANERJEE S. Bifurcation Analysis of PWM-1 Voltage-Mode-Controlled Buck Converter Using the Exact Discrete Model IEEE Transactions on Circuits and Systems I: Regular Papers, Vol. 54, No. 5, pp. 1120-1130, May 2007. [ Links ]
[6] YUE MA, KAWAKAMI H. AND TSE C.K. Bifurcation analysis of switched dynamical systems with periodically moving borders IEEE Transactions on Circuits and Systems I: Regular Papers, Vol. 51, No. 6, pp. 1184-1193, June 2004. [ Links ]
[7] TSE C.K., Complex Behavior of Switching Power Converters, Boca Raton, CRC Press, 2004. [ Links ]
[8] DI BERNARDO M., CHAMPNEYS A.R., HOGAN S.J., HOMER M.E., KOWALCZYK P., KUZNETSOV YU.A. AND NORDMARK A.B. Two-parameter non-smooth bifurcations of limit cycles: Challenges and open problems, International Journal of Bifurcation and Chaos, Vol. 16, No. 3, pp. 601 - 629, 2006. [ Links ]
[9] EL AROUDI A. AND ROBERT B., Modeling and Dynamics of a High Voltage DC-DC Converter: A Nonlinear Approach, NOLTA 04, pp. 311 - 316, Fukuoka, Japan, 2004. [ Links ]
[10] EL AROUDI A., BENADERO L., TORIBIO E. AND MACHICHE S. Quasiperiodicity and Chaos in the DC-DC Buck-Boost Converter, International Journal of Bifurcation and Chaos, Vol. 10, No. 2, pp. 359 - 371, October 2000. [ Links ]
[11] FOSSAS E., GRIÑÓ R. AND BIEL D. Quasi-sliding control based on pulse width modulation, zero average and the norm, in Advances in Variable Structure System, Analysis, Integration and Applications. Edited by Xinghuo Yu and Jian-Xin Xu. Ed. World Scientific, pp. 335-344, 2001. [ Links ]
[12] ANGULO F. Análisis de la dinámica de convertidores electrónicos de potencia usando PWM basado en promediado cero de la dinámica del error (ZAD). [Ph.D. Thesis], Universitat Politécnica de Catalunya, July 2004 (in Spanish). Available at http://www.tdx.cesca.es/TDX-0727104-095928/. [ Links ]
[13] ANGULO F., FOSSAS E. AND OLIVAR G. Transition From Periodicity to Chaos in a PWM-Controlled Buck Converter with ZAD strategy. International Journal of Bifurcations and Chaos, Vol. 15, No. 10, pp. 3245–3264, 2005.
[14] ANGULO F., OLIVAR G. AND DI BERNARDO M. Two-Parameter Discontinuity-Induced Bifurcation Curves in a ZAD-Strategy-Controlled DC–DC Buck Converter. IEEE Transactions on Circuits and Systems I, Vol. 55, No. 8, pp. 2392-2401, 2008.
[15] RAMOS R., BIEL D., FOSSAS E. AND GUINJOAN F. A fixed-frequency quasi-sliding control algorithm: Application to power inverters design by means of FPGA implementation IEEE Transactions on Power Electronics, Vol. 18, No. 1, January 2003. [ Links ]
[16] BIEL D. AND FOSSAS E. SMC applications in Power Electronics, in Variable Structure Systems: from principles to implementation, L. Fridman, A. Sabanovi and S. Spurgeon Ed., IEE, 2004. [ Links ]
[17] ANGULO F., OCAMPO C., OLIVAR G AND RAMOS R. Nonlinear and nonsmooth dynamics in a DC-DC Buck converter: two experimental set-ups. Nonlinear Dynamics, Vol. 46, pp. 239-257, 2006. [ Links ]
[18] ANGULO F., OLIVAR G. AND TABORDA A. Continuation of periodic orbits in a ZAD-strategy controlled buck converter. Chaos, Solitons and Fractals, Vol. 38, pp. 348-363, 2008. [ Links ]
[19] FOSSAS E., HOGAN S.J. AND M-SEARA, T. Two-parameter bifurcation curves in power electronic converters. International Journal of Bifurcation and Chaos, Vol. 19, No. 1, pp. 349-357, 2009. [ Links ]
[20] GRANADOS A. Virtual orbits and two-parameter bifurcation analysis in power electronic converters M.Sc. Thesis, Universitat Politécnica de Catalunya, 2009. [ Links ]
[21] TABORDA A., ANGULO F. AND OLIVAR G. Bifurcation Analysis on Nonsmooth Torus Destruction Scenario of Delayed-PWM Switched Buck Converter International Journal of Bifurcations and Chaos, Vol. 19, No. 7, pp. 2193–2212, July 2009.
[22] TAN S-C., LAI Y.M., TSE C.K., MARTINEZ-SALAMERO L. AND WU C.K., A Fast-Response Sliding-Mode Controller for Boost-Type Converters with a Wide Range of Operating Conditions, IEEE Transactions on Industrial Electronics, Vol. 54, No. 6, pp. 3276 - 3286, December 2007. [ Links ]
[23] ANGULO F., BURGOS E. AND OLIVAR G. Study of nonlinear dynamics in a buck converter controlled by lateral PWM and ZAD, in Modeling, simulation and control of nonlinear engineering dynamical systems, state-of-the-art, perspectives and applications, pp. 313-324. Jan Awrejcewicz Ed., Springer, 2009. [ Links ]
[24] TABORDA J.A., ANGULO F., OLIVAR G. AND HOYOS F. Non-smooth dynamics and FPIC chaos control in a DC/DC ZAD strategy power converter Proceedings of EUROMECH Nonlinear Dynamics Conference ENOC, Saint Petersburg, Russia, 2008. [ Links ]
[25] SIRA-RAMÍREZ H. A geometric approach to pulse-width modulated control in nonlinear dynamical systems IEEE Transactions on Automatic Control, Vol. 34, No. 2, pp. 184-187, February 1989. [ Links ]
[26] ANGULO F., FOSSAS E. M-SEARA T. AND OLIVAR G. Bounding the Output Error in a Buck Power Converter Using Perturbation Theory Hindawi Publishing Corporation, Mathematical Problems in Engineering,Vol.2008, Article ID 732039. doi:10.1155/2008/732039. [ Links ]
[27] CARPITA M., MARCHESONI M., OBERTI M., AND PUGUISI L. Power Conditioning System Using Slide Mode Control Proceedings of the IEEE Power Electronic Specialists Conference, Kyoto, Japan, pp. 623-633, 1988. [ Links ]
[28] EL AROUDI A., ANGULO F., OLIVAR G., ROBERT B.G.M., AND FEIKI M. Stabilizing a Two-Cell DC-DC Buck Converter by Fixed Point Induced Control International Journal of Bifurcations and Chaos, Vol. 19, No. 6, pp. 2043–2057, June 2009.
[29] HOYOS F.E., ANGULO F., TABORDA J.A AND OLIVAR G. Implementation of a new digital control technique for dc-dc and dc-ac power converters. To be published, DYNA (in Spanish). 2009. [ Links ]














