Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Cited by Google
Cited by Google -
 Similars in
SciELO
Similars in
SciELO -
 Similars in Google
Similars in Google
Share
DYNA
Print version ISSN 0012-7353
Dyna rev.fac.nac.minas vol.82 no.192 Medellín July/Aug. 2015
https://doi.org/10.15446/dyna.v82n192.47752
DOI: http://dx.doi.org/10.15446/dyna.v82n192.47752
Settlement analysis of friction piles in consolidating soft soils
Análisis de asentamientos de pilas de fricción en suelos blandos compresibles
Juan F. Rodríguez-Rebolledo a, Gabriel Y. Auvinet-Guichard b & Hernán E. Martínez-Carvajal a,c
a Department of Civil & Environmental Engineering, University of Brasilia, Brazil. jrodriguezr72@hotmail.com
b Instituto de Ingeniería, Universidad Nacional Autónoma de México, Mexico. gauvinetg@iingen.unam.mx.
c Facultad de Minas, Universidad Nacional de Colombia, Sede Medellín, Medellín, Colombia. hmartinezc30@gmail.com
Received: December 8th, 2014. Received in revised form: April 10th, 2015. Accepted: Abril 30th, 2015.
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.

Abstract
The paper shows how axisymmetric finite element numerical models can be used to optimize the design of friction piles foundations in an environment that is prone to regional subsidence. The study considers friction piles in typical Mexico City soft clays, that are subjected to external loads and soil consolidation due to variations in piezometric conditions. The constitutive models used to numerically simulate the behavior of the clays vary from a basic elastic perfectly-plastic model to a critical state model that is able to account for the anisotropic yielding behavior of Mexico City clay. The simulations consider the long term behavior of the internal piles within a large pile group.
Keywords: friction piles; pile group; regional subsidence; numerical modeling; anisotropy; constitutive models, Mexico City clay.
Resumen
Este artículo muestra como el uso de modelos axisimétricos implementados en códigos de elementos finitos pueden ser usados para optimizar el diseño de cimentaciones con pilotes de fricción en ambientes susceptibles a hundimiento regional. El estudio considera pilotes de fricción instalados en el suelo blando de la Ciudad de México, sometidos a cargas externas y a la consolidación del suelo debida a variaciones en las condiciones piezométricas. Los modelos constitutivos empleados para simular el comportamiento del suelo compresible varían desde un elástico-plástico perfecto hasta uno basado en la teoría del estado crítico que toma en cuenta la plastificación anisotrópica de las arcillas de la Ciudad de México. Las simulaciones consideran el comportamiento a largo plazo de un grupo de pilotes supuesto infinito.
Palabras clave: pilotes de fricción, grupos de pilotes, hundimiento regional, modelado numérico, anisotropía, modelos constitutivos, arcilla de la Ciudad de México.
1. Introduction
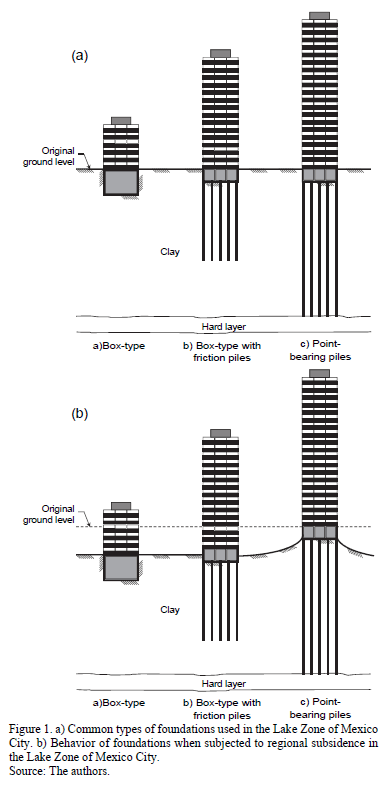
Commonly, three main types of foundations (Fig. 1a) are used in the lacustrine zone of Mexico City [1]: box-type shallow foundation for small buildings, box-type foundation with friction piles for intermediate height buildings [2,3] and point-bearing piles for very tall or heavy structures. Friction piles transfer most of their load to the soil through skin friction. In the soft soils of Mexico City, friction piles are mainly used as a complement to box-type foundations to reduce settlements. Infrequently, they have been used to ensure the overall foundation stability (bearing capacity design). In all cases, a complex interaction between soil, piles and structure can be expected, because the foundations are submitted to the effects of a double process of consolidation: firstly due to the load of the structure and, secondly, due to pore pressure drawdown associated to intense pumping of water from the subsoil in the urban area.
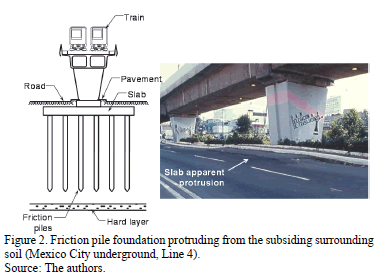
Since the end of the 19th century, Mexico City Lake Zone has suffered a regional subsidence, which, in some areas, has exceeded 10m. In such conditions, point-bearing piles foundations can lead to apparent protrusion of the structure, as shown in Fig. 1b, with loss of confinement of the upper part of the piles and damage to neighboring structures. On the other hand, when not properly designed, friction pile foundations can either settle excessively or, on the contrary, protrude from the subsiding surrounding soil (Fig. 2).
Some field tests were conducted on piles in a consolidating soil subjected to pore pressure drawdown [4-7], but only a few of them involve friction piles. Analytical methods for the design of friction pile foundation in these difficult conditions have been proposed [2,3,8]. The finite element method (FEM) has been increasingly used for the analysis and design of pile foundations subjected to negative skin friction [9-14]. Numerical modeling allows soil behavior complexities and soil-structure interaction, to be taken into account as well as changes in pore pressure regime.
The aim of this paper is to demonstrate how 2D finite element analyses can be used to optimize the design of friction piles in an environment that is prone to regional subsidence. Firstly, some background information regarding typical Mexico City soil conditions and regional subsidence is provided. Next, parametric analyses on the behavior of friction piles subjected to external loads and soil consolidation due to variations in piezometric conditions are presented. The constitutive models used, vary from a basic elastic perfectly-plastic Mohr Coulomb model to S-CLAY1, a critical state model with plastic anisotropy [15,29,30]. The principles of numerical modeling and the constitutive models used are briefly described, followed by numerical analyses and discussion of the results.
2. Background
2.1. Typical soil conditions
The urban area of Mexico City can be divided in three geotechnical zones [17]: Foothills (Zone I), Transition (Zone II) and Lake (Zone III), as defined in the present building code [1]. In the Foothills Zone, very compact and heterogeneous volcanic soils and lava fluxes are found. These materials contrast with the highly compressible soft soils of the Lake Zone. Generally, in between, a Transition Zone is found where clayey layers of lacustrine origin alternate with erratically distributed sandy alluvial deposits. The main difficulties for foundations of high buildings are encountered in the Lake and Transition zones.
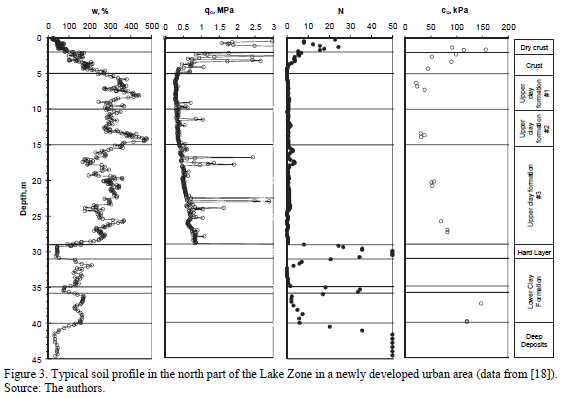
Until the end of the 18th century, the valley of Mexico was a closed basin with a number of shallow lakes, including the Texcoco and Xaltocan lakes. The valley became an open basin when the Nochistongo cut, a channel 7 km long and up to 50 m deep (dug by hand between 1637 and 1789) was completed. Progressively, the lakes were drained, mainly through the Tequisquiac and Deep Drainage (Emisor Central) tunnels, and practically disappeared. A large part of the city was built on lacustrine sediments, which are highly plastic soft clays interbedded with layers of silt, sand and sandy gravels of alluvial origin. In Fig. 3, a typical Lake Zone soil profile is presented, which was obtained from the SIG [18]. Three main clayey layers are referred to as Upper Clay Formation (UCF), Lower Clay Formation (LCF) and Deep Deposits (DD). The clays of the Upper Clay Formation are separated from the Lower Clay Formation by the Hard Layer (HL), a sandy silt or clay stratum, between 2 and 3 m thick, lying typically at a depth of from 29 to 35 m. Generally, a dry crust of desiccated soils and/or anthropic fill, several meters thick, is found above the Upper Clay Formation layer, which has been influenced by drying and wetting cycles due to historic fluctuations of the water table.As seen in Fig. 3, Mexico City clay has a very high water content (corresponding to a void ratio as high as 10), a low cone resistance in the CPT and practically a nil blow count in an SPT test. Undrained shear strength increases with depth, with values of around 20 kPa in the upper part of the UCF and 80 kPa in the contact with HL. This slightly overconsolidated material (overconsolitation ratio varies from 1 to 1.3 [18]) is highly compressible.
2.2. Regional subsidence
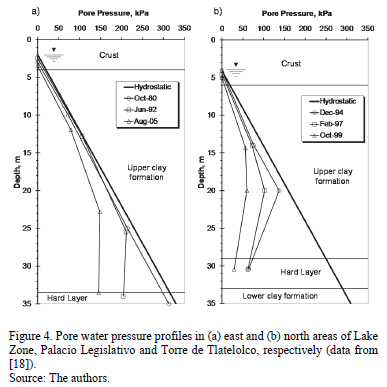
Due mainly to exploitation of ground-water to supply the growing population through pumping wells, Mexico City has suffered regional subsidence that in some locations exceeds 10 m. Recent data shows that the rate of subsidence tends to decrease in certain areas. However, in newly developed urban zones, such as the eastern and western parts of Texcoco Lake and the former Xochimilco and Chalco lakes, the consolidation process is only in its first stage and the rate of subsidence can be as high as 0.4m/year. Pore pressure drawdown due to the pumping of water in deep pervious strata (Hard Layer and Deep Deposits) leads to the typical piezometric profiles that are shown on Fig. 4. The resulting settlements of the Upper Clay Formation soil considerably affect the behavior of pile foundations.
3. Numerical modeling
3.1. General considerations
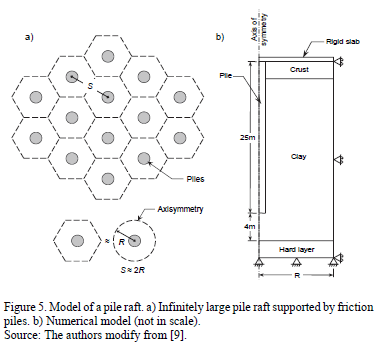
A group of friction piles, connected to an infinitely large rigid slab is considered (Fig. 5a). The simulations deal with the long term behavior of the internal piles. The tributary area [19] of each internal pile is hexagonal, but it can be idealized as a circular unit cell (Fig. 5a). The radius of the tributary area is then equal to half of the center-to-center spacing between piles. The problem can then be modeled as axisymmetric (Fig. 5b). Given that the main interaction between piles and soil takes place within the Upper Clay Formation, the less compressible layers below the Hard Layer have not been included in the analyses. The problem was discretized using a finite element mesh with more than 2,500 fifteen-node triangular elements (total density about 55 elements/m2). A mesh densification (from 350 to 750 elements/m2) along the pile shaft and below the rigid slab and the pile cap had to be considered [31]. Lateral boundaries were fixed in the horizontal direction, and the bottom boundary in both directions (Fig. 5b). Sensitivity studies showed that the mesh was dense enough to give accurate results and that it was not necessary to use interface elements at the soil-pile contact.
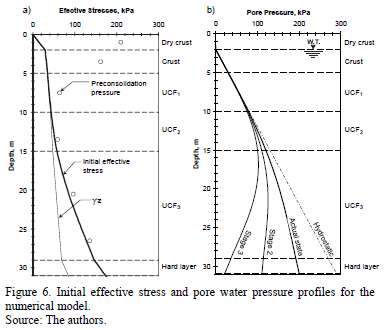
Parametric studies were performed for a foundation slab on friction piles, which were assumed to be 25 m long (finishing 4 m above of the Hard Layer) and 0.5 m in diameter. The weight of a typical five to ten floor building was considered by applying a load of 75 kPa directly to the rigid slab. The analyses were developed in three stages. In Stage 1, pile was placed (no installation effects were considered, only the pile weight as discussed below) and the 75 kPa load was applied on the slab; in Stage 2 the first pore pressure drawdown was introduced (Fig. 6), simulating typical future piezometric conditions (Fig. 4.a); and in Stage 3 a second pore pressure drawdown was considered (Fig. 6), representing an extreme, but possible, future piezometric condition in Mexico City (Fig. 4.b). The conditions at the end of each stage of the analysis, after the excess pore pressure due to the applied load and pore pressure drawdown having been completely dissipated, were assessed.
Pile driving in very soft soils results in soil disturbance: changes in soil structure and excess pore pressures around the piles. These effects are difficult to model with finite element analyses, due to the excessive mesh distortions, and are hence ignored. However, given that the combined pile weight and the frictional force at soil-pile interface far exceed the buoyancy force, the system is not in equilibrium after pile driving and thus additional deformations occur simply due to pile weight. These deformations are not negligible in Mexico City Clay, as demonstrated by Auvinet and Hanel [7] by means of field observations. Therefore, the effect of pile weight has been taken into account in the numerical analyses as part of Stage 1. In general, the displacements induced by installation are almost negligible in comparison with long-term displacements in Mexico City due to the regional subsidence.
3.2. Constitutive models
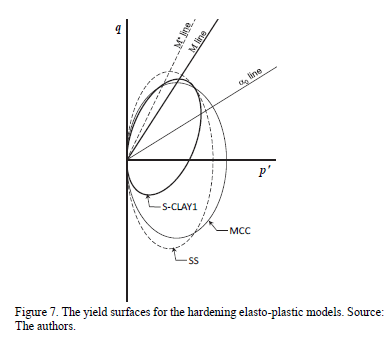
For simulation of the clay behavior (Upper Clay Formation), elastic perfectly-plastic and hardening elasto-plastic constitutive models were used. The elastic perfectly-plastic model is the Mohr-Coulomb (MC) model commonly used in industry. The hardening elasto-plastic models include two isotropic models: Modified Cam-Clay [20] and the Soft Soil (SS) model. The Soft Soil model, available in the commercial version of PLAXIS finite element code, has been inspired by the MCC model, but the yield surface and failure surface have been decoupled in order to have a proper K0 prediction at normally consolidated states. Hence, the ellipsoidal yield surface is much steeper than the ellipse of the MCC model (see Fig. 7) and the shape is defined by the input of estimated K0NC (used for calculating the value for shape parameter M*). According to Ovando-Shelley et al. [21], K0NC for Mexico City clay has been measured to correspond to Jaky's K0. The failure condition in the SS model can be described by a Mohr Coulomb failure line, and consequently on the 'dry' side of failure line, the model reverts to a non-linear elastic-perfectly plastic model with zero dilatancy. On the 'wet' side of the failure line, the SS model predicts volumetric hardening similarly to the MCC model, but this is expressed in terms of modified compression and swelling indices, and hence at large strains the results will deviate from those of the MCC model.
The S-CLAY1 [15] model is a critical state model, which accounts for initial and plastic strain induced anisotropy through an inclined initial yield surface and a rotational hardening law that describes the evolution of anisotropy as a function of plastic strains. Based on data by Diaz-Rodríguez et al. [16], S-CLAY1 is able to accurately represent the extremely high anisotropy at yielding that Mexico City clay exhibits [15].
3.3. Soil properties
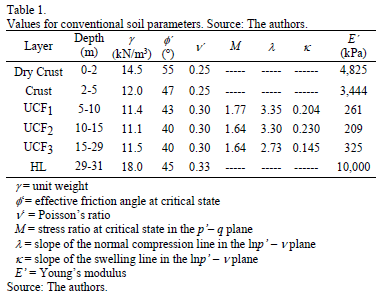
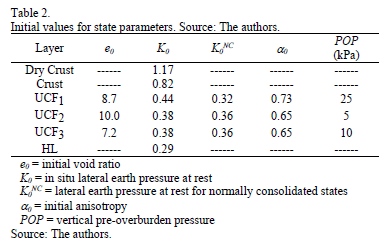
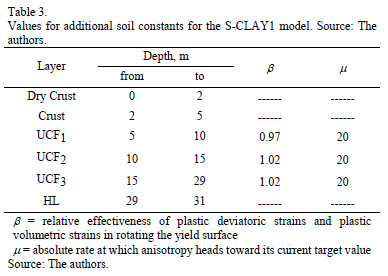
The soil conditions correspond to a relatively new residential area, near the former Texcoco Lake. This is practically virgin terrain with no previous loading history. A notable amount of ground investigation data were available from [18] database, including cone penetration tests, standard penetration tests mixed with Shelby sampling, piezometric measurements and laboratory triaxial and consolidation tests. Fig. 3 shows the considered soil profile, and Tables 1 to 3 present the values of soil constants and state parameters of the materials considered in the analyses based on ground investigation data and laboratory testing. Due to natural variability, there was some scatter in the values, and for each layer representative mean values have been chosen.
The values for f' and M were deduced from CD and CU triaxial tests published by Marsal and Mazari, Marsal and Salazar, Lo, Alberro and Hiriart and Villa [17,22-25], and the values for e0, l, k, E' and the vertical pre-overburden pressure POP have been obtained from one-dimensional consolidation tests and calibrated with triaxial consolidation results published by Villa and Diaz-Rodríguez et al. [25,16]. The values for K0NC for the input of the Soft Soil model have been estimated from Jaky's formula [26]. The in situ values of K0 were derived from the equation presented by Mayne and Kulhawy [27]. As explained by Wheeler et al. [15], the values for the initial inclination of the yield surface (a0) and the soil constant b can be theoretically derived based on the value of friction angle at critical state (f'). The value for m has been taken as the smallest value reported by [15], given experimental results that would enable the optimization of the value are not available. As shown by Wheeler et al. [15], S-CLAY1 model predictions are not particularly sensitive to this value. The soil constant m controls the absolute rate at which the inclination of the yield surface a heads toward its current target value, and b controls the relative effectiveness of plastic deviatoric strains and plastic volumetric strains in rotating the yield surface.
Fig. 6 shows the initial effective vertical stress and pore-water pressure profiles that are assumed in the numerical analyses. The actual state of the pore water pressure (Fig. 6b) has been obtained from piezometers installed in thin sandy layers within Upper Clay Formation and Hard Layer. The water table is assumed to, be at a depth of 2 m, and to stay constant. A significant pressure reduction, of about 90 kPa with respect to the hydrostatic distribution, was observed in the Hard Layer due to groundwater extraction. With these values and with the measured soil density of each layer, the initial effective stresses were evaluated (Fig. 6a). The preconsolidation pressure was estimated from 1-D consolidation tests (experimental results are shown with open circles). Due to historical wet-dry cycles, the dry crust, which geologically is part of Upper Clay formation, shows evidence of significant overconsolidation and its behavior may be simulated by using Mohr Coulomb model. The Upper Clay Formation exhibits evident overconsolidation because of water table variations with depth during dry and rainy seasons. Fig. 6b also shows the pore pressure distributions considered for Stages 2 and 3 of the analyses. The future pore pressure drawdown considered in each stage was assumed to be equal to the difference between the actual and the assumed profiles. This assumption results in a consolidation of UCF of about 5 cm/year, for a 20 and 40 year period, for Stages 2 and 3 respectively due to regional subsidence. This is consistent with the consolidation rates measured based on superficial and deep settlement benchmarks installed in the Lake Zone of Mexico City.
3.4. Results of numerical analysis
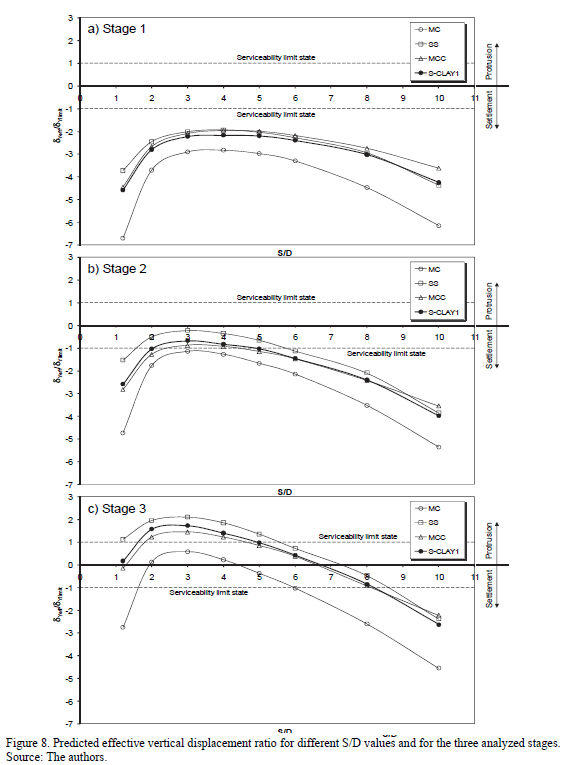
Fig. 8 presents the predicted effective vertical displacement ratio, dYeff/dYlimit for different normalized pile spacing (S/D), for the three stages of the analysis. The variable dYeffrefers to the vertical effective displacement predicted for the piled raft, which is defined as:
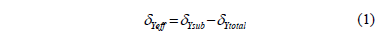
where dYsubis the superficial subsidence induced by pore pressure drawdown (in absence of piles) and dYtotal is the total vertical displacement predicted for the piled raft. The variable dYlimitrefers to the Mexico City Code serviceability limit state for isolated structures. Negative values of dYeff mean that the piled raft is settling and positive values correspond to apparent protruding.
For the first stage of the analysis (i.e. the load of the building and pile weight), dYsub=0; therefore, the effective displacement is the same as the total one, dYeff = dYtotal. It was observed that all models exhibit the same trend and the predicted settlements ratios exceed the serviceability limit state. For S/D < 3, the settlement increases due to the effect of the pile weight, since the unit weight of the soil (mean value of 11.5 kN/m3 for Upper Clay Formation) is partially substituted by the unit weight of the pile (24 kN/m3). The smallest settlements are observed for relative spacing ratio S/D in the 3-5 range. The Mohr-Coulomb model predicts the largest settlement ratio (Fig. 8). This type of model can hardly be considered as realistic since a constant value for elastic stiffness parameters is assumed, independently of the stress level and the evolution of the stress path.
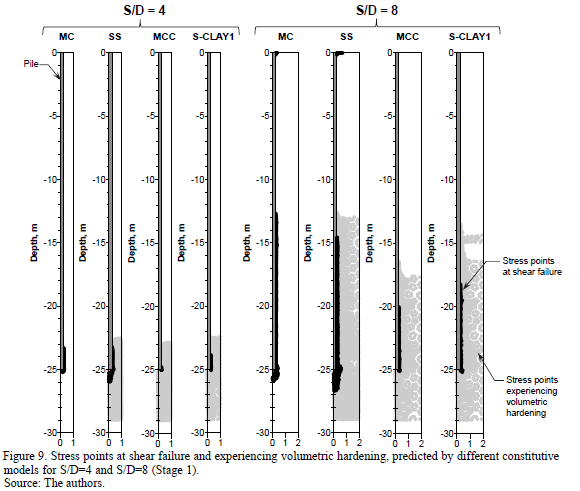
As shown in Fig. 9, the increase in the settlements predicted by the different constitutive models for S/D>5 is due to the increase in the number of stress points at shear failure and undergoing volumetric hardening. The Mohr-Coulomb and the Soft Soil models predict a localized zone of failure below the pile tip, not detected by the critical state models (MCC and S-CLAY1S), and the predicted extent of full mobilization of friction along the shaft varies depending on the different models.
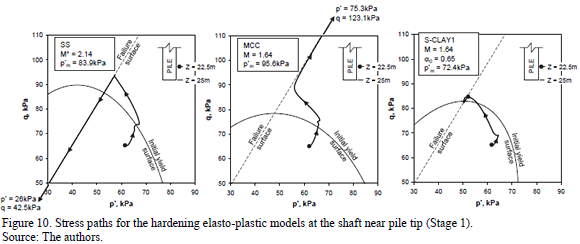
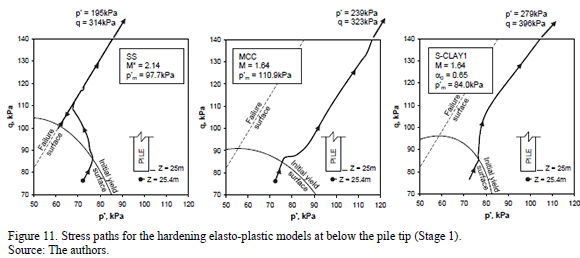
For center-to-center spacing smaller than 5D, the results obtained with all hardening elasto-plastic models are almost identical (Fig. 8), which demonstrates that the input values have been consistently calibrated. Beyond this point, some differences between the models can be seen. The Soft Soil (SS) model predicts a much larger plastic zone than the MCC and S-CLAY1 models (Fig. 9). This is due to the differences in the predicted stress paths during the loading process. To illustrate this, two stress points have been selected for inspection of relative pile spacing of S/D= 8: one next to the pile shaft near the tip (Fig. 10) and one just beneath the pile tip (Fig. 11). In addition to the stress paths, the yield and failure surfaces have been outlined.
Fig. 10 shows that the isotropic SS and MCC models predict totally different stress path directions when reaching failure/critical state. This is due to the assumption of Mohr-Coulomb failure adopted in the SS model. For the S-CLAY1 model prediction, most of the stress path remains within the elastic region, but ultimately the critical state is reached, followed by some softening due to the effect of anisotropy.
Fig. 11 shows that for the point situated at a depth of 0.8D beneath the pile tip, failure is predicted only by the SS model, as would be expected based on Fig. 9. The MCC model predicts an unrealistically high K0NC value (Fig. 11), whilst S-CLAY1 prediction is consistent with measurements of Mexico City clay [21]. Despite the notable differences in the predicted stress paths in the soil near the pile shaft and tip, the differences in the predicted general behavior are not particularly significant (Fig. 8). It is concluded that for long-term analyses the compressibility behavior of the reinforced soil mass is more relevant than the pile-soil interaction. This explains why in this analysis soil-pile interface elements are not required, although they could actually be required for bearing capacity simulations.
Fig. 8.b shows the predicted displacements ratio for Stage 2 of the analyses (i.e. when the first pore pressure drawdown was introduced). Again, all models show the same trend, and the values of dYeff / dYlimit are closer to zero and to the serviceability limit state than those obtained for the first stage. This is because the soil has been reinforced with piles, and consequently the settlements due to the pressure drawdown are smaller in the reinforced area than in the surrounding soil. The optimum pile center-to-center spacing, corresponding to predictions that are closest to the serviceability limit state, is within the 2D to 4D spacing range.
Further regional subsidence due to an additional decrease in pore water pressure (Stage 3 of the analyses), induces a substantial change in the predicted behavior of the pile foundation, as shown in Fig. 8.c. For example, the Soft Soil model suggests that for a pile spacing of 3D, the foundation protrudes from the surrounding soil and is more than twice as high as dYlimit. On the other hand, for S/D=10, dYeff = dYlimit equals to -2.3; the foundation settles and exceeds 2.3 times the dYlimit. An optimum solution would be reached with -1< dYeff / dYlimit<1. Overall the results by the hardening elasto-plastic models suggest that the optimum pile spacing corresponds to values of S/D between 5.5 and 8, while the Mohr-Coulomb model suggests a range of considerably lower values, between 3 and 6.
In addition, the results presented in Fig. 8 indicate that in order to reach an optimum foundation design in a subsiding environment it is important to make an accurate prediction of the future pore pressure drawdown at the site. Unfortunately, it is difficult to develop a reliable estimation of the future piezometric condition at the site due to the changing requirements of Mexico City's water supply. Therefore, design should be based on an intermediate solution, which minimizes the inconveniences of both settlement and apparent protrusion. In the example presented, according to the S-CLAY1 model, a relative spacing of S/D=5 appears to be adequate, since no protrusion or settlement greater than dYlimit was predicted for Stages 2 and 3. The choice of constitutive model has an equally important role for a realistic optimum foundation design. Given the non-linearity of soft soil response, the Mohr Coulomb model is unlikely to give accurate predictions. In areas prone to regional subsidence due to groundwater extraction accounting for stress-dependent stiffness is extremely important.
Some differences between the hardening elasto-plastic model predictions can be observed for Stages 2 and 3. The magnitude of the total displacements is becoming larger, and hence the minor differences between the models in terms of stress-strain calculations become more significant, such as the slightly different considerations for computing volumetric strains implicit in the Soft Soil model as compared to MCC and S-CLAY1 (namely the use of l* instead of l). The shape of the yield surface is another factor (Fig 7). The Soft Soil model predicts the smallest displacements (i.e. the largest protrusion) because it has the largest elastic domain in the region of interest, i.e. between K0 line and critical state/failure line (Fig. 7). The sizes of the yield surfaces coincide initially at a stress ratio corresponding to one-dimensional loading (defined by Jaky's K0) in order to predict yield with the same value of vertical effective stress. The MCC and the S-CLAY1 models show some differences in the predictions because anisotropy influences the calculation of horizontal stresses [30].
4. Conclusions
The behavior of friction pile foundations in typical Mexico City soft clays that are subjected to external loads and soil consolidation due to variations in piezometric conditions was studied using numerical analyses. Vertical displacements of the foundation for different relative spacing S/D (center-to-center spacing vs. pile diameter) were predicted using different constitutive models: an elastic-perfectly plastic Mohr-Coulomb model, and three hardening elasto-plastic models. The Modified Cam Clay and Soft Soil models are isotropic models and the S-CLAY1 model is an anisotropic model that is able to account for the high anisotropy in yielding that is typical for Mexico City clay.
For the three stages of analyses, all hardening elasto-plastic models exhibit the same trend. The Mohr-Coulomb model predicts however notably larger settlements than the other models, but these predictions are unlikely to be realistic as a constant Young's modulus has to be assumed.
Some differences between the predictions by the hardening elasto-plastic models were observed when pore pressure drawdown was included, triggering regional subsidence. This is because when the magnitude of the total displacements is large, the differences between the models become significant. Specific considerations in each model for computing volumetric strains, the shape of the yield surface and the effect of initial anisotropy can explain these differences.
The models show that due to soil consolidation, a neutral level separating positive skin friction from negative skin friction develops on the pile shaft. The position of this level depends more on pile spacing than on the magnitude of the pore pressure drawdown. For close pile spacing, the neutral level is near the pile tip and the piles can protrude from the consolidating surrounding soil as a result of regional subsidence.
According to the results obtained, to reach an optimum foundation design in a subsiding environment, it is important to make an accurate prediction of the future pore pressure drawdown at the site. Unfortunately, due to changing hydrogeological conditions, it is difficult to develop a reliable estimation. Therefore, an optimum foundation design should be based on an intermediate solution minimizing inconveniences of both settlement and protrusion, and using a constitutive model which is a most representative idealization of the soil behavior. However, for further studies, a coupling between hydrogeological and soil mechanics numerical models could be envisioned to improve foundation design. These could be achieved by advanced hydrogeological research in order to improve long-term pore-pressure drawdown predictions.
It is shown that axisymmetric finite element numerical models can be used to optimize the design of friction piles foundations in an environment that is prone to regional subsidence.
References
[1] Gobierno del Distrito Federal, Normas técnicas complementarias para diseño y construcción de cimentaciones, Gaceta Oficial del Distrito Federal, 6th October, VII, N 103-BIS, Mexico City, 2004, pp. 11-3. [ Links ]
[2] Zeevaert, L., Compensated friction-pile foundation to reduce the settlements of buildings on the highly compressible volcanic clay of Mexico City, Proc. 4th ICSMFE, London, 2, pp. 81-86, 1957. [ Links ]
[3] Zeevaert, L., Foundations problems related to ground surface subsidence in Mexico City, ASTM (STP), 322, pp. 57-66, 1963. [ Links ]
[4] Plomp, A. and Mierlo, W.C., Special problems, effects of drainage by well points on pile foundations, Proc. 2nd ICSMFE, Rotterdam, 4, pp. 141-148, 1948. [ Links ]
[5] Endo, M., Minou, A., Kawasaki, T. and Shibata, T., Negative skin friction acting on steel pipe pile in clay, Proc. 7th ICSMFE, Mexico City, 2, pp. 85-92, 1969. [ Links ]
[6] Fellenius, B.H. and Broms, B.B., Negative skin friction for long piles driven in clay, Proc. 7th ICSMFE, Mexico City, 2, pp. 93-98, 1969. [ Links ]
[7] Auvinet, G. and Hanel, J.J., Negative skin friction on piles in Mexico City clay, Proc. 10th ICSMFE, Stockholm, 2, pp. 599-604, 1981. [ Links ]
[8] Reséndiz, D. and Auvinet, G., Analysis of pile foundations in consolidating soils, Proc. 8th ICSMFE, Moscow, 2, pp. 211-218, 1973. [ Links ]
[9] Auvinet, G. and Rodríguez, J.F., Friction piles in consolidating soils, Proc. 15th ICSMGE, Istanbul, 2, pp. 843-846, 2001. [ Links ]
[10] Auvinet, G. and Rodríguez, J.F., Modeling of friction piles in consolidating soils, Proc. Int. Deep Found. Cong., ASCE, Orlando, USA, pp. 224-235, 2002. [ Links ]
[11] Comodromos, E.M. and Bareka, S.V., Evaluation of negative skin friction effects in pile foundations using 3D nonlinear analysis. Comp. and Geotech. J., 32, pp. 210-221, 2005. DOI: 10.1016/j.compgeo.2005.01.006 [ Links ]
[12] Jeong, S., Kim, S. and Briaud, J.L., Analysis of downdrag on pile groups by the finite element method. Comp. and Geotech. J., 21 (2), pp. 143-161, 1997. DOI: 10.1016/S0266-352X(97)00018-9 [ Links ]
[13] Jeong, S., Lee, J. and Lee, C.J., Slip effect at the pile-soil interface on dragload. Comp. and Geotech. J., 31, pp. 115-126, 2004. DOI: 10.1016/j.compgeo.2004.01.009. [ Links ]
[14] Lee, C.J. and Charles, W.W., Development of downdrag on piles and pile groups in consolidating soil. J. Geotech. and Geoenv. Engng., 130 (9), pp. 905-914, 2004. DOI: 10.1061/(ASCE)1090-0241(2004)130:9(905) [ Links ]
[15] Wheeler, S.J., Näätänen, A., Karstunen, M. and Lojander, M., An anisotropic elastoplastic model for soft clays. Can. Geotech. J., 40, pp. 403-418, 2003. DOI: 10.1139/t02-119 [ Links ]
[16] Diaz-Rodríguez, J.A., Leroueil, S. and Aleman, J.D., Yielding of Mexico City clay and other natural clays. J. Geotech. Engng. ASCE, 118 (7), pp. 981-995, 1992. DOI: 10.1061/(ASCE)0733-9410(1992)118:7(981) [ Links ]
[17] Marsal, R.J. and Mazari, M., El subsuelo de la Ciudad de México, Tesis, Facultad de Ingeniería, UNAM, Mexico, 1959. [ Links ]
[18] SIG. Sistema de Información Geográfica. Laboratorio de Geoinformática, Instituto de Ingeniería, UNAM, [Önline]. 2012. Available at: http://sitios.iingen.unam.mx/geoinformatica/. [ Links ]
[19] Schlosser, F., Jacobsen, H.M. and Juran, I., Le renforcement des sols (1). Revue Française de Géotechnique, 29, pp. 7-32, 1984. [ Links ]
[20] Roscoe, K.H. and Burland, J.B., On the generalized stress-strain behavior of "wet" clay. Engineering Plasticity, in: Heyman, J. and Leckie, F.A. eds., Cambridge University Press, pp. 535-609, 1968. [ Links ]
[21] Ovando-Shelley, E., Trigo, M. and López-Velázquez, O., The value of K0 in Mexico City clay from laboratory and field tests. Proc. 11th PCSMFE, Foz de Iguazú, Brasil, pp 433-439, 1999. [ Links ]
[22] Marsal, R.J. and Salazar, J., Pore pressure and volumetric measurement in triaxial compression tests, Proc. Research Conference on Shear Strength on Cohesive Soils, ASCE, Boulder, Colorado, pp. 965-983, 1960. [ Links ]
[23] Lo, K.Y., Shear strength properties of a sample of volcanic material of the Valley of Mexico. Geotechnique, 12, pp. 303-319, 1962. DOI: 10.1680/geot.1962.12.4.303 [ Links ]
[24] Alberro, J. and Hiriart, G., Resistencia a largo plazo de las arcillas del Valle de México. Instituto de Ingeniería Series, UNAM, No. 317, 1973. [ Links ]
[25] Villa, R., Aplicación del principio de proporcionalidad natural para describir el comportamiento esfuerzo-deformación de la arcilla del Valle de México sometida a ensayes de compresión triaxial drenados y no drenados, en estado preconsolidado. MSc. Eng. Thesis, Programa de Maestría y Doctorado en Ingeniería, UNAM, Mexico, 2004. [ Links ]
[26] Jaky, J., The coefficient of earth pressure at rest. J. Society Hungarian Arch. and Eng., pp. 355-358, 1944. [ Links ]
[27] Mayne, P.W. and Kulhawy, F.H., K0-OCR relationships in soil. J. Geotech. Engng. ASCE, 108 (GT6), pp. 851-872, 1982. [ Links ]
[28] Auvinet, G. and Díaz-Mora, C., Programa de computadora para predecir movimientos verticales de cimentaciones, Instituto de Ingeniería Series, UNAM, No. 438, 1981. [ Links ]
[29] Karstunen, M. and Koskinen, M., Plastic anisotropy of soft reconstituted clays. Can. Geotech. J., 45, pp. 314-328, 2008. DOI: 10.1139/T07-073 [ Links ]
[30] Karstunen, M., Krenn, H., Wheeler, S.J., Koskinen, M., and Zentar, R., The effect of anisotropy and destructuration on the behaviour of Murro test embankment. Int. J. of Geomech., 5, pp. 87-97, 2005. DOI: 10.1061/(ASCE)1532-3641(2005)5:2(87) [ Links ]
[31] Diaz-Segura, E.G., Método simplificado para la estimación de la carga última de pilotes sometidos a carga vertical axial en arenas, DYNA, 80 (179), pp. 109-115, 2013. [ Links ]
J.F. Rodriguez Rebolledo, is graduated BSc. in Civil Engineering in 1996, an MSc. degree in Soil Mechanics in 2001, and a PhD degree (with honors) in Civil Engineering in 2011, all from the Universidad Nacional Autónoma de Mexico - UNAM, Mexico. As part of his PhD project, in 2008, he undertook a one-year academic placement in the University of Strathclyde, Scotland, U.K. From 1995 to 2013, he worked for the UNAM in geotechnical research projects related to numerical modeling, deep foundations and tunnels. From 1997 to 2013 he participated as geotechnical consultant in civil engineering projects across the country (Mexico). Currently, he is an associated professor at the Department of Civil and Environmental Engineering of the Universidade de Brasilia, Brasil. From 2008 to 2013, he participated in international research projects founded by the European Commission. ORCID.ORG/0000-0003-2929-7381
G.Y. Auvinet-Guichard, is graduated in 1964 from Ecole Spéciale des Travaux Publics de Paris, France. He received his PhD degree in Engineering in 1986, from Universidad Nacional Autónoma de México - UNAM, Mexico. He is a faculty member of UNAM Engineering School Postgraduate Division and head of the Geotechnical Computing Laboratory of the Institute of Engineering, UNAM, Mexico. He has dedicated his research to the solution of geotechnical problems with emphasis on deep foundations in consolidating soft soils and on application of probabilistic and geostatistical methods to Civil Engineering. He has been President of Mexican National Society for Soil Mechanics (1991-1992) and Vice-President for North America of the International Society for Soil Mechanics and Geotechnical Engineering (2009-2013). ORCID.ORG/0000-0003-4674-1659
H.E. Martinez-Carvajal, received a BSc. Eng. in Geological Engineering in 1995 from the Universidad Nacional de Colombia in Medellin, Colombia, an MSc. degree in Soil Mechanics in 1999 from the Universidad Nacional Autonoma de Mexico - UNAM, Mexico and the Dr. degree in Geotechnics in 2006 from the University of Brasilia, Brazil. From 1993 to 1999 he worked for civil engineering consulting companies as a geologist. He is currently an associated professor in the Civil and Environmental Engineering Department at the University of Brasilia and professor at the Facultad de Minas of the Universidad Nacional de Colombia in Medellin, Colombia. ORCID.ORG/0000-0001-7966-1466