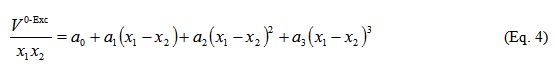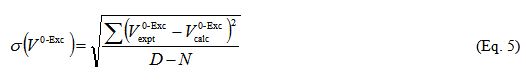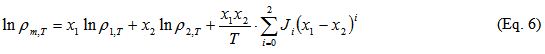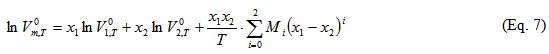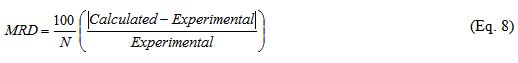Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Cited by Google
Cited by Google -
 Similars in
SciELO
Similars in
SciELO -
 Similars in Google
Similars in Google
Share
Revista Colombiana de Ciencias Químico - Farmacéuticas
Print version ISSN 0034-7418On-line version ISSN 1909-6356
Rev. colomb. cienc. quim. farm. vol.40 no.2 Bogotá July/Dec. 2011
Artículo de investigación científica
Volumetric properties of some pharmaceutical binary mixtures at low temperatures and correlation with the Jouyban-Acree model
Propiedades volumétricas de algunas mezclas binarias de uso farmacéutico a bajas temperaturas y correlación con el modelo Jouyban-Acree
Gerson A. Rodríguez1, Daniel R. Delgado1, Fleming Martínez1*, Maryam Khoubnasabjafari2, Abolghasem Jouyban3
1Grupo de Investigaciones Farmacéutico-Fisicoquímicas, Departamento de Farmacia, Facultad de Ciencias, Universidad Nacional de Colombia, A.A. 14490, Bogotá, D.C., Colombia.
*Correspondence: E-mail: fmartinezr@unal.edu.co
2Tuberculosis and Lung Disease Research Center, Tabriz University of Medical Sciences, Tabriz, Iran.
3Drug Applied Research Center and Faculty of Pharmacy, Tabriz University of Medical Sciences, Tabriz, Iran.
Recibido para evaluación: Agosto 19 de 2011
Aceptado para publicación: Septiembre 30 de 2011
SUMMARY
Excess molar volumes and partial molar volumes were investigated from density values for a) ethanol (1) + water (2), b) 1,2-propanediol (1) + water (2), and c) ethanol (1) + 1,2-propanediol (2) mixtures, at temperatures from (278.15 to 288.15) K. Excess molar volumes were fitted by Redlich-Kister equation. The systems exhibit negative excess volumes probably due to increased interactions like hydrogen bonding and/or large differences in molar volumes of components. The Jouyban-Acree model was used for density and molar volume correlations of the studied mixtures at different temperatures. The mean relative deviations between experimental and calculated data in density data were 0.15, 0.08, and 0.01 %, for a), b), and c), respectively; whereas, in molar volume data the values were 1.9, 2.1, and 0.1 %, for a), b), and c), respectively.
Key words: ethanol, 1,2-propanediol, water, binary liquid mixtures, density, excess volume, Jouyban-Acree model.
RESUMEN
En este trabajo se calcularon los volúmenes molares de exceso a partir de valores de densidad para los sistemas a) etanol + agua, b) 1,2-propanodiol + agua y c) etanol + 1,2-propanodiol, en todo el intervalo de composición, a temperaturas entre 278,15 y 288,15 K. Los volúmenes molares de exceso se modelaron de acuerdo a la ecuación de Redlich-Kister. Los sistemas estudiados presentan volúmenes de exceso altamente negativos probablemente debido a las fuertes interacciones por unión de hidrógeno entre las moléculas de los dos compuestos y a la gran diferencia en los volúmenes molares de los dos componentes puros. Finalmente se usó el modelo Jouyban-Acree para correlacionar la densidad y el volumen molar de las diferentes mezclas. Las desviaciones medias relativas en densidad fueron, 0,15, 0,08 y 0,01 %, para a), b) y c), respectivamente; mientras que el caso de volumen molar los valores fueron 1,9, 2,1 y 0,1 %, para a), b) y c), respectivamente.
Palabras clave: Glicerol, Agua, Mezclas líquidas binarias, Volúmenes de exceso, Modelo de Jouyban-Acree.
INTRODUCTION
Water-cosolvent mixtures have been used widely in pharmacy in order to increase the solubility of drugs poorly soluble in water during the design of homogeneous pharmaceutical dosage forms, such as syrups and elixirs, among others (1, 2). Ethanol and 1,2-Propanediol are the cosolvents most used in design nowadays, especially those intended for elaboration of peroral and parenteral medications (3). Thus, several examples of pharmaceutical formulations using these cosolvents have been presented by Rubino (1) and Yalkowsky (2).
The mixtures obtained using these cosolvents and water show highly non-ideal behavior due to increased interactions between unlike molecules and large differences in molar volumes of pure components, which leads to non-additive volumes on mixing (4, 5). For this reason it is necessary to characterize the volumetric behavior of these binary mixtures as a function of temperature in order to extend the physicochemical information available for liquid mixtures used in pharmacy. This information is useful to understand the intermolecular interactions present in liquid pharmaceutical systems (8). Also, data related to density of solute free mixture of solvents might be useful in prediction of the density of pharmaceutical substances in mixture of solvents (6).
In this report, the excess molar volumes and the molar and excess molar volumes of the respective binary systems conformed by ethanol, 1,2-propanediol, and water at various low temperatures (from 278.15 to 288.15 K) were calculated according to modified procedures widely exposed in the literature (7-9). This report is a continuation of those presented previously about some volumetric properties for the same binary systems at temperatures from 293.15 to 313.15 K (10-12). It is important to note that in the literature is scarce the volumetric information about these mixtures at low temperatures. Additionally, the Jouyban-Acree model is used to correlate densities and molar volumes with solvent compositions as has been for other binary systems at several temperatures (13-16).
EXPERIMENTAL
Materials
In this investigation dehydrated ethanol (from Merck, Germany) and dehydrated 1,2-propanediol (propylene glycol from Dow Chemical Company, U.S.A.) were used and they were in agreement with the quality requirements indicated for medicinal products indicated in the American Pharmacopeia USP (17); distilled water with conductivity lower than 2µS*cm-1 was also used. The dehydrated ethanol and 1,2-propanediol employed were maintained over molecular sieve to obtain dry solvents prior to prepare the solvent mixtures. Solvent dryness was verified based on densities obtained which were compared with the literature values (9-12).
Cosolvent mixtures preparation
All binary mixtures were prepared in quantities of 40.00 g by mass using a Ohaus Pioneer TM PA214 analytical balance with sensitivity + 0.1 mg, in concentrations from 0.10 to 0.90 varying in 0.10 in mass fraction of solvent 1, to study 9 mixtures and the two pure solvents. This procedure implies an uncertainty of + 2 x 10-5 in mole fraction. The mixtures were allowed to stand in Magni Whirl Blue M or Neslab RTE 10 Digital Plus (Thermo Electron Company) water baths at temperatures from 278.15 K to 288.15 K varying in 5.00 + 0.05 K for at least 30 minutes prior to density determinations.
Density determination
This property was determined using a DMA 45 Anton Paar digital density meter connected to a Neslab RTE 10 Digital Plus (Thermo Electron Company) recirculating thermostatic water bath according to a procedure previously described (18). The equipment was calibrated according to Instruction Manual using air and water at the different temperatures studied (19). Density measurements were carried out at least three times and averaged. From density values, volumetric properties were calculated as will be indicate in the next section.
RESULTS AND DISCUSSION
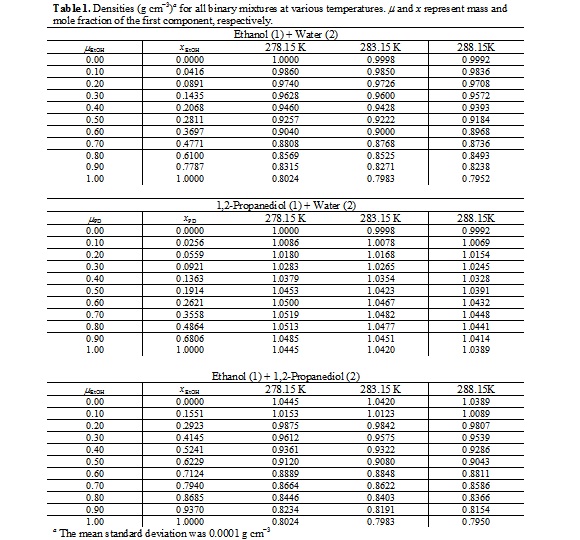
In Table 1 the composition of the three binary mixtures, in mass percent and mole fraction, in addition to density values at several temperatures are presented. Density values follow the same tendency as those reported for the same systems at temperatures from 293.15 to 313.15 K (10-12). Our density values for ethanol (1) + water (2) at 283.15 and 288.15 K are similar to those reported in the literature (20). In similar way, our density values for 1,2-propanediol (1) + water (2) are similar to those reported by Geyer et al. at 278.15 and 288.15 K (21, 22). It is important to note that up to the best of our knowledge for ethanol (1) + 1,2-propanediol (2) no density values at these temperatures have been reported. In all cases the density decreases almost linearly as the temperature increases.
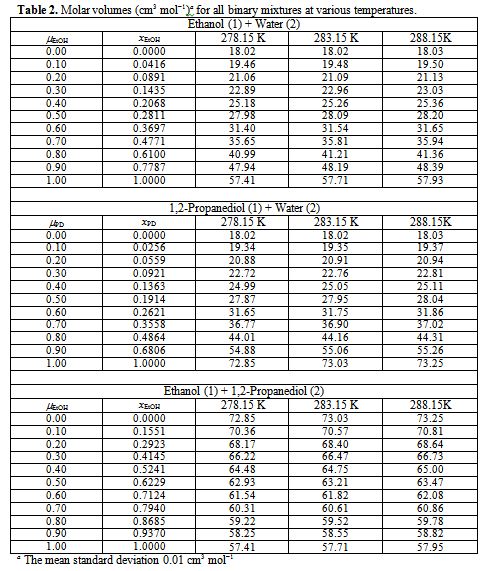
Molar volumes and excess molar volumes
Table 2 summarizes the molar volumes (V0) for the binary mixtures at all temperatures. V0 values were calculated from Eq. 1
where, x1 and x2 are the mole fractions and M1 and M2 are the molar masses, for both components respectively, and ρ is the mixture density.
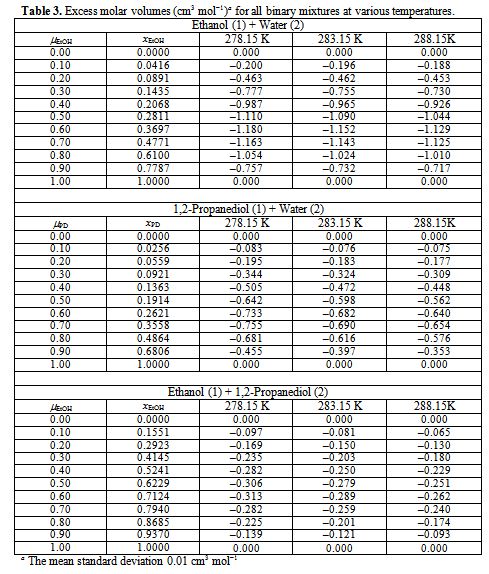
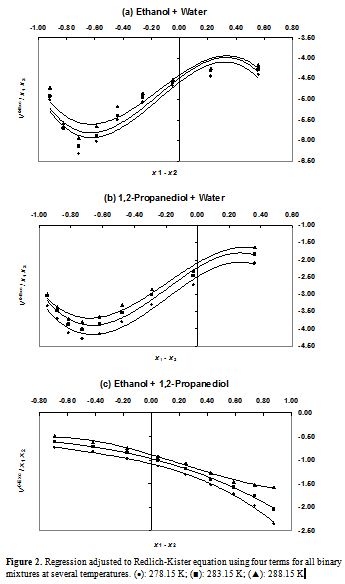
On the other hand, the excess volumes (V0-Exc) calculated from Eq. 2 (where, ρ1 and ρ2 are the densities of pure components) at all temperatures studied are presented in Table 3. This behavior is shown graphically in Figure 1 for the three systems at all temperatures.
Analogous to the behavior obtained at upper temperatures (10-12), in all cases the excess volumes are negative indicating contraction in volume. As mentioned before (10-14), according to Fort and Moore (23), a negative excess volume is an indication of strong heteromolecular interactions in the liquid mixtures and is attributed to charge transfer, dipole-dipole, dipole-induced dipole interactions, and hydrogen bonding between the unlike components, while a positive sign indicates a weak interaction and is attributed to dispersion forces (London interactions) which are likely to be operative in all cases.
In the aqueous evaluated systems, where the hydrogen bonding predominates, the contraction in volume has been interpreted basically in qualitative terms considering the following events, first: expansion due to depolymerization of water by addition of cosolvent; second: contraction due to free volume difference of unlike molecules; and third: contraction due to hydrogen bond formation between cosolvent and water through -OH---OH bonding (23).
Thus, the large negative values of V0-Exc over the free volume contribution indicate the presence of strong specific interactions with predominance of formation of hydrogen bonds between cosolvent and water over the rupture of hydrogen bonding in water-water.
The excess molar volumes becomes less negative as the temperature is raised indicating volume expansion which points out the decrease in the interactions between cosolvent and water molecules with increase in temperature.
Correlation of excess molar volume by using the Redlich-Kister equation
Redlich and Kister (24) introduced in 1948 the general form of Eq. 3 to facilitate the representation of thermodynamic properties and the classification of solutions in multicomponent systems, especially those important in petroleum chemistry. The Redlich-Kister equation has been used in recent decades for manipulating several kinds of physicochemical values of mixtures such as: excess volumes, excess viscosities, solubilities in cosolvent mixtures, among others.
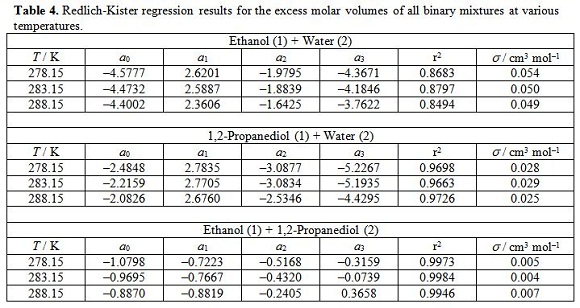
In the analysis of excess volume data, Eq. 3 was used in the form of third degree polynomial equations using least square analyses, and therefore, obtaining four coefficients as presented in Eq. 4. Polynomials of second and third degrees are the most widely used in this case again, based on their relevant statistic parameters such as determination coefficients and standard deviations.
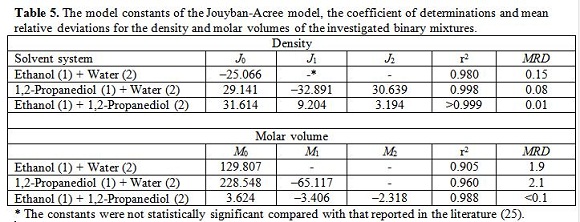
The Redlich-Kister parameters for the three mixtures at all temperatures studied are presented in Table 4 in addition to determination coefficients and standard deviations calculated according to Eq. 5 (where D is the number of compositions studied and N is the number of terms used in the regression, that is 9 and 4 respectively in this case). Eq. 5 has been widely used in the literature (7-16). Figure 2 shows the Redlich-Kister equation applied to excess molar volume data at all temperatures studied.
The coefficients of determinations are lower than 0.88 for ethanol (1) + water (2) mixtures, whereas they were greater than 0.96 and 0.99 for 1,2-propanediol (1) + water (2) and ethanol (1) + 1,2-propanediol (2) mixtures, respectively. These coefficients indicate that the obtained regular polynomials regressions describe adequately the excess volumes for the last two kinds of mixtures because the standard deviations are similar to those presented in the literature for other mixtures (8, 10-15). For ethanol (1) + water (2) mixtures the behavior obtained was not satisfactory but it is similar to that obtained at upper temperatures (10). On the other hand, σ values obtained for all the binary mixtures are similar to those reported at temperatures from 293.15 to 313.15 K by using also third degree of regular polynomials (10, 12).
Data correlation using the Jouyban-Acree model
For binary data analyses, the Jouyban-Acree model was used to correlate the experimental density data of mixed solvents (15, 25):
where ρm,T , ρ1,T , ρ2,T are densities of mixed solvents, solvents 1 and 2 at different temperatures (T), respectively. The x1, x2 are mole fractions of solvents 1 and 2, respectively. The methodology to find the Ji terms was described in previous works (15, 25). The main advantage of the Jouyban-Acree model over Redlich-Kister equation is that it includes the effects of temperature in the model constants and provides the possibility of density predictions at other temperatures using interpolation technique, whereas the constants of the Redlich-Kister equation is only valid for one temperature.
An adopted version of Eq. 6 could be used for representing the molar volume data of mixed solvents. The trained version of the model for glycerol + water mixtures at various temperatures is:
where V0m,T , V01,T , V02,T are molar volumes of mixed solvents, solvents 1 and 2 at different temperatures and Mj are the model constants.
The mean relative deviation (MRD) between experimental and calculated data was calculated using:
where N in is the number of data points in the data set.
The computed model constants of the Jouyban-Acree model, the coefficients of determinations and MRD values for density and molar volume data of the investigated solvent systems are listed in Table 5. Careful examination of the results reveal that the model describes the density and molar volumes of the investigated solvent systems and could be recommended for practical applications where the simulation of such data is required in process design.
CONCLUSIONS
This report expands widely the experimental volumetric information about these three cosolvent binary systems available nowadays because it includes the behavior at other three temperatures commonly found in technological and storage conditions. As mentioned earlier, this information could be employed in several chemical engineering processes and for the theoretical understanding of the behavior of cosolvent mixtures used in the pharmaceutical industries. Based on the presented results and those reported in the literature, it can be concluded that these mixtures clearly show non ideal behavior. These observations demonstrate clearly that it is necessary to characterize systematically all possible pharmaceutical binary system in order to have complete experimental information about the physical and chemical properties useful in the understanding of all kind of liquid pharmaceutical dosage systems.
ACKNOWLEDGMENTS
We thank the DIB of the Universidad Nacional de Colombia (UNC) for the financial support. Additionally we thank the Department of Pharmacy of UNC for facilitating the equipment and laboratories used.
REFERENCES
1. J.T. Rubino, Cosolvents and Cosolvency, in: "Encyclopedia of Pharmaceutical Technology", Vol. 3, edited by J. Swarbrick, J.C. Boylan, Marcel Dekker, Inc., New York, 1988. pp. 375-98. [ Links ]
2. S.H. Yalkowsky, "Solubility and Solubilization in Aqueous Media", American Chemical Society and Oxford University Press, New York, 1999, pp. 180-235. [ Links ]
3. D.C. Pérez, C.C. Guevara, C.A. Cárdenas, J.A. Pinzón, H.J. Barbosa, F. Martínez, Solubility and displacement volumes of acetaminophen in binary mixtures formed by propylene glycol, ethanol, and water at 25.0 oC (in Spanish), Rev. Colomb. Cienc. Quím. Farm., 32, 116 (2003). [ Links ]
4. R. Battino, Volume changes on mixing for binary mixtures of liquids, Chem. Rev., 71, 5 (1971). [ Links ]
5. S.J. Rodríguez, D.M. Cristancho, P.C. Neita, E.F. Vargas, F. Martínez, Volumetric properties of the octyl methoxycinnamate + ethyl acetate solvent system at several temperatures, Phys. Chem. Liq., 48, 638 (2010). [ Links ]
6. Sh. Soltanpour, A. Jouyban, Solubility of acetaminophen and ibuprofen in binary and ternary mixtures of polyethylene glycol 600, ethanol and water, Chem. Pharm. Bull. (Tokyo), 58, 219 (2010). [ Links ]
7. J.A. Salas, J.L. Zurita, M. Katz, Excess molar volumes and excess viscosities of the 1-chlorobutane + pentane + dimethoxyethane ternary system at 298.15 K, J. Argent. Chem. Soc., 90, 61 (2002). [ Links ]
8. R.D. Peralta, R. Infante, G. Cortez, R.R. Ramírez, J. Wisniak, Densities and excess volumes of binary mixtures of 1,4-dioxane with either ethyl acrylate, or butyl acrylate, or methyl methacrylate, or styrene at T = 298.15 K, J. Chem. Thermodynamics, 35, 239 (2003). [ Links ]
9. J.M. Resa, C. González, J.M. Goenaga, M. Iglesias, Temperature dependence of excess molar volumes of ethanol + water + ethyl acetate, J. Solution Chem., 33, 169 (2004). [ Links ]
10. J. Jiménez, J. Manrique, F. Martínez, Effect of temperature on some volumetric properties for ethanol + water mixtures, Rev. Colomb. Cienc. Quím. Farm., 33, 145 (2004). [ Links ]
11. J. Jiménez, F. Martínez, Study of some volumetric properties of 1,2-propanediol + water mixtures at several temperatures, Rev. Colomb. Cienc. Quím. Farm., 34, 46 (2005). [ Links ]
12. J. Jiménez, F. Martínez, Volumetric properties of ethanol + 1,2-propanediol mixtures at different temperatures, Phys. Chem. Liq., 44, 521 (2006). [ Links ]
13. D.M. Cristancho, D.R. Delgado, F. Martínez, M.A.A. Fakhree, A. Jouyban, Volumetric properties of glycerol + water mixtures at several temperatures and correlation with the Jouyban-Acree model, Rev. Colomb. Cienc. Quím. Farm., 40, 92 (2011). [ Links ]
14. D.R. Delgado, F. Martínez, M.A.A. Fakhree, A. Jouyban, Volumetric properties of the glycerol formal + water cosolvent system and correlation with the Jouyban-Acree model, Phys. Chem. Liq., DOI: 10.1080/00319104.2011.584311. [ Links ]
15. G.A. Rodríguez, D.R. Delgado, F. Martínez, M.A.A. Fakhree, A. Jouyban, Volumetric properties of glycerol formal + propylene glycol mixtures at several temperatures and correlation with the Jouyban-Acree model, J. Solution Chem., submitted. [ Links ]
16. A.R. Holguín, D.R. Delgado, F. Martínez, M.A.A. Fakhree, A. Jouyban, Study of some volumetric properties of glycerol formal + ethanol mixtures and correlation with the Jouyban-Acree model, Rev. Acad. Colomb. Cienc., submitted. [ Links ]
17. US Pharmacopeia, 23rd edition, United States Pharmacopeial Convention, Rockville, MD, 1994. [ Links ]
18. F. Martínez, A. Gómez, C.M. Ávila, Partial molal volumes of transfer of some sulfonamides from water to the (X = 0.5) ethanol-water mixture, Acta Farm Bonaerense, 21, 107 (2002). [ Links ]
19. O. Kratky, H. Leopold, H. Stabinger, "DMA45 Calculating Digital Density Meter, Instruction Manual", Anton Paar, K.G., Graz, Austria, 1980, pp. 1-12. [ Links ]
20. U.S. Department of Commerce, Standard density and volumetric tables, N.B.S., National Bureau of Standards Circular No. 19 (1913). [ Links ]
21. H. Geyer, P. Ulbig, M. Görnert, Measurement of densities and excess molar volumes for (1,2-ethanediol, or 1,2-propanediol, or 1,2-butanediol + water) at the temperatures (278.15, 288.15, 298.15, 308.15, and 318.15) K and for (2,3-butanediol + water) at the temperatures (308.15, 313.15, and 318.15) K, J. Chem. Thermodynamics, 32, 1585 (2000). [ Links ]
22. H. Geyer, P. Ulbig, M. Görnert, A. Susanto, Measurement of densities and excess molar volumes for (1,2-propanediol, or 1,2-butanediol + water) at the temperatures (288.15, 298.15, and 308.15) K and at the pressures (0.1, 20, 40, and 60) MPa, J. Chem. Thermodynamics, 33, 987 (2001). [ Links ]
23. R.T. Fort, W.R. Moore, Viscosities of binary liquid mixtures, Trans. Farad. Soc., 62, 1112 (1966). [ Links ]
24. O. Redlich, A.T. Kister, Algebraic representation of thermodynamic properties and the classification of solutions, Ind. Eng. Chem., 40, 345 (1948). [ Links ]
25. A. Jouyban, A. Fathi-Azarbayjani, M. Khoubnasabjafari, W.E. Acree, Jr., Mathematical representation of the density of liquid mixtures at various temperatures using Jouyban-Acree model, Indian J. Chem. A, 44, 1553 (2005). [ Links ]