Services on Demand
Journal
Article
Indicators
-
 Cited by SciELO
Cited by SciELO -
 Access statistics
Access statistics
Related links
-
 Cited by Google
Cited by Google -
 Similars in
SciELO
Similars in
SciELO -
 Similars in Google
Similars in Google
Share
Lecturas de Economía
Print version ISSN 0120-2596
Lect. Econ. no.62 Medellín Jan./June 2005
Stable and Farsighted Set of Networks
Sistema estable y clarividente de redes
Ensemble stable et prévoyant de réseaux
Jorge Hugo BarrientosI
I Jorge Hugo Barrientos-Marin: Ph.D student at the Quantitative Economic Doctorate and Teaching Assistant in Mathematical Statistics and Econometrics. E-mail: jbarr@merlin.fae.ua.es. This paper was elaborated for the Social Networks seminar at the Department of Fundamentos del Análisis Económico, University of Alicante. Alicante, Spain. Acknowledgements: The author is grateful with the anonymous readers and with the Students of Social Networks course. All the mistakes and errors in this paper are responsibility only of the author.
-Introduction. -I. Traditional Notation and Model of Network. -II. The stability of the networks set. -III. Summary and Forthcoming. -Appendix. - References.
Resumen: En este artículo proponemos un modelo de formación de redes sociales donde los agentes pueden mirar hacia delante y tomar las decisiones necesarias que implican un cambio en la forma de las redes. Esta característica significa que las decisiones de los agentes no están basadas en los pagos actuales, sino que se basan en donde ellos esperan que el proceso llegue, o en otras palabras en las potenciales redes resultantes. Por tanto, la inclusión de este rasgo en un modelo de formación de redes es la principal contribución del artículo. De otro lado, el proceso de formación propuesto aquí descansa en el concepto de Conjunto Consistente Más Grande, muy usado en la Teoría de la Situaciones Sociales.
Palabras clave: Teoría de juegos j teoría de negociación, juegos cooperativos y no cooperativos. Clasificación JEL: C70, C71, C72.
Abstract: In this paper we propose a model of network formation where the individual are farsighted. In other words, the players are able to see ahead and take decisions about changes on network structure. This characteristic means that agents' decisions that could change a network are not based on current payoffs but where they expect the process going to arrive. Hence, this feature becomes the main contribution of this paper. The other hand, the formation process proposed here rest on the crucial notion of Largest Consistent Set. It one is a notion common in Social Situations Theory
Keywords: Game theory and bargaining theory, cooperative games and non-cooperative games. JEL: C70, C71, C72.
Résumé: En cet article nous proposons un modèle déformation de réseau où l'individu sont prévoyant. En d'autres termes, les joueurs peuvent voir en avant et prendre des décisions au sujet des changements sur la structure de réseau. Cette caractéristique signifie que les décisions des agents qui pourraient changer un réseau ne sont pas basées sur des profits courants mais où elles s'attendent au processus allant arriver. Par conséquent, ce dispositif devient la contribution principale de cet article. L'autre main, le processus de formation a proposé ici le repos sur la notion cruciale de plus grand à ensemble conformé. C'un est une notion commune dans la théorie sociale de situations
Mots clés: Théorie des jeux rectangulaires et théorie de négociation, jeux non coopératifs de jeux coopératifs. JEL: C70, C71, C72.
Introduction
The organization of individuals into networks and groups, called coalitions, has an important role in the determination of the outcome of many social and economic interactions. For instance, networks of personal contacts are important to obtain information about business and job opportunities. The partitioning of societies into groups or coalitions is important to the formations of alliances, cartels, federations, unions, terrorist groups and organized delinquency as drugs trafficking.
Despite the fundamental importance of network structures in many social contexts, the development of foundational theoretical models to analyze how individual decisions contribute to the process of networks formation is still poor. We are not interested in models where individuals are naive about network structure at once two or three players are connected or where agents are myopic. We are concerned in network formation models where players are forwardlooking or farsighted.
Dutta and Jackson (2002) tell us that some questions in network formation keep open on. The most important is concerned with the developing of a complete networks formation model, which is formed over time, and, in particular a formatin model, to allow for players to be farsighted. This feature implies that players' decisions about whether to form a network are not based only on current payoffs but also on where they expect the process to go.1 Here we would like to have forward-looking players in the process of the change of networks.
Stable set is a fundamental tool in the theory of social situations and is related to solution concept. Such solution is defined by conditions on a set of outcomes. It one is non-defined by conditions on individuals. In fact we are using largest consistent set concept, this notion represents an improvement over stable set notion as usually is studied in the literature.
Why largest consistent set concept is better than stable set notion? Because stable set does not capture the assumption of farsighted players. This last idea constitutes a conceptual defect, because as expressed by Chwe (1994):"further deviation need to deter but can actually encourage a deviation". A situation of farsightedness is similar to situations where players act as forward-looking ones. In some sense it is a way of treating the problem of myopic players.
What property must stable set have? In the context of social networks it is desired that in a stable set a deviation be invalidated if a further deviation to some stable network exists. But a coalition might deviate knowing that there will be a further deviation; it might like the further deviation even better. It is a feature of farsighted coalitions.
In this paper, we have applied the notion of largest consistent set to the process of the change of networks. In other words, the objective of this work is to show that the concept of largest consistent set and the theory of networks can be made compatible. Hence, if we impose conditions on the set of networks we can achieve some approach to the idea of stable network under farsighted coalitions. This implies that players' decisions on the change of networks structure —and then about network formation— are based on where they expect the process ends arriving.
With this in mind we introduce a preferences relation upon networks set and a feasibility relation. Moreover, we setup a condition about the stability of networks. The concept of coalition will be important to develop the model, but for an easier exposition of the examples we often think in the "big coalition": a set that includes all players. We also refer to stable concept and its relation with the external and internal stability. Some examples are given to illustrate this definition. The networks can be directed and non-directed, the difference is in who pays the connection cost.
A useful concept introduced by Jackson and Watts (2001) and used here is the simultaneous improving path —SIP—. The authors tell us that SIP notion is somewhat myopic in the sense that players do no forecast how their decision to add or sever links might affect future decisions of other players or, more generally, how might influence the future evolution of the network. However, we are introducing the SIP notion together with a preferences relation and feasibility relation. This allows us to use a SIP as a possible way in which the players could anticipate where the process to go.
It is important to take into account some questions. First, this paper is only a proposal to approach the problem of forward-looking players. In that sense some problems can arise. For instance, if we define a non-transitive preferences relation a cycle will not exist, but it is possible that some steady states emerge. Second, to fully address the problem of myopia any concept of solution should allow players to look arbitrary far ahead.2
Papers closely related to this one are: Chwe (1994), Jackson and Wolinsky (1996), Watts (2001), Jackson and Watts (2001), Dutta and Jackson (2002) and Page and Kamat (2004). It is very interesting to note that Page and Kamat' paper is related with our work in the sense that both papers focus in the farsighted concept and largest consistent set. However, they go so far including a supernetworks concept, while for us the network formation process is a pure decision problem and it does not depend on network structure. In other words, we only need the improving path concept and a preferences relationship to get a stable network.
I. Traditional Notation and Model of Network
The notation used here for networks and players is the same used in the traditional literature. Additional notation is introduced for preferences and feasibility relationships. There is a set of players N = {1,2,...,n} with cardinality n, who are able to communicate with each other. This communication structure, between these agents, is represented as a network —graph—, where a node represents a player and a link between two nodes implies that these two players are able to communicate with each other. A link ij is the subset {i,j} of N containing i and j. The set G = {gιg gN} represents the set of all possible graphs and gN represent the complete graph. If player j and i are directly linked in graph g, we write ij
gN} represents the set of all possible graphs and gN represent the complete graph. If player j and i are directly linked in graph g, we write ij g. Each agent i
g. Each agent i  N receives a payoff ui(g) from network g; the value of this network is represented by v: G
N receives a payoff ui(g) from network g; the value of this network is represented by v: G  R. In some applications: v(g) = Σi
R. In some applications: v(g) = Σi  N ui(g).
N ui(g).
A process P is denoted by P = {N,Z,{<i}i  N, {
N, { S}S E N, S ≠ φ}, where N is the set of players. Let Z
S}S E N, S ≠ φ}, where N is the set of players. Let Z  G be any set of networks, Z ≠ Φ, where {<i} is the players' strong preferences relation defined on Z and {
G be any set of networks, Z ≠ Φ, where {<i} is the players' strong preferences relation defined on Z and { S} are the "feasibility relations" defined on Z. Coalition is defined by non empty set S
S} are the "feasibility relations" defined on Z. Coalition is defined by non empty set S  N, under this definition S can be {i} or N —called the big coalition—. The relations
N, under this definition S can be {i} or N —called the big coalition—. The relations  S represent what coalitions S can do: g''
S represent what coalitions S can do: g''  S g' means that if g'' is the current network, coalitions S can enforce g no matter what anyone else does; after S moves to g' from g'', another coalition S' might move to g, where g'
S g' means that if g'' is the current network, coalitions S can enforce g no matter what anyone else does; after S moves to g' from g'', another coalition S' might move to g, where g'  S g.
S g.
When the process begins, there is a current network, say g' —it can be g' = φ the empty set—. If members of coalition S decide to change the current network to another one, say g, where g'  S g', then the new network becomes g. This change of network is called coalitions' movement or deviation from g' to g. From this new current network g another coalition might move and so forth, virtually, without limit.
S g', then the new network becomes g. This change of network is called coalitions' movement or deviation from g' to g. From this new current network g another coalition might move and so forth, virtually, without limit.
If network g is reached and no coalitions decide to move from g, then g is a stable network and the process is over. Then and only then players receive their payoffs from g. From a current network g' many different coalitions will be able to move from g'. Coalitions do not move in a specified order. The process specifies what happens if coalition S1 changes from g' to g but not what happens if no coalitions change to another network. Finally, there are no time preferences. Players only care about the end process and not how many moves they have to take to get there.
A simultaneous improving path3 —SIP— is a sequence of networks that can emerge when individual into a coalition form or severs links. This decision of form or sever links are based on the improvement that the resulting network offers them, relative to the current network. More exactly a SIP is a sequence of networks g0,...,gk in G such that if g' follows g in the sequence then either:
i) g' = g - ij and either ui(g') > ui(g) or uj(g') > uj(g),
ii) g'  G and g'
G and g'  {g + ij - ik, g + ij - ik - jm, g + ij - jm}, where ij
{g + ij - ik, g + ij - ik - jm, g + ij - jm}, where ij  g and ui(g) > ui(g) and uj(g') > uj(g).
g and ui(g) > ui(g) and uj(g') > uj(g).
Here, simultaneity refers to the fact that a player may make several changes at once. A given agent could be a member of several coalitions.
DEFINITION 1. Given a coalition S  N and the current network g', we say that g is feasible for the coalition S if g'
N and the current network g', we say that g is feasible for the coalition S if g'  S g, with g' and g adjacent.
S g, with g' and g adjacent.
It is clear that if there is a SIP or improving path from g' to g then there is a sequence of different coalitions, such that, each one has at least one feasible network, and each one is adjacent. Imagine the following feasibility relation in the figure:
g0  S0 g1
S0 g1  S1 g2
S1 g2  S2 g3
S2 g3  S3 g4,...., gm - 1
S3 g4,...., gm - 1  Sm - 1 gm
Sm - 1 gm
We have that g0,...,gm is a SIP. The obvious difference between coalitions is the number of players and the difference between networks is the number of links in each one.
We are going to illustrate the intuition behind the change from g0 to g1 network showed in the figure. Note that we can write g0(S0) this means that S0 coalition moves to g1(S)0. Actually we mean with "move to" that S0 coalition based on some welfare criterion decides to change the network. In the next step, coalition contact with other players to form links or expel players to sever a link. Once the change into coalitions is carried out, we have a coalition S1 wich can move from g1(S1) to g2 and so on. In that sense, a chain of feasible networks can be seen as a SIP.
REMARK 1: we say that there is a cycle if there exists an improving path where g0 = g0.
DEFINITION 2. Given a society formed by N individual, we say that network g' can be improved by coalition S, if there is another network g that is feasible for S and such that u(g)i ≥ ui(g')Vi  S and
S and 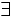 j
j  S u(g)j > uj(g').
S u(g)j > uj(g').
Note that former definitions suggest us that the utility function is monotone strictly. Then it is not possible to get two networks such that g0 / gm because u(g)i = ui(g') Vi  S, this situation implies that a no improving path exists.
S, this situation implies that a no improving path exists.
A network can be improved for S when all its members encourage new connections, this means to sever and to form links such that the coalition is able to go from g' to g and all members improve —more exactly, that some of them improve and nobody gets worse—. Two examples can illustrate this definition.
REMARK 2: If the conditions in the definition 2 are satisfied, then there is not a cycle.
EXAMPLE 1. Consider the connection model of Jackson and Wolinsky (1996) where N = S= {1,2,3}. The payoff of player i is: ui(g) = Σi ≠ j δt(ij) - Σj:ij  gc with 0 < δ < 1, c > 0 and δt(ij) is a payoff of i from being indirectly connected to j and t(ij) is the numbers of links in the shortest way to unite i and j. Then if we suppose that δ > c and we have the following networks g':
gc with 0 < δ < 1, c > 0 and δt(ij) is a payoff of i from being indirectly connected to j and t(ij) is the numbers of links in the shortest way to unite i and j. Then if we suppose that δ > c and we have the following networks g':

And g'':
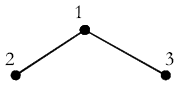
The payoff of g' is ui(g') = 2(δ - c) Vi  S and the potential payoff of g'' is u1(g') = 2(δ - c). The payoff for j = 1,2 is uj(g') = δ + δ2 - c. But for j = 1,2 uj(g') > uj(g'')
S and the potential payoff of g'' is u1(g') = 2(δ - c). The payoff for j = 1,2 is uj(g') = δ + δ2 - c. But for j = 1,2 uj(g') > uj(g'') 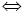 δ - c > δ2. Clearly g'' can be improved by the big coalition S and g' is feasible to S. Here g' and g'' are adjacent networks. The unique difference between them is on the link {23}.
δ - c > δ2. Clearly g'' can be improved by the big coalition S and g' is feasible to S. Here g' and g'' are adjacent networks. The unique difference between them is on the link {23}.
EXAMPLE 2. Under the connection model, if δ > c and (δ - c) > δ2, the empty network always can be improved by any coalitions. Otherwise if δ - c < 0 then the empty network cannot be improved by any coalition.
DEFINITION 3. If g' <i g Vi  S we write g' <S g. We say that g' is directly dominated by g, or g' < g, if there exists a coalition S such that g is feasible and g' <S g.
S we write g' <S g. We say that g' is directly dominated by g, or g' < g, if there exists a coalition S such that g is feasible and g' <S g.
LEMMA 1. Any network g' directly dominated can be improved.
Proof: Let N be a set of players. If network g' is directly dominated, there is some S 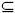 N and a network g, such that is feasible and g' <i g Vi
N and a network g, such that is feasible and g' <i g Vi  S. This implies that u(g)i > ui(g') Vi
S. This implies that u(g)i > ui(g') Vi  S and
S and 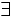 j
j  S u(g')j > uj(g').
S u(g')j > uj(g').
The lemma 1 tells us that if there is a network g' directly dominated, then another network g exists, better than g', to which the coalition S would arrive in order to get a higher utility.
DEFINITION 4. Given a society formed by N individual, we say that network g is core stable if there does not exist any set of player S 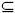 N and g'
N and g'  Z such that:
Z such that:
i) The network g is feasible
ii) The network g cannot be improved
iii) If ij  g' but ij
g' but ij 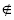 g then i
g then i  S and j
S and j  S, and ij
S, and ij 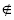 g' but ij
g' but ij  g, then either i
g, then either i  S and/or j
S and/or j  S.
S.
In other words a network g is core stable if there is no group of players who each prefer networks g' to g and who can change the network from g to g' without the cooperation of the remaining players. An application to the marriage problem can be found in Jackson and Watts (2001).
The social stability, in the spirit of definition 4, is compounded for all feasible networks that cannot be improved by any coalition. The core is given by the set of networks that does not allow players to sever or to form new links for any subset of players. It is clear that if that g cannot be improved by any coalition then any network, say g', is directly dominated by g. The logic behind the core stable definition is that if g' < g and then g' cannot be stable because the coalition S is capable of changing the network moving to g and all its members prefer g to g'.
As the reader will note, the core stable definition is very different to stability notion that we are going to introduce in the next section. The difference focuses in the fact that we use a preferences relationship and regarding farsighted agents. In fact, no definition about stability is provided, hence it one will be implicit in the consistent set notion.
The core stable commented here is defined as has been done by Jackson and Watts (2001), where the authors rule out consideration about farsighted agents. From now on, every stability situation will understand as follow: if any network g is reached and no coalition decides to get away from g, then g is considered as a stable network. The following definition, as definition 3 and example 3, is in the spirit of the definitions introduced by Chwe (1994). The fifth definition captures the idea that some coalition can anticipate other coalitions' action.
DEFINITION 5. We say that g' is indirectly dominated by g, or g' < g, if there exists a sequence of networks g0,...,gm —where g0 g' and gm = g — and S0,S1,...,Sm - 1 such that gk  Sk gk + 1 and gk <Sk g for k = 0,1,2,...,m - 1.
Sk gk + 1 and gk <Sk g for k = 0,1,2,...,m - 1.
EXAMPLE 3. Suppose that every member of S0 prefers the network g2 to g0 (g0 <S0 g2) but is not feasible for S0 (g0  S0 g2). In agreement with the logic of core, S0 is stuck at g0. However, S0 can move from the network g0to g1 (g0
S0 g2). In agreement with the logic of core, S0 is stuck at g0. However, S0 can move from the network g0to g1 (g0  S0 g1) and another coalition S1 can move from g1 to g2(g1
S0 g1) and another coalition S1 can move from g1 to g2(g1  S1 g2) and all members of S1 prefer g2 over g1 (g1 <S1 g2). Then coalition S0 could move from g0 to g1, anticipating that S1 would move to g2. Even though g0 might not be directly dominated by g2 it is indirectly dominated by g2, and hence g0, which might even be in the core, need not be stable. The following result shows us that if there is a network g' indirectly dominated by another network g, then there exists another network g* which, simultaneously, is dominated by g and dominates the network g'.
S1 g2) and all members of S1 prefer g2 over g1 (g1 <S1 g2). Then coalition S0 could move from g0 to g1, anticipating that S1 would move to g2. Even though g0 might not be directly dominated by g2 it is indirectly dominated by g2, and hence g0, which might even be in the core, need not be stable. The following result shows us that if there is a network g' indirectly dominated by another network g, then there exists another network g* which, simultaneously, is dominated by g and dominates the network g'.
LEMMA 2. If g ≥ g' there is a network g* such that g' < g* and g* < g.
PROOF: Suppose that g' is indirectly dominated by g. Then, there is a sequence of networks g',...,g*,...,gm and coalition S0,...,Sk,...,Sm - 1 such that gk  Sk gk + 1 and gk
Sk gk + 1 and gk  Sk g for k = 0,1,2,...,m - 1. Take any network from the sequence, say gk = g* such that g' < g*, thus we get the claimed result.
Sk g for k = 0,1,2,...,m - 1. Take any network from the sequence, say gk = g* such that g' < g*, thus we get the claimed result.
The fifth definition has the following interpretation. If g0 < gm and gm is presumed stable, then it is possible, not certain, that coalitions S0,...,Sk,...,Sm - 1 find a path and will change from network g0 to gm.
In ordet to check if a network g is stable consider a deviation by coalition S to g'. There might be further deviations, which end up at g'', where g' < g''. There might not be any further deviation, in which case the ending outcome is g' = g''. In either case, the ending network g'' should be stable. If some member of coalition S does not prefer g'' to the initial network g, then the deviation is deterred. A network is stable if every deviation is deterred. The concept of stability and consistent criteria will be focus in the next section.
II. The Stability of the Networks Set
If in process P the network g is reached and no coalition decides to get away from g, then g is considered as a stable network and the game is over. After that, players can get their payoffs from g. In some particular cases, it is possible that from a current network g' several4 —and different— coalitions will be able to get away from g'.
As in the previous process, coalitions do not move in a specified order. It is not the case that if a particular coalition S1 is not able to get away from g', so it does not move, another coalition S2 can get away from g' and so on. Issues such as preemptory moves arise.5 The set of stable networks should satisfy a sort of consistency criteria.
DEFINITION 6. A set of networks Y C Z is consistent if g  Y
Y 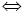 Vg', VS such that g
Vg', VS such that g  S g',
S g', 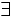 g''
g''  Y, where g' = g'' or g' < g'', such that g <S g''.
Y, where g' = g'' or g' < g'', such that g <S g''.
If Y is consistent and g  Y, does not mean that network g will be stable but it is still possible for g to be stable. If a network g' is not contained in any consistent Y, the interpretation is that g' cannot be stable. In fact, we can think that any definition of stability should include a consistent notion. Therefore, largest consistent set —LCS— is the set of all networks that can be possibly stable.
Y, does not mean that network g will be stable but it is still possible for g to be stable. If a network g' is not contained in any consistent Y, the interpretation is that g' cannot be stable. In fact, we can think that any definition of stability should include a consistent notion. Therefore, largest consistent set —LCS— is the set of all networks that can be possibly stable.
PROPOSITION 1. Consider the process. Then, there exists a unique Y such that Y is consistent and. The set Y is called the largest consistent set of P, and we denote this one by L(P).
PROOF: See appendix
The previous definition is useful to show that L(P) always exists and it is unique —proposition 1—. This result gives us a tool to find a largest consistent set. It is important to note that this result does not tell us anything about emptiness of L(P). In fact, it can be empty. Here, we suppose that networks sets are finite and the preferences are not reflexive. This means that Vi  N, Vg, g < g. These assumptions are simplifiers because no reflexive preferences are needed to do no infinite
N, Vg, g < g. These assumptions are simplifiers because no reflexive preferences are needed to do no infinite 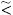 -chains. Since we are focused in networks formation it is useful to think in a finite set.
-chains. Since we are focused in networks formation it is useful to think in a finite set.
In fact, Chwe (1994) does the extension for Z countable infinite. He says that a sufficient condition for non-emptiness of L(P) is that does not exist infinite 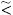 -chains: there is no g0,g1,... such that i < j
-chains: there is no g0,g1,... such that i < j  gi
gi 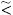 gj. The following proposition is a corollary from this commented extension.
gj. The following proposition is a corollary from this commented extension.
PROPOSITION 2. Consider the process P = {N,Z,{<i}i  N, {
N, { S}SCN, S ≠ φ} where Z is finite and preferences on the network set are no reflexive. Then L(P) is nonempty and has the external stability property: Vg'
S}SCN, S ≠ φ} where Z is finite and preferences on the network set are no reflexive. Then L(P) is nonempty and has the external stability property: Vg'  Z \ L(P),
Z \ L(P), 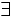 g
g  L(P) such that g'
L(P) such that g' 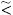 g.
g.
PROOF: Suppose there exist infinite 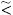 -chains: there is g0,g1,... such that i < j
-chains: there is g0,g1,... such that i < j  gi
gi 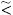 gj. Since Z is finite
gj. Since Z is finite 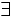 i, j such that i < j and gi = gj. Thus gi
i, j such that i < j and gi = gj. Thus gi 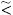 gi, a contradiction since gi <S gi but it is not possible.
gi, a contradiction since gi <S gi but it is not possible.
Now it is possible to define a concept of stable set and say that a stable set is a subset of L(P) set in a process P. Given a set Z C G of networks and a relation 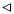 on Z, we say Ω is a stable set6 of pair (Z,
on Z, we say Ω is a stable set6 of pair (Z,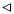 ) if: (i)
) if: (i) 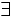 g, g'
g, g'  Z such that g
Z such that g 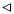 g' —internal stability—; and (ii) Vg'
g' —internal stability—; and (ii) Vg'  Z \ Ω,
Z \ Ω, 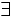 g
g  Ω such that g'
Ω such that g'  Z. Note that when =Í*, then we have the external stability. Stable set does not always exist.7 We do not do it here, but Chwe showed that if the players are farsighted, stable set of (Z,
Z. Note that when =Í*, then we have the external stability. Stable set does not always exist.7 We do not do it here, but Chwe showed that if the players are farsighted, stable set of (Z,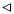 ) are good, but stable set of (Z,<) are not so good.
) are good, but stable set of (Z,<) are not so good.
PROPOSITION 3. Say P = {N,Z,{<i}i  N, {
N, { S}SCN, S ≠ φ}. If Ω is stable set of (Z,
S}SCN, S ≠ φ}. If Ω is stable set of (Z,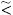 ), then Ω
), then Ω 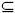 L(P).
L(P).
PROOF: See appendix.
EXAMPLE 4. Consider a process P and the Connection Model with a set of N players and the big coalition N. Then VN:
i) if c < δ and (δ - c) > δ2 then gN  Ω
Ω
ii) if c ≥ δ, then {empty network}  Ω
Ω
iii) if c < δ and (δ - c) ≤ δ2, then {star network}  Ω.
Ω.
The expression i)-iii) in the example tells us that under those conditions on c and δ the complete, empty and the star network belong to set of stable network.
The main and subtle difference between this treatment of networks and the usual one is just conceptual because we are introducing the concept of coalition, preferences and consistent set. We are given a tool that allows players to behave in a farsighted way. Farsightedness behavior allows the coalition to consider the possibility that once it acts, another coalition might react and, a third coalition might in turn react, and so on, virtually, without limit. It is clear that the notion of SIP plays an important roll in the change of the network. This change has to be carried out through a SIP. In other words, we mean that if we allow players to look arbitrarily far ahead they will only follow an improved path. Since players receive their payoffs only when the process is over the concept of SIP will not be myopic. See Watts (2001) and Jackson and Watts (2001) for a wide discussion about myopic players.
III. Summary and Forthcoming
So far we have characterized the set of stable network using the largest consistent set and consistency criteria. We defined a preference relationship and feasibility relation and we allow the players to be farsighted. The model presented here is not complete, however. The mechanisms a network changes in each step of process deserve a refinement. We refer to about the treatment of individuals' incentives to change in each resulting network up to reaching the stable one.
The study of the stability concept treated here and the efficient networks that is gave up is another topic for the further research together with applications to real cases. It will be interesting to know what happens if players participate in games at networks. Moreover, we would like to know how the equilibriums, if there is one, changes when the players are both myopic and forward looking one.
References
1. CHWE, Michael Suk-Young, 1994, "Farsighted Coalitional Stability," Journal of Economic Theory, No.63, pp 299-325. [ Links ]
2. DUTTA, Bhaskar and JACKSON, Metthew, 2001, "On The Formation of Networks and Groups". Forthcoming in the Strategic Formation of Networks and Groups, Draft, june 2001. Heidelberg: Springer-Verlag. [ Links ]
3. JACKSON, Matthew and WATTS, Alison, 1998, "The Evolution of Social and Economic Networks", Journal of Economic Theory, Vol. 106, No. 2, pp. 265-295. [ Links ]
4. JACKSON, Matthew and Wolinsky, Asher, 1996, "A Strategic Model of Social and Economic Networks," Journal of Economic Theory, No.71, pp.44-74. [ Links ]
5. PAGE, Frank and KAMAT, Samir, 2004, "Farsighted Stability in Network Formation. Forthcoming", in Group Formation in Economics: Networks, Clubs and Coalitions. Edited by: Wooders, Myrna and Demage, Gabrielle, 2004. [ Links ]
6. WATTS, Alison, 2001, "A Dynamic Model of Network Formation", Games and Economic Behavior, No.34, pp 331-341. [ Links ]
Primera versión recibida en julio de 2003; versión final aceptada en septiembre de 2004 (eds.)
Appendix
PROOF OF PROPOSITION 1. This proof is based on Tarski (1955) and Chwe (1994).
Define a network function set f : 2Z  2Z, where:
2Z, where:
f(X) = {g''  Z : V g', S/ g''
Z : V g', S/ g''  g;,
g;, 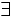 g
g  X...g' = gvg'
X...g' = gvg' 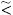 g, / g'' <s g}
g, / g'' <s g}
A set Y is consistent if and only if f(Y) = Y.
Note that if XCY  f(X)
f(X) 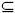 f(Y).
f(Y).
Let Σ = {XCZ : XCf(X)} with Σ ≠ φ.
Let Y = UX  ΣX.
ΣX.
Since f(X) 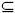 f(Y) VX
f(Y) VX  Σ, Y = UX
Σ, Y = UX  Σf(X)Uf(Y).
Σf(X)Uf(Y).
This means that f(y)Cf(f(Y)). Hence f(Y)  Σ, thus f(Y)CY.
Σ, thus f(Y)CY.
Therefore f(Y) = Y
PROOF OF PROPOSITION 3. This proof is based on Chwe (1994).
Consider Ω a stable set of (Z,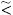 ). By proposition 1, it is sufficient to show that ΩCf(Ω). Consider a network gk such that gk
). By proposition 1, it is sufficient to show that ΩCf(Ω). Consider a network gk such that gk  Ω and gk
Ω and gk 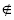 f(Ω). Then exist gl, S where gk
f(Ω). Then exist gl, S where gk  S gl such that Vgm
S gl such that Vgm  Ω gl = gmvgl
Ω gl = gmvgl 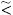 gm, gk <S gm. So gl
gm, gk <S gm. So gl  Ω
Ω  gk
gk 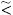 gl and Vgm
gl and Vgm  Ω such that gk
Ω such that gk 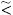 gm, gl
gm, gl 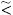 gm. Say gl
gm. Say gl  Ω. Then gk
Ω. Then gk 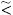 gl violating internal stability. So let gl
gl violating internal stability. So let gl  Z \ Ω. From external stability
Z \ Ω. From external stability 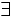 gm
gm  Ω, such that gl
Ω, such that gl 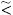 gm. But then gk
gm. But then gk 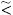 gm. Hence gk
gm. Hence gk 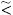 gm, violating inner stability.
gm, violating inner stability.
Notes
1. Dutta and Jacson (2002) said that steady states or cycles in network formation may emerge in this context.
2. For example, in the context of sequential subgame perfect Nash equilibrium this assumption is often used.
3. For a wide discussion about SIP see Watts (2001), Jackson and Watts (2001) and Dutta and Jackson (2002)
4. For example, under the connection model consider N=6, and suppose tha N=N1+N2, with N1=3. Suppose that N1 players form pairs of stars equals with center in any player belonging to coalitions. Then, from a current network star, two different coalitions are able to get away from.
5. Coalition S1 moves from g' to g to prevent coalition S2 from moving from g' to g.
6. Chwe has expressed that "[...]Von Newmann and Morgenstern argue that sets of (Z, <), where < is the direct dominance relation, are solution of a game, when the process is carry out as a game".
7. In voting situation there is a famous example, know as the Condorcet Paradox.














