Introducción
Fertility decisions have long been studied, specially in the field known as family economics. Becker (1960) develops an economic framework to analyze the factors that may potentially influence fertility: income, children costs, education and uncertainty. In the case of income, this author finds evidence that its increase boosts the demand for children, not only their quantity but also their quality (see Becker & Lewis, 1973, for an analysis making the distinction between quantity and quality of children).
Global reduction in fertility rates is a fact,2 as well as the increase in global real gross domestic product (GDP) per capita. Beyond this inverse relationship between income and fertility at a global level, it is interesting to analyze how households modify their decisions on birth spacing, defined as the length of time between one birth and the next birth, when economic conditions change. In this line, the purpose of this paper is to provide new evidence on Colombian households’ reactions regarding the timing of births when they face external (general and idiosyncratic) shocks. Unemployment rates and variations in GDP growth are considered as external shocks modifying the household’s budget constraints and, therefore, its decisions (McKenzie, 2003; Billingsley, 2011; Testa & Basten, 2012). We analyze the influence of changes in certain economic variables on the probability of women having a first, second, and third child. An increase in the probability of occurrence is equivalent to a reduction in birth spacing.
We use the Demographic and Health Survey of Colombia (DHS, wave 2010) carried out by Profamilia.3 This survey includes information regarding general characteristics of women from 15 to 49 years as well as the households where they live. By constructing the fertility history of each woman included in the survey, we estimate the effect of changes in per capita GDP growth and unemployment on birth timing. Also, we are interested in analyzing the role played by the age at first birth and the gender of previous children in the timing of future births. For these purposes, a Cox proportional hazards model is used, allowing us to analyze changes in the probability of occurrence of a birth within a given time period.
The DHS is carried out every five years and there are waves available for years before 2010; however, we restricted our database to the 2010 wave because historical information available regarding our variables of interest (GDP and unemployment) is limited.
In contrast to Adsera and Menéndez (2011), who analyze how labor market and economic shocks modify fertility decisions in seven Latin American countries, including Colombia, our contribution to the literature is precisely what makes this paper different from theirs: we present separate evidence for Colombia, while they only show aggregate results for Latin America. We consider this a relevant contribution to the empirical literature on the topic in Colombia. Moreover, we employ regional data on per capita GDP in order to check whether our results hold when considering heterogeneity of growth among regions. Regardless of the results of this exercise, we consider valuable to test the hypothesis according to which regional economic variations might be more important in fertility decisions than national ones; in any case, individuals might be internalizing differently regional and national economic conditions.
It is worth clarifying that our results, as well as those from previous papers on the topic, should not be interpreted as causal effects. They represent associations between fertility decisions regarding birth spacing and variations in economic conditions.
The paper is divided as follows. Section I shows the literature review. Section II presents the empirical strategy adopted in the paper. Next, we present a brief section with some relevant statistics mainly based on the DHS. Section IV presents the results of the Cox proportional hazards model. Finally, the last sction shows some concluding remarks.
I. Theoretical background and literature review
The analysis of the demand for children allows us to understand some of the complexities underlying household choices. Several studies suggest that the determinants of the demand for children are income per capita, the price of children and parents’ preferences for children relative to other goods and services that provide utility to the couple. The relative importance of these factors might differ according to the price and income elasticity of each couple’s demand.
Other things equal, higher income is expected to be associated with greater demand for children; however, this increment could be materialized through quality of children instead of quantity. That is, higher income is associated with higher opportunity cost to leave the labor market; thus, parents prefer to provide better services to their already born children than having additional children (substitution effect). Nonetheless, it is also true that higher income may allow parents to afford higher expenses from greater family size (income effect). Thus, if the income effect dominates the substitution effect, birth spacing will decrease and vice versa. In general, we can expect that the occurrence of an external shock may positively affect fertility choices (i.e., demand for children) when the marginal benefit provided by the shock is greater than the cost (in present value) of increasing the number of children.
As Boongarts (1978) pointed out, the demand for children comes from the existence of a transmission channel of behavioral factors over background external variables. That is, behavioral factors are proximate determinants that may be affected, but not always, by the presence of certain events such as socioeconomic shocks. These proximate determinants include actions like use of contraception methods. Davis and Blake (1956) previously studied these determinants calling them “ intermediate fertility variables” .
Along these lines, Easterlin (1975) highlights the necessity of a microeconomic comprehensive framework to understand human fertility behavior. In this regard, the demand for children is affected by income, the relative price of children and subjective preferences for children relative to private goods. Then, external income shocks could modify preferences toward children.
Several papers have empirically analyzed this topic using different approaches and estimation techniques. These papers have also attempted to determine whether fertility varies with economic conditions in a pro-, counter- or acyclical manner. Macrodata-based studies address the relationship between economic conditions and overall fertility, while those based on microdata are closer to our purposes since they also examine birth spacing.
Empirically, Kirk (1960) analyzes the influence of the business cycle on births in the United States using the degree of covariation of annual deviations from trend fertility rates and economic indicators such as the index of industrial production. According to his results, the economic situation explains about one half of the variance of fertility; however, this is not meant as a signal of causal relationship but as evidence on the influence that the economic situation has on fertility patterns. In line with this approach, Mocan (1990) employs time series techniques with aggregate data from the USA to explore the relation between the business cycle and fertility decisions. The author uses vector autoregressions to find that fertility moves countercyclically over the business cycle, implying, as mentioned before, that the substitution effect of a decrease in the opportunity cost associated with bad economic conditions dominates the effect of lower income. These findings are similar to those in Butz and Ward (1979) for the early 1970s and in Wang, Yip and Scotese (1994) for the postwar United States. In the latter paper, the authors estimate a fertility theoretical model through a structural vector autoregression and find a short-run negative response of fertility to an employment shock and a weak effect of output disturbances on fertility.
Unlike the previous evidence on countercyclical fertility, there is also evidence of a procyclical tendency of fertility. Macunovich (1995, 1996) suggests that the results found by Butz and Ward (1979) do not hold when different data are used; furthermore, even with the original data, the model does not fit well in the period after 1954. More specifically, the author claims that fertility remained procyclical due to the sizable negative effects of unemployment relative to the lower costs associated with children in a recession period. In the same line, Sobotka, Skirbekk and Philipov (2011) find that, for most developed countries, the great recession brought a decline in the number of births and fertility rates, suggesting these two variables are procyclical.
These previous studies employ macrodata to evaluate how economic conditions influence fertility trends. Nevertheless, there is consensus around the idea that, due to multiple and sometimes opposing effects, the impact of the economic environment on fertility decisions may differ across individuals according to their gender, age, social group, wealth and other demographic factors. In other words, analyzing data at the aggregate level only shows the demographic responses of large populations. As a result, new techniques are necessary to get more reliable estimations on the influence of the business cycle on fertility decisions. For instance, a different method that combines longitudinal micro data with macroeconomic variables within an event history framework has been developed. This statistical technique allows the researcher to distinguish between changes in the fertility level and changes in fertility timing (birth spacing).
In fact, there is a variety of studies employing survival analysis to measure birth timing and its relation with macroeconomic data. For example, Kertzer et al. (2008) use data from the Italian Longitudinal Household Survey and, through a Cox model, find that women’s employment has a strong negative effect on the transition from first to second birth. In other study, Gutiérrez-Domènech (2008) shows evidence of employed Spanish married women postponing their first childbearing because of the greater opportunity cost. This also applies for the second child but not for the third, suggesting that demographic factors (education and region) rather than employment explain this last fertility decision. Along these lines, Meron and Widmer (2002) analyze the French case for the period 1952-1973 and find that unemployment induces a delay in maternity for women living in a couple. Moreover, Karatepe (2009) finds a negative relationship between economic conditions and marital fertility decisions of Turkish women.
As previously discussed, there is no conclusive evidence on the relationship between unemployment (or GDP) and fertility decisions. This is especially clear in the German case presented by Özcan, Mayer and Luedicke (2010), who find that being unemployed is positively associated with the transition to motherhood in East Germany, whereas a significant effect cannot be shown in West Germany. These results could be related to the fact that lower wages in the east region reduce the relative weight of the income effect of unemployment.
Finally, evidence on this issue for Colombia and Latin America is scarce, which in part motivates this paper. Adsera and Menéndez (2011) study the relationship between fertility choices and the business cycle using two different approaches. First, they employ macrodata to obtain the response of total fertility to economic shocks (unemployment and GDP growth) in 18 countries. Second, they use microdata to estimate a Cox proportional hazards model in order to analyze the timing of births in seven countries. According to their results, there exists a procyclical relationship: bad economic conditions (high unemployment and slowdowns in GDP per capita growth) lead to longer birth spacing. They also find that changes in employment are more important in this procyclical relationship than changes in economic growth. This study is the closest to our research; the difference is that their results correspond to Latin America, while we analyze the case of Colombia by considering regional heterogeneity in economic conditions.
II. Empirical strategy
We want to determine the association between external shocks, such as changes in economic conditions, and women’s fertility decisions related to the spacing of births. For this purpose, we need a methodology that allows us to model changes in the probability of occurrence of a birth within a given time period. Survival analysis is precisely concerned with exploring the time to the occurrence of an uncertain event, where, in this case, the uncertainty stems from both biological reasons and the behavioral character of the childbearing decisions. Under this approach, the researcher can use parametric models (Retherford et al. 2010), semi-parametric analysis (Adsera and Menéndez, 2011) or non-parametric analysis. A drawback of parametric modeling is the need to assume a normal distribution of the residuals, which is not reasonable in many cases of survival analysis. As for non-parametric models, Cleves et al. (2008) point out that they do not appropriately deal with censoring and other issues related to survival data.
We use here the Cox proportional hazards regression, a semi-parametric continuos-time model that better suits our purposes. Let us define the hazard rate for an individual  as
as
Recall that the hazard rate is the probability that the failure event (i.e., a birth) occurs in a given time interval. h0(t) is called the baseline hazard, X corresponds to a vector of characteristics of individual i, and β is a vector of regression coefficients. One advantage of this methodology comes from the fact that it is not necessary to give a functional form to the baseline hazard, which is not even estimated. The model is semi-parametric because the baseline hazard function is not parameterized, but the effects of other covariates are. Also, there are no assumptions about the shape of the hazard; instead, the hazard is supposed to be the same for every individual in the data: one individual’s hazard is a multiplicative replica of another individual’s hazard.
As Newman and McCulloch (1984) emphasize, the essential feature of the hazard rate approach is “to shift the focus of the problem from one where factors directly affect the occurrence of an event to one where they influence the risk of occurrence” (p. 939). In our case, the hazard estimates the probability that a birth occurs for woman i in each time period t, conditional upon the birth not occurring in a previous time period,
Where Ti is the time period in which the birth occurs (when woman i gives birth); if there is no birth, Ti corresponds to the year of the survey. We formulate a separate hazard function for the first, the second, and the third birth. This separating approach helps to solve the potential problem of risk periods and outcomes for each woman being correlated over time. Thus, we are comparing outcomes across women of equal parity or at each risk period (from no children to the first child, from the first to the second child, and from the second to the third child). Using the DHS, we construct the individual fertility history of each woman in the sample, considering her date of birth and the birth dates of her children.
Survival analysis requires defining two fundamental variables: duration and occurrence of the event. In our case, the duration variable is defined as the difference in months between the birth date of the nth child (T) and the birth date of the nth-1 child ( t0 ). For the first child, we use two specifications to calculate duration: first, the difference in months between the date of the first childbearing (T) and the date at which the woman becomes 12 years old ( t0 ), which corresponds to the average age of menarche (Conde et al., 2003); second, we use as an alternative t0 the date at which the woman experiences her first intercourse. The reason to make two different specifications of t0 is that we are aware of the difficulty of accurately determining the first birth interval; thus, having two options is a type of robustness test of the results.
For the second child, duration is the difference in months between the date of the second childbearing (T) and the date of the first childbearing (
t0
). For the third child,
t0
would be the date of birth of the second child and T the date of birth of the third child. If the birth of the 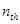 child does not occur, the time period is set between the date of birth of the
nth-1
child and the date of the survey, unless the woman had a sterilization procedure; in this case, T is the date of the sterilization.
child does not occur, the time period is set between the date of birth of the
nth-1
child and the date of the survey, unless the woman had a sterilization procedure; in this case, T is the date of the sterilization.
Duration is the dependent variable in all the estimated models. As for the occurrence variable, it takes the value of 1 if the event (i.e., birth) occurs in a given time period (from no children to the first child, from the first to the second, and from the second to the third birth), and 0 otherwise.
The original database does not include the duration and occurrence variables; therefore, it is necessary to construct them. In the model, the response variable is the triplet ( t0 , T, d), which denotes the time span from t0 to T with censoring indicator d (i.e., occurrence). Hence, the hazard ratios estimated for each explanatory variable through the Cox proportional model can be interpreted as the variation in the probability of occurrence as well as the variation in the spacing of birth.
In order to capture the effect of economic conditions on birth spacing, we use two specifications of GDP per capita growth rate (one- and two- period lags): national and regional. Moreover, we estimate another model using the unemployment rate (one- and two-period lags) because this variable might be more appropriate than GDP to capture the economic conditions of the family, since it is through access to job positions that most individuals get to benefit from GDP growth. In fact, Adsera and Menéndez (2011) find that women seem to postpone and reduce childbearing because of economic downturns, a behavior that is mainly associated with increases in the unemployment rate rather than decreases in GDP.
Another economic variable included in the estimations is a dummy variable that equals 1 for 1999 and thereafter. The purpose of including this variable is to take into account the strong economic crisis that took place in Colombia that year.
In addition, with the purpose of capturing potential gender preferences of parents, we are especially interested in exploring the relation of the timing of births with the gender of previous children. Furthermore, the effect of variations in economic conditions on fertility decisions may differ according to the educational attainment level of the woman and her spouse, the region in which she lives, her age, her location (rural or urban) and her age at first childbearing. We control for all these variables.
III. Data and descriptive statistics
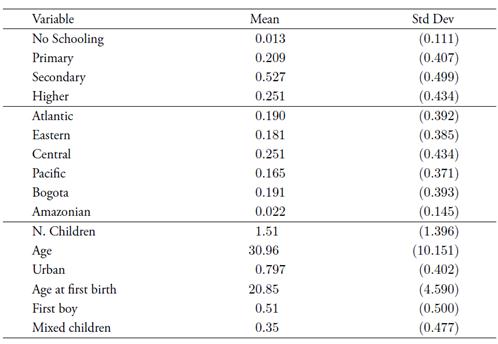
The 2010 DHS contains information on the socioeconomic conditions of households with women from 15 to 49 years old. The survey inquires on family planning methods, intrafamily violence, mortality and morbidity of children and women, incidence of sexually transmitted diseases, among other factors. The structure of the data allows researchers to figure out the complete reproductive history of each woman, which is the main input for our approach. The sample has 41,827 women. Differences in the sample size of the estimated models come from differences in the quantity of eligible women according to their number of children. In this section, we offer a description and summary statistics of the data used as well as some information on actual birth spacing and economic performance in Colombia.
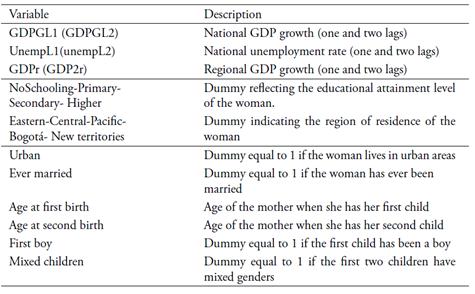
Table 1 presents a brief description of the variables included in the estimations, and Table 2 includes some summary statistics of the control variables. In addition to our variables of interest (i.e., those related to economic conditions and the gender of previous children), we control for the educational attainment level of the woman, region of residence, urban or rural location, marital status, and woman’s age at first (or second) birth. Due to data restrictions, we use the data on education and place of residence at the time of the survey instead of those corresponding to the birth of each child.
Table 2 Average birth spacing and average age of women at first, second and third birth
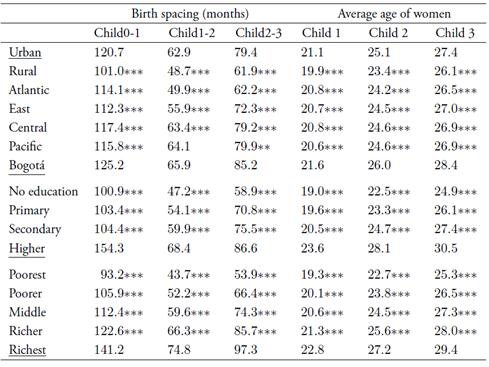
Notes: the first three columns refer to the spacing in months between births: 0 children to 1 child, 1 to 2, and 2 to 3, respectively. The first column takes as t0 the date at which each woman becomes 12 years old. The last three columns refer to the average age of women at first, second and third birth, respectively.
*** Statistically different (1%) from the average of reference -underlined category-.
Source: own calculations based on DHS.
Table 3 shows the average birth spacing from no children to the first childbearing, from the first child to the second, and from the second child to the third (three first columns). The last three columns present the average age of women when giving birth to their first, second, and third child.
It is observed that there is greater distance between the third and the second child compared to the spacing of births between the second and the first child. Also, spacing is higher for women located in urban areas, and these women’s average age at childbearing is higher in all three cases as well. Comparing different regions of the country, statistics show that birth spacing and average age at childbearing are the highest for women living in the capital city. Finally, as expected, more educated women and those belonging to the richest groups have both wider spacing of births and higher average age when bearing their first, second, and third child.
In addition, Table 4 shows that average birth spacing is much higher than median birth spacing, thus suggesting a strong positive bias of the birth spacing distribution.
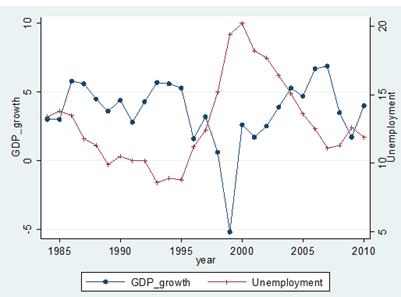
As for economic performance in Colombia, Figure 1 shows the evolution of the growth rate of GDP and the unemployment rate. It is observed that the 2000s was characterized by relatively good economic performance at least until 2008. Other Latin American countries displayed the same pattern during that decade. The impact of the global crisis experienced on 2008 reduced the GDP growth rate but it remained positive. In contrast, just before 2000, Colombia experienced the biggest economic downturn since the Great Depression due to a banking crisis that affected the labor market and households’ purchasing power.
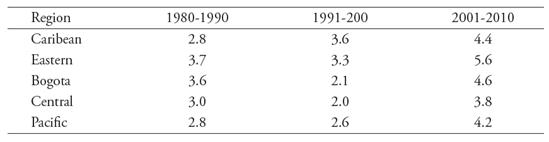
Finally, given our interest in analyzing the association between regional economic conditions and birth spacing, it is relevant to check differences in growth across regions in Colombia. In this respect, Table 5 shows the growth rates in the Colombian regions in the last three decades. Growth has increased during this century in all the regions. The lowest growth rate during the late 1990s was due to the sizable economic crisis in 1999, which motivates inclusion of the dummy variable mentioned above in the estimated models. Moreover, the Eastern region had the highest growth rate in the last decade thanks to the mining sector. The visible differences in regional economic performance motivate model estimation using regional GDP growth rates.
IV. Results
Our main results are summarized in Tables 6 to 8.4 These have four columns referring to different specifications of the dependent variable: the first and second columns correspond to the birth spacing from no children to the first childbearing using two alternative  as explained before. The third column refers to the birth spacing between the first and the second child, and the fourth column presents spacing between the second and the third child.
as explained before. The third column refers to the birth spacing between the first and the second child, and the fourth column presents spacing between the second and the third child.
Estimations correspond to the whole sample of women from 15 to 49 years. We could have made the estimations by cohorts considering that the influence of the controls might differ across the various age groups; however, instead of separating cohorts, we stratify the model using the age group since this variable does not meet the proportionality hypothesis, an assumption of the Cox proportional model.5
When using the growth rate of GDP-one- and two-period lags, GDPGL1 and GDPGL2- as the variable reflecting economic conditions (Table 6), the results suggest that better economic performance is associated with a higher probability of having an additional child, this is, it reduces birth spacing. Thus, it seems that demand for children could be procyclical, predominating the income effect. Note, nonetheless, that the sign of the second lag is the opposite. This result can have two interpretations: first, good economic conditions initially increase the demand for children; however, if these good conditions persist, the demand for children decreases, suggesting the dominance of a substitution effect. An alternative interpretation is simply that economic growth increases the probability of having children, automatically reducing the risk after the boom.6
Table 6 Hazard ratios from Cox proportional hazards model (GDP growth)
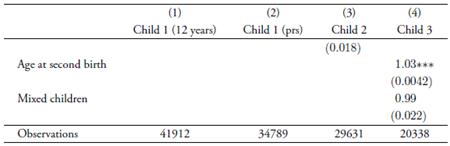

Notes: Exponentiated coefficients; Standard errors in parentheses.
Source: own calculations based on DHS.
These results are similar when using the unemployment rate-one- and two-period lags, UnemL1 and UnemL2-instead of the growth rate of GDP (Table 7). When the unemployment rate is high (low), households’ economic situation worsens (improves) and the probability of having an additional child falls (increases). Interpretation of the second lag is analogous to the previous explanation offered with respect to GDP growth. It is important to note that unemployment has a stronger effect on households’ decisions regarding birth spacing than economic growth. These results are in line with those obtained by Adsera and Menéndez (2011). As we mentioned before, the unemployment rate might be more appropriate to reflect household economic conditions than GDP, since most individuals benefit from economic booms through access to job positions.
Table 7 Hazard ratios from Cox proportional hazards model (unemployment rate)
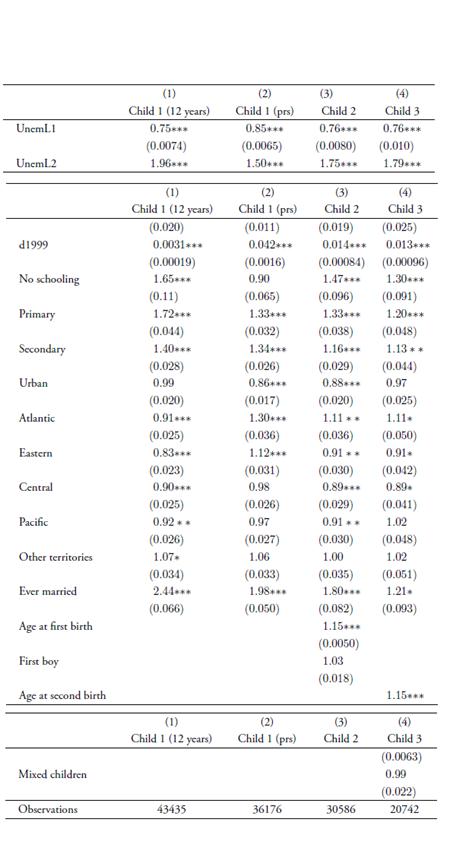
Notes: Exponentiated coefficients; Standard errors in parentheses.
Source: own calculations based on DHS.
Table 8 employs regional GDP growth rates (GDPGRL1 and GDPGRL2) as indicators of economic conditions. We observe that the effect goes in the same direction as national GDP, but it is much smaller. This is an interesting result since a stronger association between birth spacing and regional economic conditions was actually expected, compared to national conditions. Nevertheless, it is true that individuals are more exposed to national indicators (through the newspapers or TV news) than to regional information, and this actually shapes their expectations and decisions.
Table 8 Hazard ratios from Cox proportional hazards model (regional GDP)
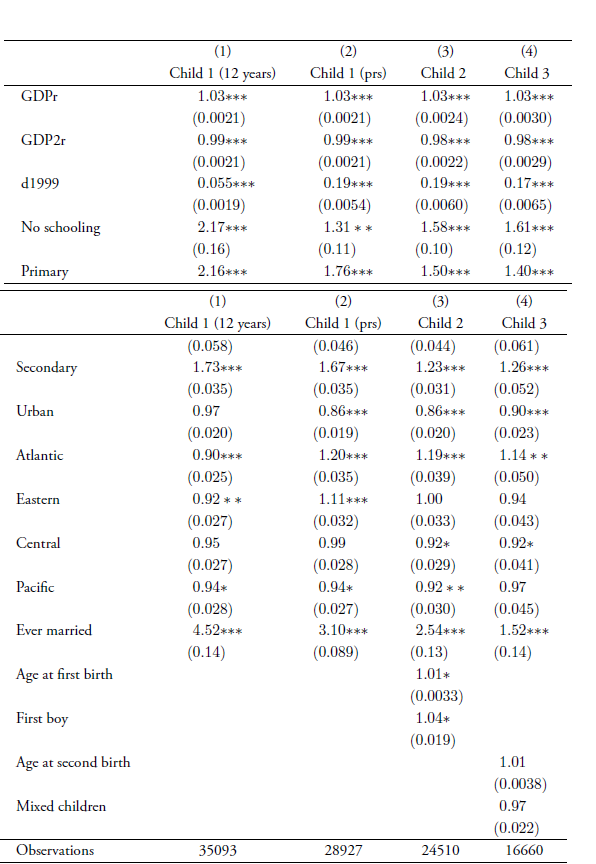
Notes: Exponentiated coefficients; Standard errors in parentheses. * p< 0.5, ** p< 0.01, *** p<0.001
Source: own calculations based on DHS.
As for the dummy variable for year 1999 onwards, the results show that the birth hazard decreases after this year, that is, birth spacing increases after the year in which Colombia was hardest hit by the worst economic crisis of the last decades.
Turning to the importance of gender preferences, our results suggest that having a boy as a first child has a small positive relation with the probability of giving birth to a second child; however, the association seems to be weak since it is not significant when unemployment is used as an indicator of economic conditions. Moreover, the results do not show a significant association between mixed-gender siblings and the probability of having a third child. These results are in line with those found by Angrist and Evans (1998), according to which the impact of the gender of the firstborn is very small and the probability of having a third child is lower for those women having mixed-gender children than for those having children of the same gender-although this last hazard ratio is not significant in our study, the sign is the same.
Let us now refer to the control variables. The importance of education in these hazard models is crucial. As expected, having lower educational background increases the probability of bearing a child. That is, birth spacing increases with schooling (Tables 3 to 5). This relationship reflects the higher opportunity cost of leaving the labor market when agents have accumulated high human capital levels. Again, these results are in line with those from previous studies.
Another interesting finding comes from the importance of age at first and second bearing (Tables 3 to 5, columns 3 and 4): our results suggest that the older the woman is when she becomes a mother (for the first and second time), the shorter the birth spacing. This is as expected since older women face higher risks associated with childbearing, and therefore they have less time to space births if they want to have more than one child.
As for the place of residence, the hazard ratio is higher for women living in rural areas. This might be due to the lower schooling and labor opportunities of women living in the countryside, which considerably reduces their opportunity cost of having children. Moreover, children in those areas are considered as future labor force for land work as well as an insurance for their parents’ old age.
Finally, as expected, having ever been married strongly increases the birth hazard.
Conclusions
This paper provides empirical evidence for Colombia on the association between economic conditions and household fertility decisions related to birth spacing. Our empirical strategy involves the use of the Cox proportional hazards approach in order to model changes in the probability of occurrence of a birth and the birth spacing of the first, second and third child, taking into account other factors such as socio-demographic conditions and gender preferences. Although this document does not deal with the causal effect methodology, our results suggest that economic conditions might be crucial for the family choices in terms of timing of births.
According to our findings, better economic performance (i.e., lower unemployment rates and higher GDP growth) is associated with a higher probability of having an additional child, that is, it reduces birth spacing. Thus, it seems that the demand for children could be procyclical, predominating an income effect; however, when good economic conditions persist, the demand for children decreases (the sign of the second lag is contrary to the sign of the first lag). This could be interpreted either as a natural decrease in the demand for children after booms or as a change in the dominant effect (towards a substitution one). The results are similar when considering regional heterogeneity in economic performance (although the hazard ratio is smaller).
Regarding gender preferences, the results reveal a small association between the gender of the first child and the probability of having a second child. Specifically, when the first child is a boy, the probability of having a second child slightly increases. As for the decision of having a third child, it does not seem to be associated with the gender of previous children.
As mentioned above, our results suggest that the business cycle might be correlated with the fertility choices of the household regarding birth spacing. To be specific, households are taking a long-term decision-having children-partially influenced by short-term economic conditions. Therefore, it is important for the government to increase its capacity to smooth economic fluctuations and to provide more information to households to take their fertility decisions.
One interesting question to be solved, which is out of the scope of this paper because of data availability, consists of evaluating the impact of direct shocks such as unemployment of one of the household members on fertility choices, compared to the effect of aggregate shocks such as variations in GDP growth or the national unemployment rate. This is left for future research