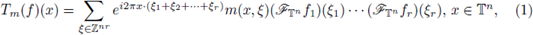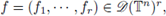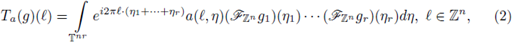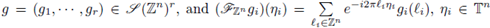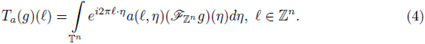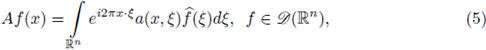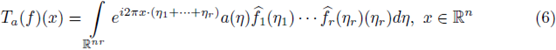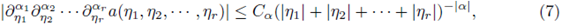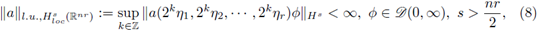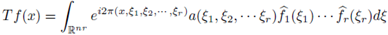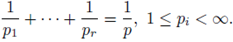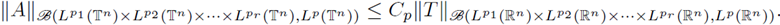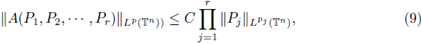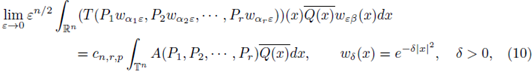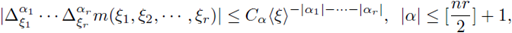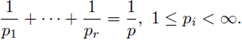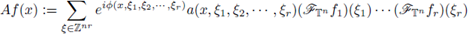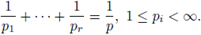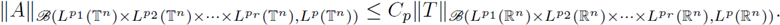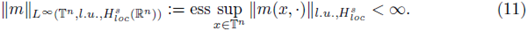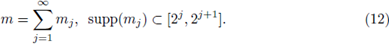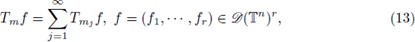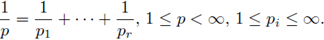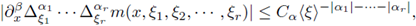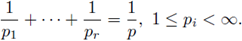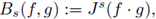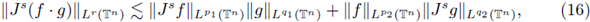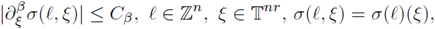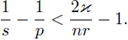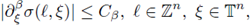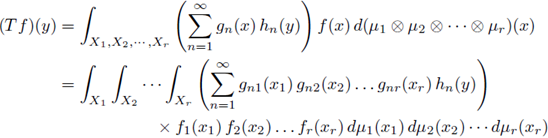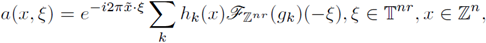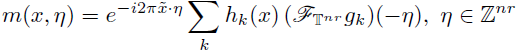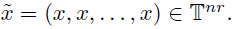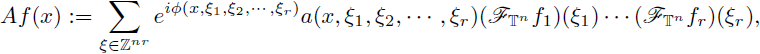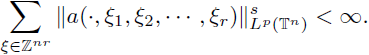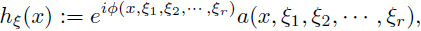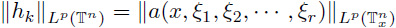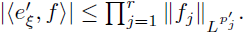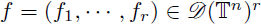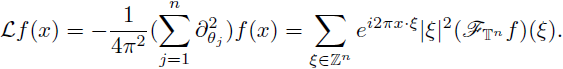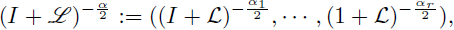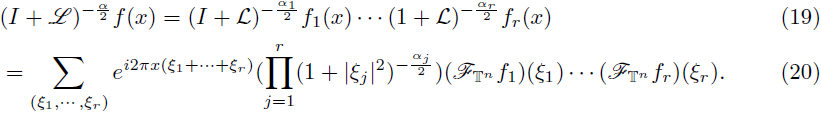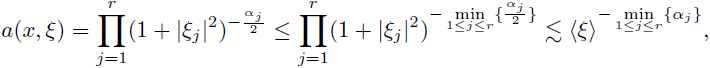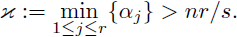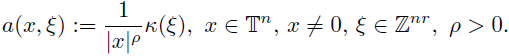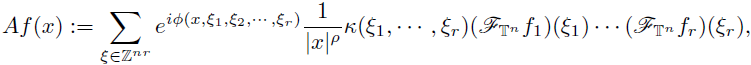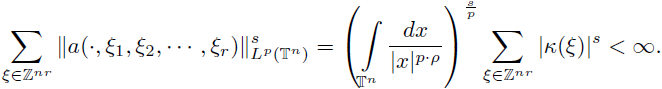1. Introduction

The goal of this note is to announce the main results about the L^p-multilinear analysis developed by the authors in ^[10] for periodic and discrete pseudo-differential operators. These operators can be defined by using the multilinear Fourier transform as follows. If 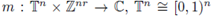is a suitable function, then the periodic multilinear-pseudo-differential operator associated to m is the operator defined as

where and

is the periodic multilinear Fourier transform of f. We have denoted by the space of smooth functions on the torus endowed with its usual Fréchet structure. On the other hand, if α: 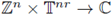is a measurable function, then the discrete multilinear-pseudo-differential operator associated to α is the multilinear operator defined by

where is

the discrete Fourier transform of g_i. For r ≥ 2, these operators have been studied by V. Catană in ^[12]. If r = 1, these quantization formulae can be reduced to the known expressions

and

Periodic pseudo-differential operators (see (3)) were defined by Volevich and Agranovich ^[1]. The subsequent works of McLean ^[29], Turunen and Vainikko ^[47], and Ruzhansky and Turunen ^[44] developed a periodic analysis from Hörmander classes to applications to periodic equations, although the symbolic calculus was consistently developed by Ruzhansky and Turunen ^[44]. Nevertheless, the references Ruzhansky and Turunen ^[44], ^[45], Cardona ^[6], ^[7], ^[8], Delgado ^[15] and Molahajloo and Wong ^[34], ^[35], ^[36] provide some complementary results for the subject. Mapping properties for more general operators as periodic Fourier integral operators appear in Ruzhansky and Turunen ^[45] and Cardona, Messiouene and Senoussaoui ^[11].

In a more recent approach, pseudo-differential operators on 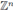(discrete pseudo-differential operators) were introduced by Molahajloo in ^[36], and some of its properties were developed in the last years in the references ^[9], ^[17], ^[28], ^[38], ^[39], ^[40], ^[41], ^[43]. However, only the fundamental work L. Botchway, G. Kibiti, and M. Ruzhansky ^[5] includes properties about a discrete pseudo-differential calculus and applications to difference equations. The reference ^[9] discusses those relations of the theory of discrete pseudo-differential operators with important problems in number theory as the Waring problem and the hypothesis K ^* by Hooley.

An overview to the mapping properties for pseudo-differential operators on 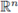provides the expected results in the discrete and periodic setting. On these operators have the form

with 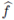the euclidean Fourier transform of f (see Hörmander ^[25]). The nuclearity of pseudo-differential operators on has been treated in Aoki and Rempala ^[2], ^[42]. In a context closely related to our work, multilinear pseudo-differential operators have been treated in Bényi, Maldonado, Naibo, and Torres, ^[3], ^[4], Michalowski, Rule and Staubach, Miyachi and Tomita ^[30], ^[31], ^[32], ^[33] and references therein. The multilinear analysis for multilinear Fourier multipliers

born with the works of Coifman and Meyer ^[13], ^[14], where the condition

for sufficiently many multi-indices α = (α₁,α₂, · · · , a_r), was proved to be sufficient for the boundedness of T_a from 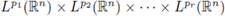into 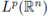provided that 1/p = 1/p₁ + 1/p₂ + · · · + 1/p_r, and 1 ≤ p_i, p < ∞. A generalization for this result was obtained by Tomita in ^[46], where it was proved that the multilinear Hörmander condition

implies the boundedness of T_a from 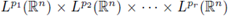into 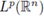provided that 1/p = 1/p₁ + 1/p₂ + · · · + 1/p_r, and 1 ≤ p_i, p < ∞. The case r = 1 is known as the Hörmander-Mihlin theorem. These multilinear theorems have been generalized to Hardy spaces 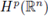for suitable values of 0 < p_i, p < ∞, in the works of Grafakos, Torres, Miyachi, Fujita, Tomita, Kenig, Stein, Muscalo, Thiele and Tao ^[19], ^[21], ^[22], ^[23], ^[24], ^[27], ^[37]. The main novelty of this work is that we provide discrete and periodic analogues for these works in the multilinear setting.

This note is organized as follows. In section 2 we provide those results on the boundedness of pseudo-differential operators on 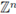and the torus. Later, in Section 3 we classify those s-nuclear multilinear integral operators on arbitrary σ-finite measure spaces and we apply this classification to periodic and discrete multilinear pseudo-differential operators.

2. Boundedness of pseudo-differential operators on 𝕋ⁿ and ℤⁿ

In this section we explain in detail the main results of our investigation on the bounded-ness of the multilinear operators considered. Our starting point is the following multilinear version of the Stein-Weiss multiplier theorem (see Theorem 3.8 of Stein and Weiss ^[48]). Sometimes we denote (x,ξ) := (x, ξ₁, · · · , ξ_r) = x · (ξ₁ + · · · + ξ_r).

Theorem 2.1. Let 1 <p < ∞ and let α: be a continuous bounded function. If the multilinear Fourier multiplier operator

extends to a bounded multilinear operator from 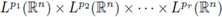into 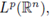then the periodic multilinear Fourier multiplier

also extends to a bounded multilinear operator from into 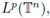provided that

Moreover, there exists a positive constant C _p such that the following inequality holds:

Remark 2.2. Theorem 2.1 can be proved in the following way. By the density of the trigonometric polynomials, we can prove that under the conditions of this theorem, we have the estimate

where the constant C does not dependent of every trigonometric polynomial P_i. For this, we will prove that

for some positive constant c_n,r,p > 0. We will assume that

Observe that by linearity, we only need to prove (10) when 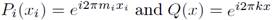for k and m_i in 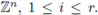The main step in our proof (see Cardona and Kumar ^[10]) is to show (10) and how it implies (9).

With the help of the previous result we prove the following fact. We use the notation

for all 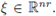Now, we provide the following discrete version of the known result of Coifman and Meyer mentioned in the introduction.

Theorem 2.3. Let T_m be a periodic multilinear Fourier multiplier. Under the condition

the operator T _m extends to a bounded multilinear operator from provided that

If we consider Fourier integral operators (FIOs) with periodic phases, we can recover the following multilinear version for FIOs of the multiplier theorem of Stein and Weiss.

Theorem 2.4. Let 1 < p < ∞ and let ф be a real valued continuous function defined on 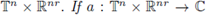is a continuous bounded function, and the multilinear Fourier integral operator

extends to a bounded multilinear operator from 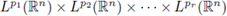into 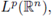then the periodic multilinear Fourier integral operator

also extends to a bounded multilinear operator from 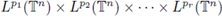into 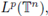provided that

Moreover, there exists a positive constant C_P such that

Now, we present some results about the boundedness of periodic multilinear pseudodifferential operators where explicit conditions on the multilinear symbols are considered.

Theorem 2.5. Let us assume that m satisfies the Hörmander condition of order s > 0,

Then the multilinear periodic pseudo-differential operator T_m associated with m extends to a bounded operator from 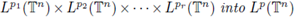provided that 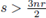and

Remark 2.6. The proof of Theorem 2.5 is based on a suitable Littlewood-Paley decomposition of the symbol m. Indeed, we decompose m as

We prove that by assuming (11), we can decompose the operator T_m as

where every operator T _mj is associated to the symbol m _j, and we prove that the operator norm of every T_mj is less than 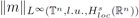multiplied by a factor proportional to 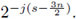We conclude our proof in ^[10] by observing that

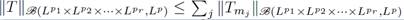
The following theorem is an extension of the Coifman-Meyer result presented above in the multilinear pseudo-differential framework.

Theorem 2.7. Let us assume that m satisfies the discrete symbol inequalities

sup |A|₁ ¹ A|² ··· A|; m(xfe, ··· ,ξr )| < C_afe-H (14)

for all 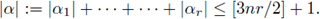Then the periodic multilinear pseudo-differential operator T_m extends to a bounded operator from 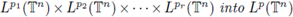provided that

Remark 2.8. We prove Theorem 2.7 by observing that (14) implies (11). We develop this delicate argument in ^[10] where we use, among other things, the periodic analysis developed by Ruzhansky and Turunen.

The condition on the number of discrete derivatives in the preceding result can be relaxed if we assume regularity in x. We show it in the following theorem.

Theorem 2.9. Let T_m be aperiodic multilinear pseudo-differential operator. If m satisfies toroidal conditions of the type

where 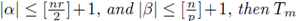extends to a bounded multilinear operator from 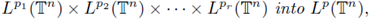provided that

Example 2.10. Theorem 2.7 applied to the bilinear operator

where J^s is the periodic fractional derivative operator 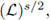or the periodic Bessel potential of order 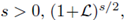implies the (well known) periodic Kato-Ponce inequality:

where 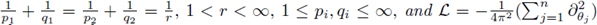is the Laplacian on the torus.

Boundedness of discrete multilinear pseudo-differential operators. Our main results about the boundedness of discrete multilinear pseudo-differential operators are stated as follows.

Theorem 2.11. Let 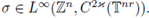If σ satisfies the discrete inequality

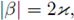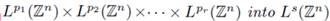for all β with then T_σ extends to a bounded operator from provided that 1 ≤ pj ≤ p ≤ ∞, and

The following result can be derived of the previous result with r = 1 and s = p.

Corollary 2.12. Let If σ satisfies the discrete inequality

for all β with then T_σ extends to a bounded operator from 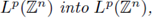provided that

3. s-Nuclearity, 0 < s ≤ 1, of pseudo-differential operators on 𝕋ⁿ and ℤⁿ

In this section we study the s-nuclearity, 0 < s ≤ 1 of multilinear discrete and periodic pseudo-differential operators. We prove Theorem 3.1 regarding the characterization of s-nuclear multilinear operators on abstract σ-finite measure spaces, and Theorem 3.2 and Theorem 3.3 regarding the characterization of s-nuclearity of periodic and discrete pseudo-differential operators. Although these theorems are multilinear extensions of the results due to Delgado ^[16], Delgado and Wong ^[17], JamalpourBirgani ^[26] and Ghaemi, JamalpourBirgani and Wong ^[20], we can recover their results from our results by considering r = 1. In order to study these multilinear operators admitting s-nuclear extensions, we prove the following multilinear version of a result by Delgado, on the nuclearity of integral operators on Lebesgue spaces (see ^[16], ^[18]). So, in the following multilinear theorem we characterize those s-nuclear (multilinear) integral operators on arbitrary (σ-finite) measure spaces (X,μ).

Theorem 3.1. Let (Χ_ί,μ_ί), 1 ≤ i ≤ r and (Y, ν) be σ-finite measure spaces. Let 1 ≤ P _i ,ρ < ∞, 1 ≤ i ≤ r and let 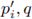be such that for 1 ≤ i ≤ r. Let 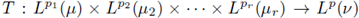be a multilinear operator. Then T is a s-nuclear, 0 < s ≤ 1, operator if, and only if, there exist sequences {g_n}_n with g_n = (g_n1,g_n2,...,g_nr) and 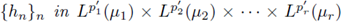and L^P(v), respectively, such that 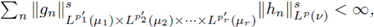and for all f = (f₁, f₂,...,f_r) ∈ L^P1 (μ) χ L^P2 (μ2) χ ··· χ L^Pr (μr ) we have

for almost every y ∈ Y.

Remark 3.2. The proof of Theorem 3.1 is based on an important lemma proved in [10, Lemma 4.1]. The proof of the if part of Theorem 3.1 follows using the definition of nuclear operators, Lemma 4.1 (iv) of ^[10] and the fact that L^p-convergence of a sequence implies the convergence of a sequence almost everywhere.

The only if part of Theorem 3.1 is a straightforward using the part (iv) of [10, Lemma 4.1] and applications of monotone convergence theorem of B. Levi and Lebesgue dominated convergence theorem.

This criterion applied to discrete and periodic operators gives the following characterizations (for the proof we refer the reader to ^[10]).

Theorem 3.3. Let a be a measurable function defined on . The multilinear pseudo-differential operator 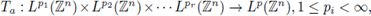for all 1 ≤ i ≤ r, is a s -nuclear, 0 < s ≤ 1, operator if, and only if, the following decomposition holds:

where 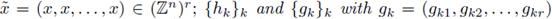are two sequences in 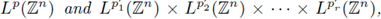respectively, such that

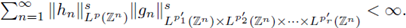
Similarly, we can classify the s-nuclearity of periodic multilinear operators.

Theorem 3.4. Let m be a measurable function on 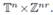Then the mutlilinear pseudo-differential operator 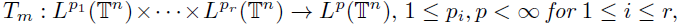is a s -nuclear, 0 < s ≤ 1, operator if, and only if, there exist two sequences {g_k}_k with 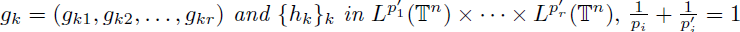for 1 ≤ i ≤ r and 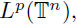respectively, such that 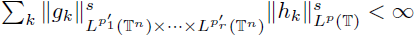and

where

Now, we present the following sharp result on the s-nuclearity of periodic Fourier integral operators.

Theorem 3.5. Let us consider the real-valued function 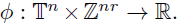Let us consider the Fourier integral operator

with symbol satisfying the summability condition

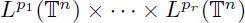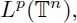Then A extends to a s-nuclear, 0 < s ≤ 1, operator from into provided that 1 ≤ p _j < ∞, and 1 ≤ p ≤ ∞.

Remark 3.6. The proof of Theorem 3.5 follows using Theorem 3.1 by considering the function

the functional

and their estimates

and

Example 3.7. In order to illustrate the previous conditions, we consider the multilinear Bessel potential. This can be introduced as follows. Consider the periodic multilinear Laplacian denoted by

acting on by

For r = 1, we recover the usual periodic Laplacian

The multilinear Bessel potential of order

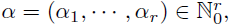
can be defined by the Fourier analysis associated to the torus as

From the estimate

Theorem 3.5 applied to 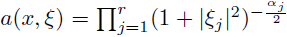implies that the multilinear Bessel potential 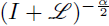extends to a s-nuclear operator from 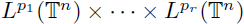into 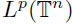for all 1 ≤ pj < ∞ and 1 ≤ p ≤ ∞, provided that

This conclusion is sharp, in the sense that if we restrict our analysis to r = 1 and p₁ = ρ = 2, the operator 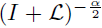extends to a s-nuclear operator on 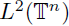if, and only if,

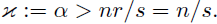
Example 3.8. Now, we consider FIOs with symbols admitting some type ofsingularity at the origin. In this general context, let us choose a sequence Let us consider the symbol

we consider the Fourier integral operator associate to α(·, ·),

the condition

0 < ρ < n/p

implies that the periodic Fourier integral operator A extends to a s -nuclear multilinear operator from 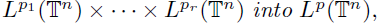for all 1 ≤ p_j < ∞ and 1 ≤ p ≤ ∞. In fact, by Theorem 3.5, we only need to verify that

But, for every ρ > 0, this happens if, and only if, 0 < ρ < n/p.