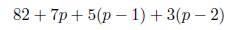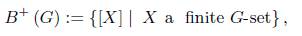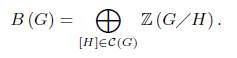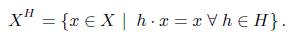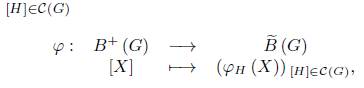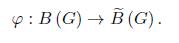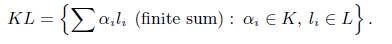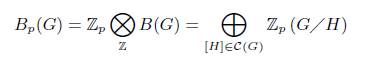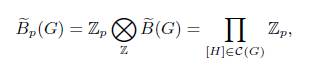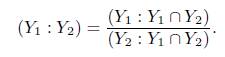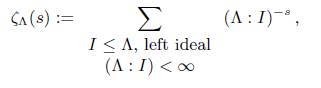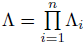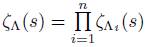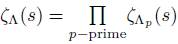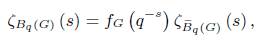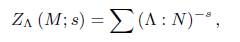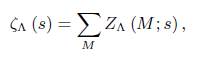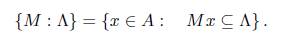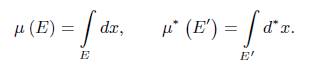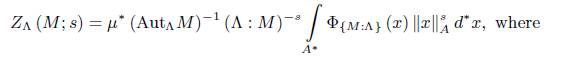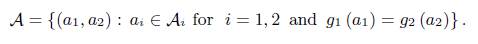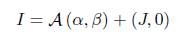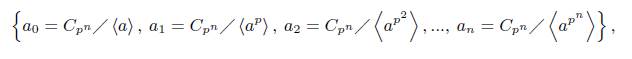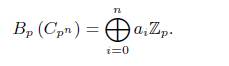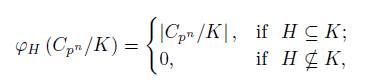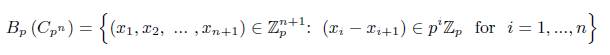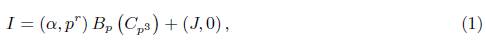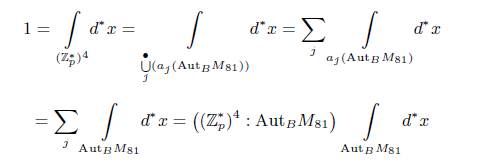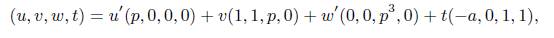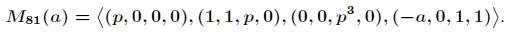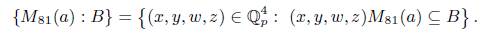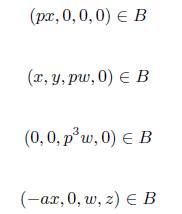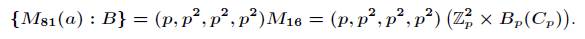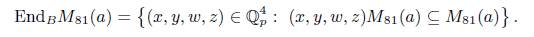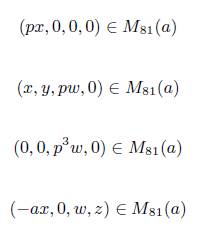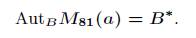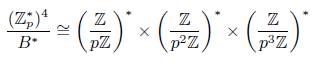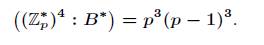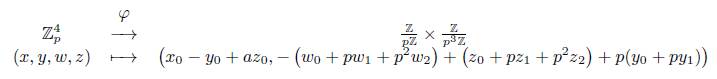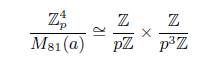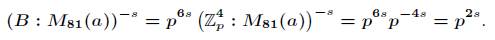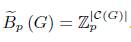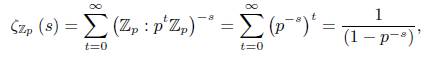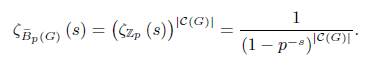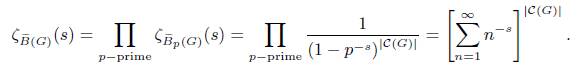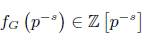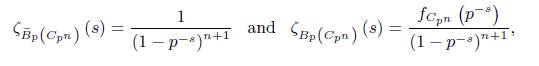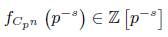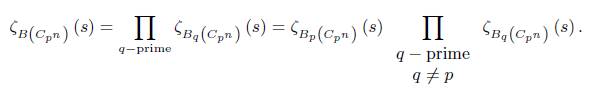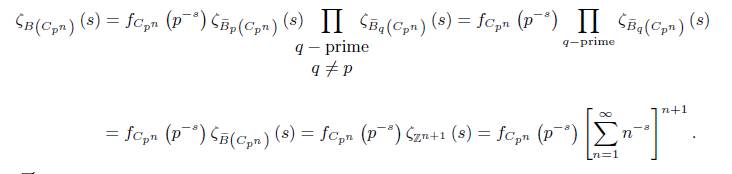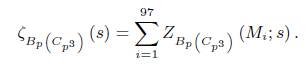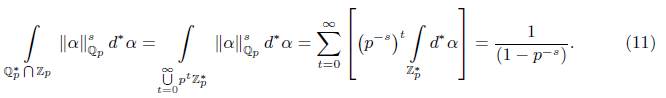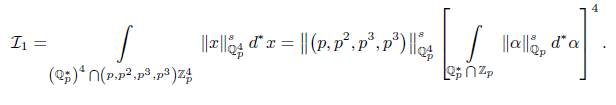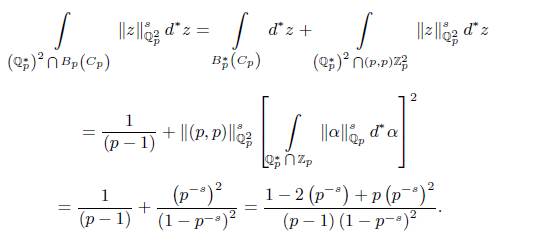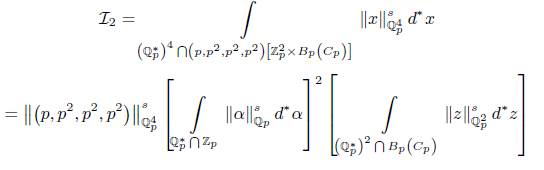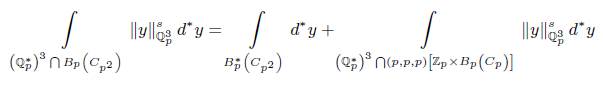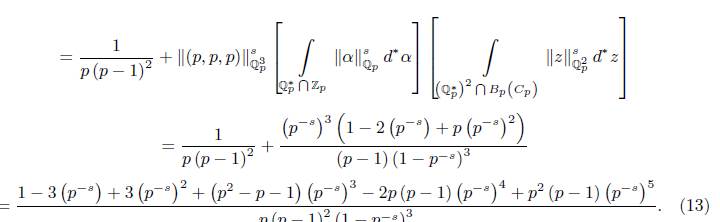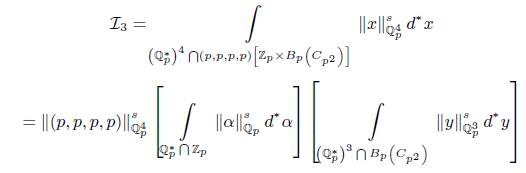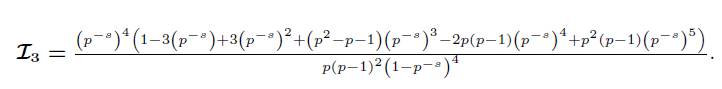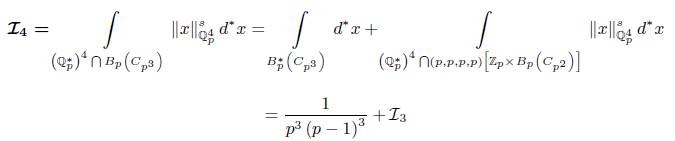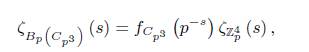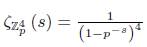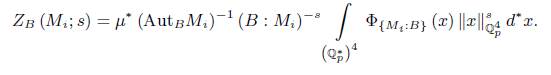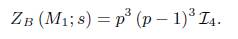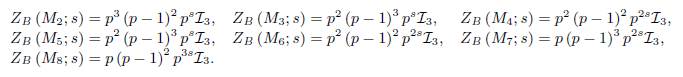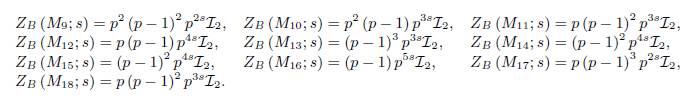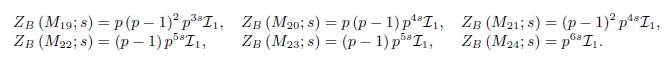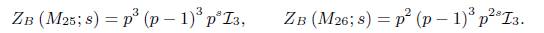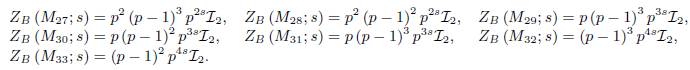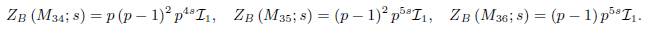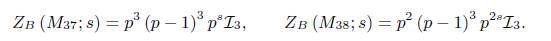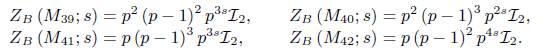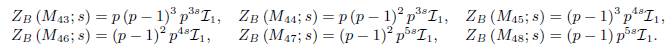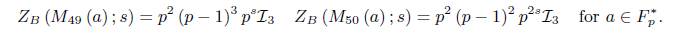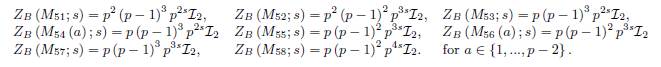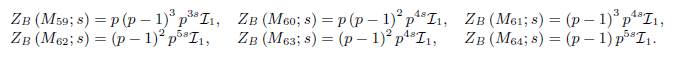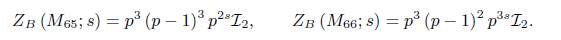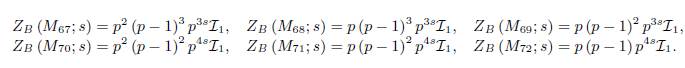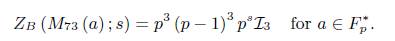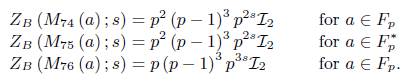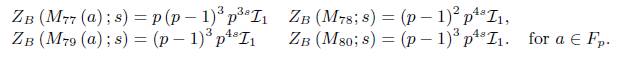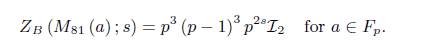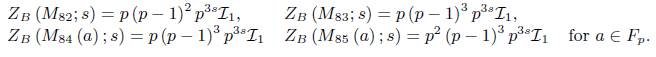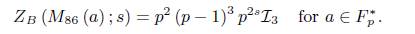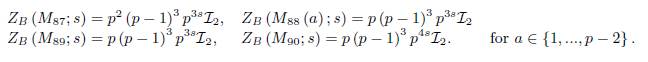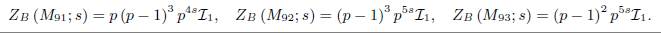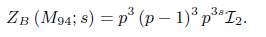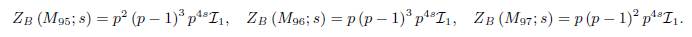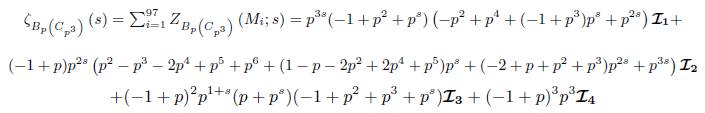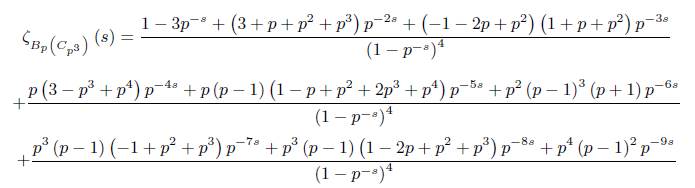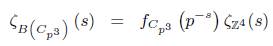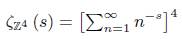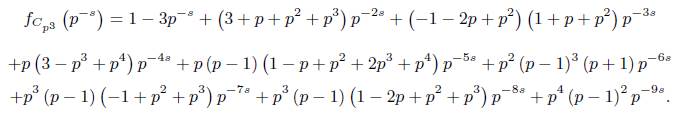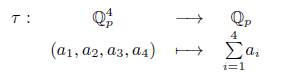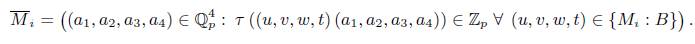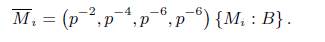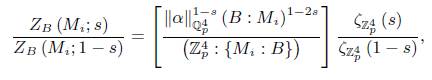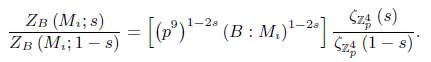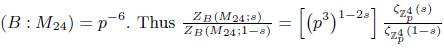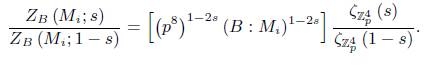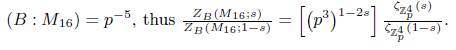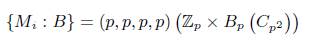Zeta function of the Burnside ring for Cp3

Ramírez-Contreras, Juan Manuel; Vázquez-Rosas, Cristhian; Villa-Hernández, David; Ramírez-Contreras, Juan Manuel; Vázquez-Rosas, Cristhian; Villa-Hernández, David

doi:10.18273/revint.v41n1-2023001

Services on Demand

Journal

Article

Indicators

Cited by SciELO
Access statistics

Revista Integración

Print version ISSN 0120-419XOn-line version ISSN 2145-8472

Integración - UIS vol.41 no.1 Bucaramanga Jan./June 2023 Epub Oct 03, 2023

https://doi.org/10.18273/revint.v41n1-2023001

Artículos originales

Zeta function of the Burnside ring for C_p3

Función Zeta del anillo de Burnside para C_p3

Juan Manuel Ramírez-Contreras^a

Cristhian Vázquez-Rosas^b

David Villa-Hernández^c

^{^a} Universidad Digital del Estado de México, Toluca, México. juan.ramirez@udemex.edu.mx

^{^b} Benemérita Universidad Autónoma de Puebla, Puebla, México. cristhian_vr16@hotmail.com

^{^c} Benemérita Universidad Autónoma de Puebla, Puebla, México. dvilla@fcfm.buap.mx.

Abstract.

The main objective of this paper is to determine the local and global Zeta function of B(C_p3 ) the Burnside ring for cyclic groups of order p³ and, to study some relations that fulfill this Zeta function.

MSC2010:

16H20, 19A22, 11S40.

Keywords: Burnside ring; Zeta function; Fiber product

Resumen.

El objetivo principal de este trabajo es determinar la función Zeta local y global del anillo de Burnside B(C_p3 ) para grupos cíclicos de orden p³, así como estudiar algunas relaciones que satisface esta función Zeta.

Palabras clave: Anillo de Burnside; Función Zeta; Producto fibrado

1. Introduction

The Burnside ring is an invariant of the group that detects solubility, as well as being a framework for the induction theorems and having different applications in topology, see [¹, ²]. On the other hand, the zeta function is an invariant of the ring that detects the distribution of prime ideals, see [⁵].

According to the definition given by Solomon for the zeta function of an order, it is necessary to know all its ideals of finite index, which might be complicated. In this work, we use a method used by Bushnell C. J. and Reiner I. [⁴], which depends only on the finite set of the isomorphism classes of the ideals of finite index. From [¹¹] we have that:

For B_p(C_p) there are 2 isomorphism classes of fractional ideals of finite index; For B_p(C_p2 ) there are 9 isomorphism classes of fractional ideals of finite index.

In both cases, we can see that this is a better alternative than the method used in [¹⁰], where the same results were obtained by computing all the ideals. However, as we will see in this paper, for B_p(C_p3 ) there are

isomorphism classes of fractional ideals of finite index. So for B_p(C_pn ), this method used by I. Reiner quickly becomes unmanageable. At the present, we are trying to discover a method that only depends on the conductors, and we conjecture that there are exactly n + 1 for the general case.

Throughout this paper, let G be a finite group. Its Burnside ring B(G) is the Grothendieck ring of the category of finite left G-sets. This is the free abelian group on the isomorphism classes of transitive left G-sets of the form G H for subgroups H of G, two of which are identified if their stabilizers H are conjugate in G; addition and multiplication are given by the disjoint union and Cartesian product, respectively.

In Section 2, we recall the Burnside ring B (G) of a finite group G, along with the Zeta function ζ_B(G)(s) of B(G) and the ideals of a fiber product of rings.

In Section 3, we determine ζ_{B(Cp3 )}(s). In [⁶] this zeta function was obtained via the calculation of all de ideals of finite index in B(C_p3 ). In this paper, we use a method employed by Bushnell C. J. and Reiner I. [⁴] which only requires the family of all isomorphism classes of the fractional ideals of the finite index of the Burnside ring for this group. First, we recall the ideals of the finite index in B_p C_p2 according to [¹¹], to compute the family of all isomorphism classes of the fractional ideals of the finite index of B_p C_p3 via the fiber product of rings. Next, we determine the Zeta function ζ B~_{(Cp3 )} (s) of the maximal order B~ C_p3 of B(C_p3 ) and the Zeta function ζ_{B (Cp3 )}(s) of the Burnside ring for a cyclic group C_p3 . Finally, we study the relations that fulfill the Zeta functions Z_{Bp(Cp3 )} (M, s), according to [¹², Theorem 2.3] where M is a representative of an isomorphism class of the fractional ideals of finite index of B_p C_p3 .

Remark 1.1. In Section 3, we correct a mistake made in [⁶, pp 17] about the calculation of the index (B : M₈₅(α))^−s , according to which (B : M₈₅(α))^−s = p^s. However, this index must be (B : M₈₅(α))^−s = p^2s, and therefore, we correct ζ_{B(Cp3 )}(s). (In the present paper, M₈₅(a) was reindexed by M₈₁(α).)

2. Zeta Functions of Burnside Rings

Let X be a finite G-set and let [X] be its G isomorphism class. We define

which is a commutative semiring with the unit, with the binary operations of disjoint union and Cartesian product.

Definition 2.1. We define the Burnside ring B (G) of G as the Grothendieck ring of B⁺ (G) .

Now, for subgroup H of G, we write [H] for its conjugacy class. We observe that as an abelian group, B (G) is free, generated by elements of the form G H, where [H] belongs to the set of representatives of all conjugacy classes of subgroups of G, which we call C (G) . That is

For further information about the Burnside ring, see [³].

Let H ≤ G be a subgroup and X a G-set, we denote the set of fixed points of X under the action of H by

We define the mark of H on X as the number of elements of X^H and we call it φ_H (X) . The reader can find some of the properties satisfied by φ_H in [¹², pp 3].

We define e B~ (G) := Π ℤ, thus we have the following map

which is a morphism of semirings that extends to a unique injective morphism of rings

Let R be a Dedekind domain with a quotient field K, and let β be a finite-dimensional K − algebra. For any finite-dimensional K − space V, a full R − lattice in V is a finitely generated R − submodule L in V such that KL = V, where

An R − order in ℬ is a subring Λ of ℬ, such that the center of Λ contains R and such that Λ is a full R − lattice in ℬ.

A fractional ideal of R is a full R − lattice I in K. We can see that there is a non-zero element r ∈ R, such that rI ⊆ R.

Let p ∈ ℤ be a rational prime and let ℤ_p be the ring of p − adic integers. We denote the following tensor products by

and

where we have that B_p(G) is a ℤ_p − order, being B~_p(G) its maximal order. For further information about orders, see [⁷, chapters 2, 3].

Let A be a finite-dimensional semisimple algebra over the rational field ℚ or over a p − adic field ℚ_p, and let Λ be an order in A. When A is a ℚ −algebra, Λ is a ℤ−order in A; when A is a ℚ_p−algebra, Λ is a ℤ_p −order in A. Let I be a left ideal of Λ, such that the index (Λ : I) is finite. We use this index symbol in a general sense: if, for example, Y₁ and Y₂ are ℤ_p − lattices spanning the same ℚ_p − vector space, we put

The symbol (Y₁: Y₂) is therefore unambiguously defined as whether or not Y₁ contains Y₂.

Definition 2.2. We define the Solomon’s zeta function ζ_Λ(s) of an order Λ, as follows:

which is a generalization of the classical Dedekind Zeta function ζ_K (s) of an algebraic number field K. When Λ is a ℤ−order in A, ζ_Λ(s) is the global zeta function; when Λ is a ℤ_p−order in A, ζ_Λ(s) is the local zeta function.

For the commutative rings B_p(G) andB~_p(G), the sum extends over all the ideals of the finite index and converges uniformly on compact subsets of

Let Λ and Λ_i be ℤ -orders, for i = 1, ..., n and let 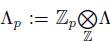, which is an order over ℤ_p. We see that the function ζ satisfies the following properties:

i) If , we have .

ii) , the Euler product.

For further information about Solomon’s Zeta function, see [⁹].

Theorem 2.3. Let G be a finite group and B(G) its Burnside ring, if q ∈ ℤ is a prime, we have

where f_G (q^−s) is a polynomial in ℤ [q^−s] . See [⁹, Theorem 1].

Remark 2.4. If q does not divide |G| , then we have that B_q (G) = B~_q (G) , and we conclude that f_G (q^−s) = 1 when q does not divide |G| .

Definition 2.5. Let M be a full Λ − lattice in A. We define the zeta function Z_Λ (M; s) , as follows:

the sum extending over all full Λ − sublattices N in A, such that N, M are in the same isomorphism class.

So we can express

the finite sum extending over all the representatives of the isomorphism classes of the full Λ − lattices in A.

We define the conductor of M in Λ, as follows:

Let Φ_{M:Λ} be the characteristic function in A of {M : Λ} . Now we choose a Haar measure d^∗x on the unit group A^∗. For measurable sets E ⊂ A, E^′ ⊂ A^∗, it will be convenient to write

We have that:

∥x∥_A = (Lx : L) for x ∈ A^∗, which is independent of the choice of full ℤ_p − lattice L in A, and we observe that it is multiplicative. Furthermore, we can see that ∥x∥_A = 1 whenever x is a unit in some ℤ_p − order in A. For further details on this result, see [⁴, 2.1 The Local Case, pp 138-139].

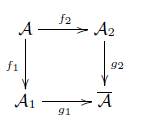
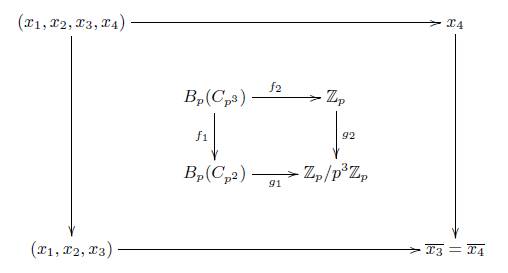
We assume that

is a fiber product diagram of rings, where all the maps are ring surjections. By definition

Let I ≤ A and I_i ≤ A_i be left ideals, such that I_i = f_i (I) for i = 1, 2. Let A₂ be a PID. Then I₂ = A₂β for some β ∈ A₂. We have α ∈ I₁ such that (α, β) ∈ I. Let J= {c ∈ A₁: (c, 0) ∈ I} , which is an ideal of A₁. We have that

and then it is determined by the following data:

a generator β of a principal ideal A₂β of A₂,
an ideal J ≤ A₁ such that g₁ (J) = 0, and
an element α ∈ A₁ such that g₁ (α) = g₂ (β) . Clearly, α is uniquely determined mod J.
Let D = {a ∈ A : f₂ (a) β = 0} which is an ideal of A. We have that f₁ (D) α ⊆ J. For further details on this result, see [⁸].

3. The Zeta function of B (C_p3 )

3.1.Isomorphism classes of the fractional ideals of B_p C_p3

Let p be a prime, and let C_pn = {α} be a ciclyc group of order pⁿ for n ∈ N. We have that the conjugacy classes of C_pn are 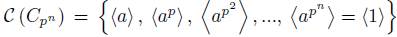. Therefore, a basis for B_p (C_pn ) is

and so,

Furthermore 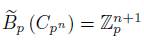is its maximal order.

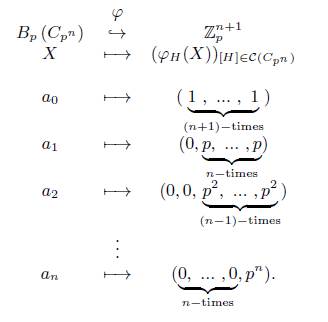
On the other hand, we know that

and then, we have that φ induces the following inclusion:

Therefore, we can see B_p (C_pn ) in B~_p (C_pn ) as follows:

and then we can give the following fiber product structure:

We observe that ℤ_p is a PID. Therefore, it has ideals of the form p^r ℤ_p, for every integer r ≥ 0, and according to the structure of the fiber product, we have that the ideals of finite index in B_p C_p3 are ideals of the form:

where α is an element of B_p (C_p2) and J ≤ Bp (C_p2) is an ideal such that:

g₁ (J) = 0,
g₁ (α) = g₂ (p^r) , where α is uniquely determined mod J, and
if D = pℤ_p × p²ℤ_p × p³ℤ_p × {0} we have that f₁ (D) α ⊆ J.

Let F_p = {0, 1, ..., p − 1} and F_p ^∗ = {1, ..., p − 1} , from [¹¹] we have that the following is a complete list of representatives of isomorphism classes of fractional ideals of B_p C_p2 :

J₁ = Z³ _p

J₂ = (x, y, z) ∈ ℤ³ _p: (y − x) ∈ pℤ_p

J₃ = (x, y, z) ∈ ℤ³ _p: (z − x) ∈ pℤ_p

J₄ = (x, y, z) ∈ ℤ³ _p: (z − y) ∈ pℤ_p

J₅ = (x, y, z) ∈ ℤ³ _p: (z − y) ∈ p²ℤ_p

J₆= (x, y, z) ∈ ℤ³: (y − x) ∈ pZ , (z − y) ∈ pℤp}

J₇ = B_p (C_p2)

J₈ = (x, y, z) ∈ ℤ³ _p: x − y + z ∈ pℤ_p

J₉ = (x, y, z) ∈ ℤ³ _p: px − y + z ∈ p²ℤ_p}

Based on the previous paragraph, we will study Eq. (1), for the nine cases above. We will denote B_p C_p3 by B.

1). From Eq. (1) for J₁, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₁=B = ((1,1,1,1), (0,p,p,p), (0, 0,p²,p²), (0, 0, 0,p³)) , for which: {Mi : B} = B, Aut_BMi = B*, (B : M₁)^-s =1, ((ℤ ^* _p)⁴: B*) = p³(p - 1)³, (ℤ ⁴p : B) = p⁶.

M₂ = {(u,v,w,t) ∈ℤ P : (w - v) ∈ p² ℤ_p, (t - w) ∈ p³ ℤ_p} = ((1, 0, 0, 0), (0,p², 0, 0), (0,1,1,1), (0, 0, 0,p³)) , for which: {M₂: B} = (p,p,p,p) Ms, AutBM2 = M₂ ^*, (B : M₂)^-s = p^s, ((ℤ*_p)⁴: M₂*) = p³(p - 1)², (ℤ⁴p : M₂) = p⁵.

M₃ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) , (w - v) ∈ pℤp, (t - w) ∈ p³ℤ_p} = ((p, 0, 0, 0), (0,p, 0, 0), (1,1,1,1), (0, 0, 0,p³)) , for which: {M₃: B} ( p, p, p, p) Ms, AutBM₃ = M₃*, (B : M₃)^-s = p^s, ((ℤ*_p)⁴: M₃*) = p²(p - 1)³, }ℤ⁴ _p: M₃) = p⁵.

M₄ = (u, v,w, t) ∈ ℤ⁴ _P: (w - v) ∈ pℤ_p, (t - w) ∈ p³ℤ_p} = (1 , 0, 0, 0) , (0, p, 0, 0) , (0, 1 , 1 , 1)₁, (0, 0, 0, p³) ), for which: { M⁴: B} = ( p, p, p, p) Ms, AutBM₄ = M₄*, (B : M₄)^-s = p^2s, ((ℤ*_p)⁴: M₄*) = p²(p - 1)², (ℤ⁴p : M₄) = p⁴.

M₅ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) ∈ pℤp, (w - v) , (t - w) ∈ p²Zp} = ((p, 0, 0, 0), (0,p², 0, 0), (1,1,1,1), (0, 0, 0,p²)) , for which: {M₅: B} = (p,p,p,p) Ms, AutBM₅ = M₅ ^*, (B : M5)^-s = p^s, ((ℤ*_p)⁴: M₅ ^*) = p²(p - 1)³, (ℤ⁴ _p: M₅) = p⁵.

M₆ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - v) , (t - w) ∈ p²ℤp} = 0, 0, 0), (0,p², 0, 0), (0,1,1,1), (0, 0, 0,p²)}, for which: {M₆: B} = (p,p,p,p) Ms, ( p, p, p, p) Ms, ( p, p, p, p) Ms, AutBM₆ = M₆* (B : M₆)^-s = p^2s, ((ℤ*_p)⁴: M₆*) = p²(p - 1)², (ℤ⁴ _p: M₆) = p⁴.

M₇ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) , (w - v) ∈ pℤ_p, (t - w) ∈ p²ℤ_p} = ((p, 0, 0, 0), (0,p, 0, 0), (1,1,1,1), (0, 0, 0,p²)), for which: {M₇: B} = AutBM₇ = M₇*, (B : M₇)^-s = p^2s, ((ℤ *_p)⁴: M₇*) = p(p - 1)³, (ℤ⁴ _p: M₇) = p⁴.

M₈ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - v) ∈ pℤp, (t - w) ∈ p²ℤp} = ( (1 , 0, 0, 0) , (0, p, 0, 0) , (0, 1 , 1 , 1)₁, (0, 0, 0, p² ) )), for which: { M₈: B} = AutBM₈ = M₈*, (B : M₈)^-s = p^3s, ((ℤ*_p)⁴: M₈*) = p(p - 1)², (ℤ⁴ _p: M₈) = p³.

M₉ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) ∈ pℤp, (t - w) ∈ p³ℤp} ((p, 0, 0, 0), (0,1, 0, 0), (1, 0,1,1),(0, 0, 0,p³)), for which: {M₉: B} (p,p²,p²,p²⁾ M₁₆, AutBM₉= M₉*, (B : M*₉)^-s = p^2s, ((ℤ *_p: M₉*) = p²(p - 1)², (ℤ⁴ _p: M₉) = p⁴.

M₁₀ = {(u,v,w,i) ∈ ℤP : (t - w) ∈ p³ ℤ_p} = ((1, 0, 0, 0), (0,1, 0, 0), (0, 0,1,1), (0,0, 0,p³)) , for which: {M₁₀: B} = (p,p²,p²,p²⁾ M₁₆ AutBM₁₀ = M₁₀, (B : M*₁₀)^-s = p^3s, ((ℤ^* _p)⁴: M₁₀) = p²(p - 1), (ℤ⁴ _p: M₁₀ )= p³.

M₁₁ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) ∈ pℤp, (t - w) ∈ p² ℤp} = ⁽(p, 0, 0, 0), (0,1, 0, 0), (1, 0,1,1), (0,0, 0,p²)) , for which: {M₁₁: B} = (p,p²,p²,p²⁾ M₁₆, AutBM₁₁ = M₁₁, (B : M*₁₁)^-s = p^3s, ((ℤ*_p)⁴: M₁₁ ^*) = p(p - 1)², (ℤ⁴ _p: M₁₁) = p³.

M₁₂ = {(u,v,w,t) ∈ ℤ⁴ _p: (t - w) ∈ p²ℤp} = 0, 0, 0), (0,1, 0, 0), (0, 0,1,1), (0,0, 0,p²)) , for which: {M₁₂: B} = (p,p²,p²,p²⁾ M₁₆, AutBM₁₂ = M₁₂, (B : M₁₂)^-s = p^4s, ((ℤ^* _p)⁴: M^* ₁₂) = p(p - 1), (ℤ⁴ _p: M₁₂) = p².

M₁₃ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) , (w - v) , (t - w) G p ℤ p} = ((p, 0, 0, 0), (0,p, 0, 0), (1,1,1,1), (0,0, 0,p)) , for which: {M₁₃: B} = (p,p²,p²,p²⁾ M₁₆ AutBM₁₃ = M₁₃, (B : M*₁₃)^-s = p^3s, ((ℤ^* _p)⁴: M₁₃ ^*) = (p - 1)³, (ℤ⁴ _p: = p³.

M₁₄ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - v) , (t - w) ∈ pℤp} = ((1, 0, 0, 0), (0,p, 0, 0), (0,1,1,1), (0,0, 0,p)) , for which: {M₁₄: B} = (p,p²,p²,p²⁾ M₁₆, AutBM₁₄ = M₁₄, (B : M*₁₄)^-s = p^4s, ((ℤ^* _p)⁴: M₁₄ ^*) = (p - 1)², (ℤ⁴ _p: M₁₄) = p².

M₁₅ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - u) , (t - w) ∈ pℤp} = ((p, 0, 0, 0), (0,1, 0, 0), (1, 0,1,1), (0,0, 0,p)) , for which: {M₁₅: B} = (p,p²,p²,p²) M₁₆, AutBM₁₅ = M₁₅, (B : M*₁₅)^-s = p^4s, ((ℤ^* _p)⁴: M₁₅ ^*) = (p - 1)², (ℤ⁴ _p: M₁₅) = p².

M₁₆ = {(u,v,w,t) ∈ ℤ⁴ _p: (t - w) ∈ p ℤ p} = ((1, 0, 0, 0), (0,1, 0, 0), (0, 0,1,1), (0,0, 0,p)) , for which: {M₁₆: B} = (p,p²,p²,p²) M₁₆, AutBM₁₆ = M₁₆, (B : M₁₆)^-s = p^5s, ((ℤ^* _p)⁴: M₁₆ ^*) = (p - 1), (ℤ⁴ _p: M₁₆) = p.

M₁₇ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - u) , (w - v) ∈ pℤp, (t - v) ∈ p² ℤp} = ⁽(p, 0, 0, 0), (1,1,1,1), (0, 0,p, 0), (0,0, 0,p²)) , for which: {M₁₇: B} = (p,p²,p²,p²) M₁₆, AutBM₁₇ = M*₁₇, (B : M₁₇)^-s = p^2s, ((ℤ^* _p)⁴: M₁₇) = p(p - 1)³, (ℤ⁴ _p: M₁₇) = p⁴.

M₁₈ = {(u, v, w, t) ∈ ℤ⁴ _p: (w - v) ∈ pℤ p, (t - v) ∈ p² ℤp} = 0, 0, 0), (0,1,1,1), (0, 0,p, 0), (0,0, 0,p²)) , for which: {M₁₈: B} = (p,p²,p²,p²) M₁₆, AutBM₁₈ = M₁₈, (B : M*₁₈)^-s = p^3s, ((ℤ^* _p)⁴: M₁₈ ^*) = p(p - 1)², (ℤ⁴ _p: M₁₈) = p³.

M₁₉ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - u) ∈ pℤp, (t - v) ∈ p² ℤ p} = ⁽(p, 0, 0, 0), (1,1, 0,1), (0, 0,1, 0), (0,0, 0,p²)) , for which: {M¹⁹: B} = (p,p²,p³,p³) M₂₄, AutBM₁₉ = M₁₉, (B : M₁₉ ^*)^-s = p^3s, ((ℤ^* _p)⁴: M₁₉ ^*) = p(p - 1)², (ℤ^* _p: M*₁₉) = p³.

M₂₀ = {(u,v,w,t) ∈ ℤ⁴ _p: (t - v) ∈ p² ℤ p) = ((p 0, 0, 0), (0,1, 0,1), (0, 0,1, 0), (0,0, 0,p²)) , for which: {M₂₀: B} = (p,p²,p³,p³) M₂₄, AutBM₂₀ = M^* ₂₀, (B : M₂₀)^-s = p^4s, ((ℤ^* _p)⁴: M*₂₀) =p(p - 1), (ℤ⁴ _p: M^* ₂₀) = p².

M₂₁ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - u) , (t - v) ∈ pZp} , ((p 0, 0, 0), (0,1, 0,1), (0, 0,1, 0), (0,0, 0,p)) , for which: {M₂₁: B} = (p,p²,p³,p³) M₂₄, Aut_BM₂₁ = B^∗ ₂₁, (B : M₂₁)^−s = p^4s, ((ℤ^* _p)⁴: M*₂₁) =p(p - 1), (ℤ⁴ _p: M^* ₂₁) = p²..

M₂₂ = (u, v, w, t) ∈ ℤ⁴ _p: (w − v) ∈ p² ℤ _p, (t − w) ∈ p³ ℤ_p} = ((p 0, 0, 0), (0,1, 0,1), (0, 0,1, 0), (0,0, 0,p)) for which: {M₂₂: B} = (p,p²,p³,p³) M_24, Aut_BM₂₂ = M₂₂ ^∗, (B : M₂₂)^−s = p^4s, ((ℤ^* _p)⁴: M*₂₂) =p(p - 1), (ℤ⁴ _p: M^* ₂₂) = p.

M₂₃ = (u, v, w, t) ∈ ℤ⁴ _p: (w − v) ∈ p² ℤ _p, (t − w) ∈ p³ ℤ_p} = = ⟨(1, 0, 0, 1), (0, 1, 0, 0), (0, 0, 1, 0), (0, 0, 0, p)⟩ for which: {M₂₃: B} = (p,p²,p³,p³) M_23, Aut_BM₂₃ = M₂₃ ^∗, (B : M₂₃)^−s = p^4s, ((ℤ^* _p)⁴: M*₂₃) =p(p - 1), (ℤ⁴ _p: M^* ₂₃) = p.

M₂₄ = ℤ⁴ _p = = ⟨(1, 0, 0, 0), (0, 1, 0, 0), (0, 0, 1, 0), (0, 0, 0, 1)⟩ for which: {M₂₄: B} = (p,p²,p³,p³) M_24, Aut_BM₂₄ = M₂₄ ^∗, (B : M₂₄)^−s = p^4s, ((ℤ^* _p)⁴: M*₂₄) =p(p - 1), (ℤ⁴ _p: M^* ₂₄) = 1.

2). From Eq. (1) for J₂, we obtain the following list of representatives of isomorphism classes

M₂₅ = (u, v, w, t) ∈ ℤ⁴ _p: (w − v) ∈ p² ℤ _p, (t − w) ∈ p³ ℤ_p} = (1, p, 0, 0), (0, p², 0, 0), (0, 0, p², 0), (0, 1, 1, 1) for which: {M₂₅: B} = (p,p,p,p) M_8, Aut_BM₂₅ = M₂₅ ^∗, (B : M₂₅)^−s = p^4s, ((ℤ^* _p)⁴: M*₂₅) =p(p - 1)³, (ℤ⁴ _p: M₂₅) = p⁵.

M₂₆ = (u, v, w, t) ∈ ℤ⁴ _p: (w − v) ∈ p² ℤ _p, (t − w) ∈ p³ ℤ_p} = (1, p, 0, 0), (0, p², 0, 0), (0, 0, p², 0), (0, 1, 1, 1) for which: {M₂₆: B} = (p,p,p,p) M_26, Aut_BM₂₆ = M₂₆ ^∗, (B : M₂₆)^−s = p^4s, ((ℤ^* _p)⁴: M*₅) =p²(p - 1)³, (ℤ⁴ _p: M₂₆) = p⁴.

M₂₇ = {(u,v,w,t) ∈ ℤ⁴ _p: (u - v + t) ∈ p² ℤ _p, (t - w) ∈ p³ ℤ p} = ( (1 , 1 , 0, 0), (0, p, 0, 0), (0, 0, p³ , 0) , (10, 1 , 1 , 1) ) , f)or which: { M₂₇: B} = (p,p²,p²,p²) M₁₆ AutBM₂₇ = M₃ ^*, (B : M₂₇)^-s = p^2s, ((ℤ^* _p)⁴: M^* ₃) = p²(p - 1)³, (ℤ⁴ _p: M₂₇) = p⁴.

M₂₈ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - u) ∈ p² ℤ _p, (t - w) ∈ p³ ℤ p} = (1 , 1 , 0, 0), (0, p, 0, 0), (0, 0, 1 , 1) , (0,10, 0, p³ ) , for which: { M₂₈: B} =(p,p²,p²,p²) M₁₆ AutBM₂₈ = M₂₈*, (B : M₂₈)^-s = p^2s, ((ℤ^*)⁴: M₂₈*) = p²(p - 1)², (ℤ⁴ _p: M₂₈)

M₂₉ = {(u,v,w,t) ∈ ℤ⁴ _p: (u - v + t) ∈ p² ℤ _p, (t - w) ∈ p³ ℤ p } =(1, 1, 0, 0), (0, p, 0, 0), (0, 0, p², 0), (0, 1, 1, 1) , , for which: {M₂₉: B} = (p,p²,p²,p²) M₁₆ AutBM₂₉ = M₇*, (B : M₂₉)^-s = p^3s, ((ℤ^* _p)⁴: M₇*) = p(p - 1)³, (ℤ⁴ _p: M₂₉) = p³.

M₃₀ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - u) ∈ p² ℤ _p, (t - w) ∈ p³ ℤ p } = ((1, 0, 0), (0,p, 0, 0), (0, 0,p², 0), (0, 0,1,1)) , for which: {M₃₀: B} (p,p²,p²,p²) M₁₆ AutBM₃₀ = M^* ₃₀, (B : M₃₀)^-s = p^3s, ((ℤ^* _p)⁴: M₃₀*) = p(p - 1)², (ℤ⁴ _p: M₃₀) = p³.

M₃₁ = {(u, v, w, t) ∈ ℤ⁴ _p: (pu - v + t) ∈ p² ℤ _p (t - w) ∈ p³ ℤ p } = ((1,p, 0, 0), (0,p², 0, 0), (0, 0,p, 0), (0,1,1,1)) , for which: {M₃₁: B} (p,p²,p²,p²) M₁₆ AutBM₃₁ = M₁₇ ^*, (B : M₃₁)^-s = p^3s, ((ℤ^* _p)⁴: M₁₇ ^*) = p(p - 1)³, (ℤ⁴ _p: M₃₁) = p³.

M₃₂ = _{(u,v,w,t) ∈ ℤ⁴ _p: (u - v + t) , (t - w) ∈ p² ℤ _p} = ((1,1, 0, 0), (0,p, 0, 0), (0, 0,p, 0), (0,1,1,1)) , for which: {M₃₂: B} (p,p²,p²,p²) M₁₆ AutBM₃₂ = M^* ₁₃, (B : M₃₂)^-s = p^4s, ((ℤ^* _p)⁴: M₁₃*) = (p - 1)³, (ℤ⁴ _p: M₃₂) = p².

M₃₃ = {(u, v, w, t) ∈ ℤ⁴ _p: (u - v) , (t - w) ∈ p² ℤ _p } = ((1,1, 0, 0), (0,p, 0, 0), (0, 0,p, 0), (0,0,1,1)) , for which: {M₃₃: B} (p,p²,p²,p²) M₁₆, AutBM₃₃ = M^* ₃₃, (B : M₃₃)-^s = p^4s, ((ℤ^* _p)⁴: M₃₃ ^*) = (p - 1)², (ℤ⁴ _p: M₃₃) = p².

M₃₄ = {(u,v,w,t) ∈ ℤ⁴ _p: (pu - v + t) ∈ p² ℤ _p } = ((1,p, 0, 0), (0,p², 0, 0), (0, 0,1, 0), (0,1, 0,1)) , for which: {M₃₄: B} = (p,p²,p³,p³) M_24, AutBM₃₄ = M₁₉ ^*, (B : M₃₄)^-s = p^4s, ((ℤ^* _p)⁴: M₁₉ ^*) = p(p - 1)², (ℤ⁴ _p: M₃₄) = p².

M₃₅ = {(u,v,w,t) ∈ ℤ⁴ _p: (u - v + t) ∈ p² ℤ _p } = ( (1 , 1 , 0, 0) , (0, p, 0, 0) , (0, 0, 1 , 0) , (0,11 , 0, 1)) , for which: { M₃₅: B} (p,p²,p³,p³) M₂₄ AutBM₃₅ = M₂₁ ^*, (B : M₃₅)^-s = p^5s, ((ℤ^* _p)⁴: M₂₁ ^*) = (p - 1)², (ℤ⁴ _p: M₃₅) = p.

M₃₆ = {(u, v, w,t) ∈ ℤ⁴ _p: (u - v) ∈ p² ℤ _p } = ( (1 , 1 , 0, 0) , (0, p, 0, 0) , (0, 0, 1 , 0) , (0,10, 0, 1)) , for which: {M₃₆: B} (p,p²,p³,p³) M₂₄ AutBM₃₆ = M^* ₃₆, (B : M₃₆)^-s = p^5s, ((ℤ^* _p)⁴: M^* ₃₆) = (p - 1), (ℤ⁴ _p: M₃₆) = p.

3). From Eq. (1) for J₃, we obtain the following list of representatives of isomorphism classes

M₃₇ = {(u,v,w,t) ∈ ℤ⁴ _p: (p²u - w + t) ∈ p³ ℤ _p, (t - v) ∈ p² ℤ _p } = (( 1,0,p², 0), (0,p², 0, 0), (0, 0,p³,0), (0,1,1,1)) , for which: {M₃₇: B} (p, p, p, p)M₈, AutBM₃₇ = B*, (B : M₃₇)^-s = p^s, ((ℤ^* _p)⁴: B *) = p³(p - 1)³, (ℤ⁴ _p: M₃₇) = p⁵.

M₃₈ = {(u,v,w,t) ∈ ℤ⁴ _p: (p²u - w + t) ∈ p³ ℤ p, (t - v) ∈ p ℤ p} ={(1,0,p², 0), (0,p, 0, 0), (0, 0,p³, 0), (0,1,1,1)) , for which: {M₃₈: B} = (p,p,p,p) M₈, AutBM₃₈ = M₃*, (B : M₃₈)^-s = p^2s, ((ℤ^* _p)⁴: M₃*) = p²(p - 1)³, (ℤ⁴ _p: M₃₈) = p⁴.

M₃₉ = {(u, v, w,t) ∈ℤ⁴ _p: (p²u - w + t) ∈ p³ ℤ p = {(1, 0,p², 0), (0,1, 0, 0), (0, 0,p³, 0),(0, 0,1,1)) , for which: {M₃₉: B} = (p,p²,p²,p²) M₁₆, AutBM₃₉ = M₉*, (B : M₃₉)^-s = p^3s, ((ℤ^* _p)⁴: M₉*) = p²(p - 1)², (ℤ⁴ _p: M₃₉) = p³.

M₄₀ = {(u, v, w, t) ∈ℤ⁴ _p: (pu - w + t) , (t - v) ∈ p² ℤ p} = {(1,0,p, 0), (0,p², 0, 0), (0, 0,p², 0),(0,1,1,1)) , for which: {M₄₀: B} = (p,p²,p²,p²) M₁₆, AutBM₄₀ = M₅*, (B : M₄₀)^-s = p^2s, ((ℤ^* _p)⁴: M₅ ^*) = p²(p - 1)³, (ℤ⁴ _p: M₄₀ ⁾ = p⁴.

M₄₁ = {(u, v, w, t) ∈ℤ⁴ _p: (pu - w + t) ∈p² ℤ p, (t - v) ∈ p ℤ p} = {(1, 0,p, 0), (0,p, 0, 0), (0, 0,p², 0), (0,1,1,1)) , for which: {M₄₁: B} = (p,p²,p²,p²) M16, AutBM₄₁ = M₇*, (B : M₄₁)^-s = p^3s, ((ℤ^* _p)⁴: M₇*) =p(p - 1)³, (ℤ⁴ _p: M₄₁) = p³.

M₄₂ = {(u, v, w, t) ∈ℤ⁴ _p: (pu - w + t) ∈ p² ℤ p} ={(1,0,p, 0), (0,1, 0, 0), (0, 0,p², 0), (0, 0,1,1)) , for which: {M₄₂: B} = (p,p²,p²,p²) M₁₆, AutBM₄₂ = M^* ₁₁, (B : M₄₂)^-s = p^4s, ((ℤ^* _p)⁴: M^* ₁₁) = p(p - 1)², (ℤ⁴ _p: M₄₂) = p².

M₄₃ = {(u,v,w,t) ∈ℤ⁴ _p: (u - w + t) ∈ p ℤ p, (t - v) ∈ p² ℤ p} = ( (1, 0, 1 , 0), (0, p² , 0, 0) , (0, 0, p, 0) , (0_, , 1 , 1 , 1) ) , for which: { M₄₃: B} = ( p, p² , p³ , p³⁾ M₂₄, AutBM₄₃ = M*₁₇, (B : M₄₃)^-s = p^3s, ((ℤ^* _p)⁴: M₁₇ ^*) = p(p - 1)³, (ℤ⁴ _p: M₄₃) = p³.

M₄₄ = {(u, v, w, t) ∈ℤ⁴ _p: (u - w) ∈ p ℤ _p, (t - v) ∈ p² ℤ _p} = ( (1 , 0, 1 , 0), (0, p² , 0, 0) , (0, 0, p, 0) , (0_, , 1 , 0, 1) ) , for which: { M₄₄: B} = ( p, p² , p³ , p³) M₂₄, AutBM₄₄ = M^* ₄₄, (B : M₄₄)^-s = p^3s, ((ℤ^* _p)⁴: M₁₄) = p(p - 1)², (ℤ⁴ _p: M₄₄) = p³.

M₄₅ = {(u, v, w, t) ∈ℤ⁴ _p: (u - w + t) , (t - v) ∈ p ℤ p} = ((1, 0,1, 0), (0,p, 0, 0), (0, 0,p, 0), (0,1,1,1)) , for which: {M₄₅: B} = (p,p²,p³,p³) M₂₄, AutBM₄₅ = M^* ₁₃, (B : M₄₅)^-s = p^4s, ((ℤ^* _p)⁴: M₁₃ ^*) = (p - 1)³, (ℤ⁴ _p: M₄₅) = p².

M₄₆ = {(u, v, w, t) ∈ℤ⁴ _p: (u - w) , (t - v) ∈ p ℤ p } = ((1, 0,1, 0), (0,p, 0, 0), (0, 0,p, 0), (0,1, 0,1)) , for which: {M₄₆: B} = (p,p²,p³,p³) M₂₄, AutBM₄₆ = M*₄₆, (B : M₄₆)^-s = p^4s, ((ℤ^* _p)⁴: M*₄₆) = (p - 1)², (ℤ⁴ _p: M₄₆ ⁾ = p².

M₄₇ = { (u, v, w, t) ∈ℤ⁴ _p: (u - w + t) ∈ p ℤ _p} = ( (1 , 0, 1 , 0) , (0, 1 , 0, 0) , (0, 0, p, 0) , (0,_, 0, 1 , 1)) , for which: {M₄₇: B} = ( p, p² , p³ , p³) M₂₄, AutBM₄₇ = M*₁₅, (B : M₄₇)^-s = p^5s, ((ℤ^* _p)⁴: M₁₅*) = (p - 1)², (ℤ⁴ _p: M₄₇ ⁾ = p.

M₄₈ = {(u, v, w,t) ∈ℤ⁴ _p: (u - w) ∈ p ℤ _p } = ( (1 , 0, 1 , 0) , (0, 1 , 0, 0) , (0, 0, p, 0) , (0,_, 0, 0, 1)) , for which: {M₄₈: B} = (p, p² , p³ , p³) M₂₄, AutBM₄₈ = M^* ₄₈, (B : M₄₈)^-s = p^5s, ((ℤ^* _p)⁴: M*₄₈) = (p - 1), (ℤ⁴ _p: M₄₈ ⁾ = p.

4). From Eq. (1) for J₄, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₄₉ (α) = {(u, v, w, t) ∈ℤ⁴ _p: (pav - (1 + pa)w + t) ∈ p³ ℤ p, (u - t) , (v - t) ∈ p ℤ p} = ((p, 0, 0, 0), (0,p,p²(1+ pa)^-1a, 0), (0, 0, (1 + pa)^-1p³, 0), (1,1,1,1)) , for a e F_p* and for which: {M₄₉ (a): B} = (p,p,p,p) M₈, Aut_B M₄₉ (a) = M*₄₉ (a) , (B : M₄₉ (a))^-s = p^s, = ((ℤ^* _p)^{4 4}: M₄₉ ^∗(a) = p²(p − 1)³, ℤ ⁴ _p: M₄₉(a) = p⁵.

M₅₀ (α) = {(u, v, w, t) ∈ℤ⁴ _p: (pav - (1 + pa)ω + t) ∈ p³ ℤ p, (v - t) ∈ p ℤ p} = ((1, 0, 0, 0), (0,p,p²(1+ pa)^-1a, 0), (0, 0, (1 + pa)^-1p³, 0), (0,1,1,1)) , for a e F_p* and for whicli: {M₅₀ (a): B} = (p,p,p,p) M₈, Aut_B M₅₀ (a) = M_b (a) , (B : M₅₀ (a))^-s = p^2s, ((ℤ^* _p)⁴: M^* ₅₀,(a)) = p²(p - 1)², (ℤ ⁴ _p: M₅₀(a)) = p⁴.

M₅₁ = {(u,v,w,t) ∈ℤ⁴ _p: (p²v - w + t) ∈ p³ ℤ _p, (u - t) ∈ p ℤ _p} = ((p, 0, 0, 0), (0,1,p², 0), (0, 0,p³, 0),(1, 0,1,1)) , for which: {M₅₁: B} = ⁽p,p²,p²,p²⁾ M₁₆, Aut_BM₅₁ = M₃*, (B : M₅₁)^-s= p^2s, ((ℤ^* _p)⁴: M₃*) =p²(p - 1)³, (ℤ ⁴ _p: M₅₁) = p⁴.

M₅₂ = {(u,v,w,t) ∈ℤ⁴ _p: (p²v - w + t) ∈ p³ ℤ p} =(1, 0, 0, 0),, (0,1,p², 0), (0, 0,p³, 0),(0, 0,1,1)⁾ , for which: {M₅₂: B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₂ = M₄*, (B : M₅₂)^-s = p^3s, ((ℤ^* _p)⁴: M₄*) = p²(p - 1)², (ℤ ⁴ _p: M₅₂ ⁾ = p³.

M₅₃ = {(u, v, w, t) ∈ℤ⁴ _p: (v - w) ∈ p² ℤ p, (v - u) , (t - v) ∈ p ℤ p}= ((p, 0, 0, 0), (1,1,1,1), (0, 0,p², 0), (0, 0, 0,p)) , for which: {M₅₃: B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₃ = M^* ₅₃, (B : M₅₃)^-s = p^2s, ((ℤ^* _p)⁴: M₅₃*) = p(p - 1)³, (ℤ ⁴ _p: M₅₃) = p⁴.

M₅₄ (a) = {(u, v, w, t) ∈ℤ⁴ _p: (av - (1 + a)w + t) ∈ p² ℤ p, (t - u) , (v - t) ∈ p ℤ p} = ⁽(p, 0, 0, 0), (0,p,p(1+ a)^-1a, 0), (0, 0, (1 + a)^-1p², 0), (1,1,1,1)⁾ , for a e {1,...,p - 2} and for which: {M₅₄ (a) : B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₄ (a) = M^* ₅₄ (a) , (B : M₅₄ (a))^-s = p^2s, ((ℤ^* _p)⁴: M₅₄*)) = p(p - 1)³, (ℤ ⁴ _p: M₅₄(a)) = p⁴.

M₅₅ = {(u,v,w,t) ∈ℤ⁴ _p: (v - w) ∈ p² ℤ p, (t - v) ∈p ℤ p} = ( (1 , 0, 0, 0), (0, 1 , 1 , 1) , (0, 0, p² , 0) , (01, 0, 0, p) ) , for which: { M₅₅: B} = ( p, p² , p² , p² ) M₁₆ , Aut_BM₅₅ = M*₅₅, (B : M₅₅)^-s = p^3s, ((ℤ^* _p)⁴: M*₅₅) = p(p - 1)², (ℤ ⁴ _p: M₅₅) = p³.

M₅₆ (a) = {(u, v, w, t) ∈ℤ⁴ _p: (av - (1 + a)w + t) ∈ p² ℤ p, (v - t) ∈ p ℤ p} = 0, 0, 0), (0,p,p(1 + a)^-1a, 0), (0, 0,(1 + a)^-1p², 0), (0,1,1,1)) , for a ∈ {1,...,p - 2} , and for which: {M₅₆ (a) : B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₆ (a) = M*₅₆ (a) , (B : M*₅₆ (a))^-s = p^3s, ((ℤ^* _p)⁴: M*₅₆(a) = p(p - 1)², (ℤ ⁴ _p: M₅₆(a)) = p³.

M₅₇ = {(u, v, w, t) ∈ ℤ⁴ _p: (pv - w + t) ∈ p² ℤ p, (t - u) ∈ p ℤ p} = ((p, 0, 0, 0), (0,1,p, 0), (0, 0,p², 0), (1, 0,1,1)⁾ , for which: {M₅₇: B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₇ = M₇*, (B : M₅₇)^-s = p^3s, ((ℤ^* _p)⁴: M₇*) = p(p - 1)³, (ℤ ⁴ _p: M₅₇) = p³.

M₅₈ = {(u,v,w,t) ∈ ℤ⁴ _p: (pv - w + t) ∈ p² ℤ p} = ((1, 0, 0, 0), (0,1,p, 0), (0, 0,p², 0), (0, 0,1,1)) , for which: {M₅₈: B} = (p,p²,p²,p²) M₁₆, Aut_BM₅₈ = M₈*, (B : M₅₈)^-s = p^4s, ((ℤ^* _p)⁴: M₈*) = p(p - 1)², (ℤ ⁴ _p: M₅₈) = p².

M₅₉ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - pw + t) ∈ p² ℤ p, (t - u) ∈ p ℤ p} = ((p, 0, 0, 0), (0,p², 0, 0), (0,p, 1, 0), (1, -1, 0,1)) , for which: {M₅₉: B} = (p,p²,p³,p³) M₂₄, Aut_BM₅₉ = M*₁₇, (B : M₅₉)^-s = p^3s, ((ℤ^* _p)⁴: M*₁₇) = p(p - 1)³, (ℤ ⁴ _p: M₅₉) = p³.

M₆₀ = { (u, v, w, t) ∈ ℤ⁴ _p: (v - pw + t) ∈ p² ℤ p, } = ( (1 , 0, 0, 0) , (0, p² , 0, 0) , (0, p, 1 , 0) , (0, - 1 , 0, 1) ) , for which: { M₆₀: B} = ( p, p² , p³ , p³) M₂₄, Aut_BM₆₀ = M*₁₈, (B : M₆₀)^-s = p^4s, ((ℤ^* _p)⁴: M₁₈*) = p(p - 1)², (ℤ ⁴ _p: M60) = p².

M₆₁= {(u, v, w, t) ∈ ℤ⁴ _p: (v - w + t) , (t - u) ∈ p ℤ p}= ((p, 0, 0, 0), (0,p, 0, 0), (0,1,1, 0), (1,-1, 0,1)) , for which: {M₆₁: B} = (p,p²,p³,p³) M₂₄, AutBM₆₁ = M*₁₃, (B : M₆₁)^-s = p^4s, ((ℤ^* _p)⁴: M*₁₃ )= (p - 1)³, (ℤ ⁴ _p: M61) = p².

M₆₂ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w + t) ∈ p ℤ p} = ((1, 0, 0, 0), (0,p, 0, 0), (0,1,1, 0), (0,-1, 0,1)) , for which: {M₆₂: B} = (p,p²,p³,p³) M₂₄, AutBM₆₂ = M*₁₄, (B : M₆₂)^-s = p^5s, ((ℤ^* _p)⁴: M₁₄*) = (p - 1)², (ℤ ⁴ _p: M₆₂ ⁾ = p.

M₆₃ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w) , (t - u) ∈ p ℤ p }= ((p, 0, 0, 0), (0,p, 0, 0), (0,1,1, 0), (1,0, 0,1)) , for which: {M₆₃: B} = (p,p²,p³,p³) M₂₄, AutBM₆₃ = M₆₃, (B : M₆₃)^-s = p^4s, ((ℤ^* _p)⁴: M₆₃) = (p - 1)², (ℤ ⁴ _p: M₆₃ ⁾ = p².

M₆₄ = {(u, v, w,t) ∈ ℤ⁴ _p: (v - w) ∈ p ℤ p } = ((1, 0, 0, 0), (0,p, 0, 0), (0,1,1, 0), (0,0, 0,1)) , for which: {M₆₄: B} = (p,p²,p³,p³) M₂₄, AutBM₆₄ = M*₆₄, (B : M₆₄)^-s = p^5s, ((ℤ^* _p)⁴: M₆₄) = (p - 1), (ℤ ⁴ _p: M₆₄ ⁾ = p.

5). From Eq. (1) for J₅, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₆₅ = {(u,v,w,t) ∈ ℤ⁴ _p: (pv - w + t) ∈ p³ ℤ p, (t - u) ∈ p ℤ p} = ((p, 0, 0, 0), (0,1,p, 0), (0, 0,p³, 0),(1, 0,1,1)) , for which: {M₆₅* : B} = (p,p²,p²,p²) M₁₆, AutBM₆₅ = B *, (B : M₆₅*)^-s = p^2s, ((ℤ^* _p)⁴: B *) =p³(p - 1)³, (ℤ ⁴ _p: M₆₅*⁾ = p⁴.

M₆₆ = {(u, v, w,t) ∈ ℤ⁴ _p: (pv - w + t) ∈ p³ ℤ p} = ( (1 , 0, 0, 0), (0, 1 , p, 0), (0, 0, p³ , 0) , (_,0, 0, 1 , 1) ) , for which: { M₆₆: B} = ( p, p² , p² , p^{2 )} M₁₆ , AutBM₆₆ = M₂*, (B : M₆₆)^-s = p^3s, ((ℤ^* _p)⁴: M₂*) = p³(p - 1)², (ℤ ⁴ _p: M₆₆) = p³.

M₆₇ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - w + t) ∈ p² ℤ p, (t - u) ∈ p ℤ p} = ((p, 0, 0, 0), (0,1,1, 0), (0, 0,p², 0), (1, 0,1,1)) , for which: {M₆₇: B} = (p,p²,p³,p³) M₂₄, AutBM₆₇ = M₅*, (B : M₆₇)^-s = p^3s, ((ℤ^* _p)⁴: M₅*) = p²(p - 1)³, (ℤ ⁴ _p: M₆₇) = p³.

M₆₈ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w + pt) ∈ p² ℤ p, (t - u) ∈ p ℤ p} = ((p, 0, 0, 0), (0,1,1, 0), (0, 0,p², 0), (1, 0,p, 1)) , for which: {M₆₈: B} = (p,p²,p³,p³) M₂₄, AutBM₆₈ = M₅₃, (B : M₆₈)^-s = p^3s, ((ℤ^* _p)⁴: M₅₃) = p(p - 1)³, (ℤ ⁴ _p: M₆₈ ⁾ = p³.

M₆₉ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w) ∈ p² ℤ p, (t - u) ∈ p ℤ p} = ⁽(p, 0, 0, 0), (0,1,1, 0), (0, 0,p², 0), (1, 0, 0,1)) , for which: {M₆₉: B} = (p,p²,p³,p³) M₂₄, AutBM₆₉ = M₆₉, (B : M₆₉)^-s = p^3s, ((ℤ^* _p)⁴: M₆₉*} = p(p - 1)², (ℤ ⁴ _p: M₆₉) = p³.

M⁷⁰ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - w + t) ∈ p² ℤ p} = ((1,0 0, 0, 0), (0,1,1, 0), (0, 0,p², 0), (0, 0,1,1)) , for which: {M₇₀: B} = (p,p²,p³,p³) M₂₄, AutBM₇₀ = M₆*, (B : M₇₀)^-s = p^4s, ((ℤ^* _p)⁴: M₆*) = p²(p - 1)², (ℤ ⁴ _p: M₇₀) = p².

M₇₁ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - w + pt) ∈ p² ℤ p} = ( (1 , 0, 0, 0), (0, 1 , 1 , 0) , (0, 0, p² , 0) , (0_, , 0, p, 1) ) , for which: { M₇₁: B} = ( p, p² , p³ , p³) M₂₄, AutBM₇₁ = M₅₅, (B : M₇₁)^-s = p^4s, ((ℤ^* _p)⁴: M*₇₂) = p(p - 1)², (ℤ ⁴ _p: M₇₁) = p².

M₇₂ = {(u,v,w,t) ∈ ℤ⁴ _p: (v - w) ∈ p² ℤ p} = 0, 0, 0), (0,1,1, 0), (0, 0,p², 0), (0, 0, 0,1)) , for which: {M₇₂: B} = (p,p²,p³,p³) M₂₄, AutBM₇₂ = M*₇₂, (B : M₇₂)^-s = p^4s, ((ℤ^* _p)⁴: M₇₂*) = p(p - 1), (ℤ ⁴ _p: M₇₂) = p²

6). From Eq. (1) for J₆, we obtain the following list of representatives of isomorphism classes of fractional ideals of B:

M₇₃ (a) ={(u, v, w,t) ∈ ℤ⁴ _p: (pau - v + t) ∈ p² ℤ p, (p²u - w + t) ∈ p³ ℤ p} = ((1,pa,p², 0), (0,p², 0, 0), (0, 0,p³, 0), (0, 1, 1,1)) , for a ∈ F_p*. {M₇₃ (a) : B} = (p,p,p,p) M₈, AutBM₇₃ (a) = B *, (B : M₇₃ (a))^-s = p^s, ((ℤ^* _p)⁴: B *) = p³(p - 1)³, (ℤ ⁴ _p: M₇₃(a)⁾ = p⁵.

M₇₄ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (p²u - w + t) ∈ p³ ℤ _p, (u - v + at) ∈ p ℤ _p} = ((1, 1,p², 0), (0,p, 0, 0), (0, 0,p³, 0), (0,a, , for a ∈ Fp. {M₇₄ (a) : B} = (p,p²,p²,p²) M₁₆, AutBM₇₄ (a) = M₃*, (B : M₇₄ (a))^-s = p^2s, ((ℤ^* _p)⁴: M₃*) = p²(p - 1)³, (ℤ ⁴ _p: M₇₄(a)) = p⁴.

M₇₅ (a) ={(u, v, w, t) ∈ ℤ⁴ _p: (pau - v + t) ∈ p² ℤ p, (pu - w + t) ∈ p² ℤ p} = ((1,pa,p, 0), (0,p², 0, 0), (0, 0,p², 0), (0,1,1,1)) for a ∈ F_p*. {M₇₅ (a) : B} = (p,p²,p²,p²) M₁₆, AutBM₇₅ (a) = M₅*, (B : M₇₅ (a))^-s = p^2s, ((ℤ^* _p)⁴: M₅*) = p²(p - 1)³, (ℤ ⁴ _p: M₇*(a)) = p⁴.

M₇₆ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (pu - w + t) ∈ p² ℤ _p, (u - v + at) ∈ p ℤ _p} ={(1, 1,p, 0), (0,p, 0, 0), (0, 0,p², 0), (0,a, 1,1)) , for a e Fp. {M₇₆ (a) : B} = (p,p²,p²,p²) M₁₆, AutBM₇₆ (a) = M₇*, (B : M₇₆ (a))^-s = p^3s, ((ℤ^* _p)⁴: M₇*) = p(p - 1)³, (ℤ ⁴ _p: M₇₆(a)) = p³.

M₇₇ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (pu - v + t) ∈ p² ℤ _p, (u - w + at) ∈ p ℤ _p} = ((1,p, 1, 0), (0,p², 0, 0), (0, 0,p, 0), (0,1,a, 1)) , for a ∈ F_p. {M₇₇ (a) : B} = (p,p²,p³,p³) M₂₄, AutBM₇₇ (a) = M*₁₇, (B : M₇₇ (a))^-s = p^3s, ((ℤ^* _p)⁴: M₁₇*) = p}p - 1)³, (ℤ ⁴ _p: M₇₇(a)) = p³.

M₇₈ = {(u, v, w, t) ∈ ℤ⁴ _p: (u - w) ∈ p ℤ p, (u - v) ∈ p ℤ p} = ((1,1,1, 0), (0,p, 0, 0), (0, 0,p, 0), (0,0, 0,1)) , for which: {M₇₈: B} = (p,p²,p³,p³) M₂₄, AutBM₇₈ = M*₇₈, (B : M₇₈)^-s = p^4s, ((ℤ^* _p)⁴: M₇₈*) = (p - 1)², (ℤ ⁴ _p: M₇₈) = p².

M₇₉ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (u - w + t) ∈ p ℤ _p, (u - v + at) ∈ p ℤ _p} = ((1,1,1, 0), (0,p, 0, 0), (0, 0,p, 0), (0,a, 1,1), , for a ∈ F_p. {M₇₉ (a) : B} = (p,p²,p³,p³) M₂₄, AutBM₇₉ (a) = M*₁₃, (B : M₇₉ (a))^-s = p^4s, ((ℤ^* _p)⁴: M*₁₃) = (p - 1)³, (ℤ ⁴ _p: M₇₉(a)) = p².

M₈₀ = {(u, v, w, t) ∈ ℤ⁴ _p: (u - w) ∈ p ℤ p, (u - v + t) ∈ p ℤ p} = ((1,1,1, 0), (0,p, 0, 0), (0, 0,p, 0), (0, 0, 0,1)) , for which: {M₈₀: B} = (p,p²,p³,p³) M₂₄, AutBM₈₀ = M*₁₃, (B : M₈₀)^-s = p^4s, ((ℤ^* _p)⁴: M*₁₃) = (p - 1)³, (ℤ ⁴ _p: M₈₀) = p².

7). From Eq. (1) for J₇, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₈₁ (a) ={ (u, v, w, t) ∈ ℤ⁴ _p: (pv - w + t) ∈ p³ ℤ _p, (u - v + at) ∈ p ℤ _p} = ((p, 0, 0, 0), (1,1,p, 0), (0, 0,p³, 0), (-a, 0,1,1)⁾ for a ∈ F_p. {M₈₁ (a) : B} = (p,p²,p²,p²) M₁₆, AutBM₈₁ (a) = B *, (B : M₈₁ (a))^-s = p^2s, ((ℤ^* _p)⁴: B *) = p³(p - 1)³, (ℤ ⁴ _p: M₈₁(a)) = p⁴.

M₈₂ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w) ∈ p² ℤ p, (u - w) ∈ p ℤ p} = ((p, 0, 0, 0), (0,p², 0, 0), (1,1,1, 0), (0, 0, 0,1)) , for which: {M₈₂: B} = (p,p²,p³,p³) M₂₄, AutBM₈₂ = M*₈₂, (B : M₈₂)^-s = p^3s, ((ℤ^* _p)⁴: M₈₂*) = p(p - 1)², (ℤ ⁴ _p: M₈₂) = p³.

M₈₃ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w) ∈ p² ℤ p, (u - w + t) ∈ p ℤ p} = ((p, 0, 0, 0), (0,p², 0, 0), (1,1,1, 0), (-1, 0, 0,1)⁾ , for which: {M₈₃: B} = (p,p²,p³,p³) M₂₄, AutBM₈₃ = M*₅₃, (B : M₈₃)^-s = p^3s, ((ℤ^* _p)⁴: M*₅₃) = p(p - 1)³, (ℤ ⁴ _p: M₈₃) = p³.

M₈₄ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w + pt) ∈ p² ℤ _p, (u - w + at) ∈ p ℤ _p} = ((p, 0, 0, 0), (0,p², 0, 0), (1,1,1, 0), (-a, -p, 0,1)⁾ for a ∈ F_p. {M₈₄ (a): B} = (p,p²,p³,p³) M₂₄, AutBM₈₄ (a) = M*₅₃, (B : M₈₄ (a))^-s = p^3s, ((ℤ^* _p)⁴: M₅₃) = p(p - 1)³, (ℤ ⁴ _p: M₈₄( a)= p³.

M₈₅ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (v - w + t) ∈ p² ℤ p, (u - w + at) ∈ p ℤ p} = ( ( p, 0, 0, 0) , (0, p² , 0, 0) , (1 , 1 , 1 , 0) , ( - a, - 1 , 0, 1) ) for a ∈ F_p. { M₈₅ ( a) : B} = (p,p²,p³,p³) M₂₄, AutBM₈₅ (a) = M*, (B : M₈₅ (a))^-s = p^3s, ((ℤ^* _p)⁴: M₅*) = p²(p - 1)³, (ℤ ⁴ _p: M₈₅ ( a) ) = p³.

8). From Eq. (1) for J₈, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₈₆ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (pav - (1 + pa)w - p²u + t) ∈ p³ ℤ _p, (v - t) ∈ p ℤ _p} = 0, -p²(1+ pa)^-1, 0), (0,p,p²a(1+ pa)^-1, 0), (0, 0, 0,p³), (0,1,1,1)) , for a ∈F_p*. ,M₈₆(a): B} = (p,p,p,p) M₈, Aut_BM₈₆ (a) = M₄₉* (a) , (B : M₈₆ (a))^-s = p^2s, ((ℤ^* _p)⁴: M₄₉*(a) = p²(p - 1)³, (ℤ ⁴ _p: M₈₆(a)) = p⁴.

M₈₇ (a) = {(u, v, w, t) ∈ ℤ⁴ _p: (p²u - w- p²u+t) ∈ p³ ℤ _p, = (1, 0,−p², 0), (0, 1, p², 0), (0, 0, p³, 0), (0, 0, 1, 1), for which: {M₈₇: B} = (p, p², p², p²) M₁₆, AutBM₈₇ = M₃*, (B : M₈₇)^-s = p^3s, ((ℤ^* _p)⁴: M₃*) = p²(p - 1)³, (ℤ ⁴ _p: M₈₇) = p³.

M₈₈ (a) = { (u, v, w, t) ∈ ℤ⁴ _p: (av - (1 + a)w - pu + t) ∈ p² ℤ _p, (v - t) ∈ p ℤ _p} = {(1, 0, -p(1+ a)^-1, 0), (0,p,pa(1+ a)^-1, 0), (0, 0,p², 0), (0,1,1,1)) , for a e {1,...,p - 2} {M₈₈(a): B} = (p,p²,p²,p²) M₁₆, Aut_BM₈₈ (a) = M*₅₄ (a) , (B : M₈₈ (a))^-s = p ((ℤ^* _p)⁴: M*₅₄(a) = p(p - 1)³, (ℤ ⁴ _p: M₈₈(a)⁾ = p³.

M₈₉= {(u, v, w, t) ∈ ℤ⁴ _p: (v - w - pu) ∈ p² ℤ p, (v - t) ∈ p ℤ p} = {(1, 0, -p, 0), (0,1,1,1), (0, 0,p², 0),(0, 0, 0,p)) , for which: {M₈₉: B} = (p,p²,p²,p²) M₁₆, AutBM₈₉ = M*₅₃, (B : M₈₉)^-s = p^3s, ((ℤ^* _p)⁴: M₅₃) = p(p- 1)³, (ℤ ⁴ _p: M₈₉) = p³.

M₉₀ = {(u, v, w, t) ∈ ℤ⁴ _p: (pv - pu - w + t) ∈ p² ℤ p} = {(1, 0, -p, 0), (0,1,p, 0), (0, 0,p², 0),(0, 0,1,1)) , for which: {M₉₀: B} = (p,p²,p²,p²) M₁₆, AutBM₉₀ = M₇*, (B : M₉₀)^-s = p^4s, ((ℤ^* _p)⁴: M₇*) = p(p - 1)³, (ℤ ⁴ _p: M₉₀) = p².

M₉₁ = {(u, v, w, t) ∈ ℤ⁴ _p: (pu - v + pw + t) ∈ p² ℤ p} = ((1,p, 0, 0), (0,p², 0, 0), (0,p, 1, 0), (0,1, 0,1)) , for which: {M₉₁: B} = (p,p²,p³,p³) M₂₄, AutBM₉₁ = M*₁₇, (B : M₉₁)^-s = p^4s, ((ℤ^* _p)⁴: M*₁₇) =p(p - 1)³, (ℤ ⁴ _p: M₉₁ ⁾ = p².

M₉₂ = {(u, v, w, t) ∈ ℤ⁴ _p: (v - u - w + t) ∈ p ℤ p} = ((1,1, 0, 0), (0,p, 0, 0), (0,1,1, 0), (0,-1, 0,1)) , for which: {M₉₂: B} = (p,p²,p³,p³) M₂₄, AutBM₉₂ = M*₁₃, (B : M₉₂)^-s = p^5s, ((ℤ^* _p)⁴: M*₁₃} = (p - 1)³, (ℤ ⁴ _p: M92) = p.

M⁹³ = {(u, v, w,t) ∈ ℤ⁴ _p: (v - w - u) ∈ p ℤ p} = ( (1 , 1 , 0, 0) , (0, p, 0, 0) , (0, 1 , 1 , 0), (0,_, 0, 0, 1) ) , for which: { M₉₃: B} = ( p, p² , p³ , p³) M₂₄, AutBM₉₃ = M₇₈*, (B : M₉₃)^-s = p^5s, ((ℤ^* _p)⁴: M₇₈*) = (p - 1)², (ℤ ⁴ _p: M₉₃ ⁾ = p.

9). From Eq. (1) for J₉, we obtain the following list of representatives of isomorphism classes of fractional ideals of B :

M₉₄ = {(u, v, w, t) ∈ ℤ⁴ _p: (pv - w - p²u + t) ∈ p³ ℤ p} = {(1, 0, -p², 0), (0,1,p, 0), (0, 0,p³, 0), (0, 0,1,1)}, for which: {M₉₄: B} = (p,p²,p²,p²) M₁₆, AutBM₉₄ = B *, (B : M₉₄)^-s = p^3s, ((ℤ^* _p)⁴: B *) = p³(p- 1)³, (ℤ ⁴ _p: M94) = p³.

M₉₅ = {(u, v, w, t) ∈ ℤ⁴ _p: (pu + w - v + t) ∈ p² ℤ p} = ( (1 , 0, - p, 0) , (0, 1 , 1 , 0) , (0, 0, p² , 0) ,_, (0, 0, - 1 , 1)}, for which: { M₉₅: B} = ( p, p² , p³ , p³) M₂₄, AutBM₉₅ = M₅*, (B : M₉₅*)^-s = p^4s, ((ℤ^* _p)⁴: M*₅) = p²(p- 1)³, (ℤ ⁴ _p: M₉₅) = p².

M₉₆ = {(u, v, w, t) ∈ ℤ⁴ _p: (pu + w - v + pt) ∈ p² ℤ p} = ( (1 , 0, - p, 0) , (0, 1 , 1 , 0) , (0, 0, p² , 0) , _,(0, 0, - p, 1) ) for which: { M₉₆: B} = ( p, p² , p³ , p³) M₂₄, AutBM₉₆ = M₅₃*, (B : M₉₆)^-s = p^4s, ((ℤ^* _p)⁴: M₅₃) = p(p - 1)³, (ℤ ⁴ _p: M₉₆) = p².

M₉₇ = {(u, v, w, t) ∈ ℤ⁴ _p: (pu + w - v) ∈ p² ℤ p} = ( (1 , 0, - p, 0) , (0, 1 , 1 , 0) , (0, 0, p² , 0) , _,(0, 0, 0, 1) ) , for which: { M₉₇: B} = ( p, p² , p³ , p³) M₂₄, AutBM₉₇ = M₈₂*, (B : M₉₇)^-s = p^4s, ((ℤ^* _p)⁴: M*₈₂) = p(p - 1)², (ℤ ⁴ _p: M97) = p².

Remark 3.1. We have that M₁,...,M₉₇ form a set of representatives of all the isomorphism classes of fractional ideals of finite index in B_p (C_p3( . Inn the previous list we observe that (lie only conductors are B_p (C_p3) , (p,p,p,p) (ℤ _p x B_p (C_p2)) , (p,p²,p²,p²) (ℤ p x B_p (C_p)) and ((p,p²,p³,p³)) ℤ p. Therefore, in the following subsection (The Local Zeta Function for B_p (C_p ³)) it will be sufficient to compute the integrals corresponding to these four conductors.

Remark 3.2. The way to get {M_i: B} , Aut_BM_i, (B : M_i) ^-s , ((ℤ *_p)⁴: (ℤ _p ⁴: M_i ), for i = 1,... , 97, is very similar. As an example, we present a sketch of proof for M₈₁:

We choose a Haar measure d*x on (ℚ_p ^*)⁴ , such as

and then, we have that μ∗(Aut_BM₈₁)⁻¹ =(( ℤ ∗_p)⁴: Aut_BM_81).

Now, if M₈₁ (a) =(u, v,w, t) ∈ ℤ⁴ _p: (pv − w + t) ∈ p³ ℤ_p, (u − v + at) ∈ p ℤp for a ∈ F_p, and (u, v,w, t) ∈ M₈₁(a), then w = t+pv +p³w′ and u = v −at+pu′ for w′, u′ ∈ ℤ_p. It follows that.

and it is easy to see that as ℤ_p−module

First, let’s see how to calculate {M₈₁(a) : B} . We know that

If (x, y,w, z) ∈ {M₈₁(a) : B} , then from Eq. (2) we have that

From Eq. (6) it follows that w ∈ p²ℤ_p and z −w ∈ p³ℤ_p, then z ∈ p²ℤ_p. From Eq. (4) it follows that pw − y ∈ p²ℤ_p and y − x ∈ pℤ_p, then y ∈ p²ℤ_p and x ∈ pℤ_p. From Eq. (3) and Eq. (5) it follows that x ∈ ℤ_p and w ∈ ℤ_p respectively, then y, z ∈ ℤ_p. So, it is easy to see that

Next, let’s see how to calculate Aut_BM₈₁(a). We know that

If (x, y, w, z) ∈ End_BM₈₁(a), then from Eq. (2) we have that

From Eq. (8) and Eq. (10), it follows that y − x ∈ pℤ_p, w − y ∈ p²ℤ_p and z − w ∈ p³ℤ_p. From Eq. (7) and Eq. (9), it follows that x, w ∈ ℤ_p respectively, and then y, z ∈ ℤ_p. So, it is easy to see that End_BM₈₁(a) = M₁ = B, and then

To calculate µ^∗(Aut_BM₈₁(a))⁻¹ = ((ℤ∗_p)⁴: B∗). Let

where x = x₀ + px₁ + p²x₂ + · · · ; y = y₀ z = z₀ + pz₁ + p²z₂ + · · · , for x₀, y₀, w₀, z₀ + py₁ + p²y₂ + · · · ; w = w₀ + pw₁ + p²w₂ + · · · ; ∈ F_p ^∗ and x_i, y_i, w_i, z_i ∈ F_p for i = 1, 2, ...; It is easy to see that φ is a surjective homomorphism of multiplicative groups, such that ker(φ) = B^∗. The first isomorphism theorem gives

and then

To calculate ℤ ⁴ _p: M₈₁(a) . Let

where x = x₀ + px₁ + p²x₂ + · · · ; y = y₀ + py₁ + p²y₂ + · · · ; w = w₀ + pw₁ + p²w₂ + · · · ; z= z₀ + pz₁ + p²z₂ + · · · , and x_i, y_i, w_i, z_i ∈ F_p for i = 0, 1, 2, ...; It is easy to see that φ is a surjective homomorphism of additive groups, such that ker(φ) = M₈₁(a). The first isomorphism theorem gives

and then

Finally, we know that (M₈₁(a) : B) = (M₈₁(a) : ℤ_p ⁴) (ℤ⁴ _p: B = p⁶ (ℤ⁴: M₈₁(a) ⁻¹ then

3.2. The Zeta function of B C_p3

Proposition 3.3. Let G be a finite group and let 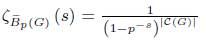B~ (G) have that and 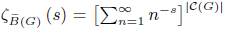Moreover, .

Proof. We have that e . Since

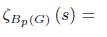
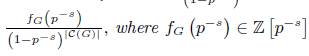It follows that

Now, by the Euler product, we have that

Finally, from the Theorem 2.3 we obtain that

Where .

Corollary 3.4. Let n ∈ ℕ and B (C_p ⁿ) be the Burnside ring for a cyclic group of order pn, and let e 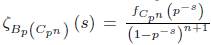B~ (C_p ⁿ) be its maximal order. Then we have that , where . Moreover, .

Proof. We have that there are n + 1 conjugacy classes of C_pn , therefore 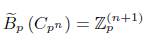, then from the above proposition, it follows that

Where

Now, by the Euler product, it follows that

Now, by Remark 2.4, since f_Cpn (q^−s )=1, when q # p, according to Theorem 2.3 we obtain:

The Local Zeta Function for B_p C_p3

Remember that:

Hence, to compute the zeta function of B_p C_p3 , it is necessary to compute Z_{Bp Cp3} (M_i; s) for i = 1, . . . , 97. According to the previous subsection, we only need to compute the integrals that we will study in the following four Remarks.

Remark 3.5. We choose a Haar measure d∗x on (ℚ∗_p)⁴ , such that d∗x = (d∗α)⁴ , where d∗α is a Haar measure of ℚ∗_p such that 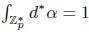. Thus

Besides, we have

Thus, from Eq. (11), we obtain:

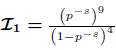
Remark 3.6. We choose a Haar measure d* z on (ℚ∗_p)², such that d* z = (d* α)². We know that, B_p (C_p) is local, where rad (B_p (C_p)) = (p, p) ℤ_p ². Thus 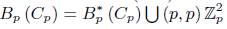and then

Besides. We have:

Thus, from Eq. (11) and Eq. (12), we obtain: .

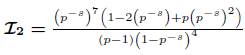Remark 3.7. We choose a Haar measure d* y on (ℚ∗p)3 such that d* y = (d*α)3. We know that, Bp (Cp2) is local, where rad (Bp (Cp2)) = (p,p,p)[ ℤp x Bp (Cp)]. Thus 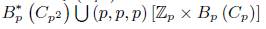and then

Besides, we have:

Thus, from Eq. (11) and Eq. (13), we obtain:

Remark 3.8. We know that, Bp (Cp3) is local, where rad (Bp (Cp3) ) (p,p,p,p) { ℤp x Bp Cp2)]. Thus 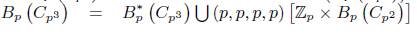and

Proposition 3.9. Let p be a rational prime and let B = Bp (Cp3) be the Burnside ring for a cyclic group Cp3 of order p3. Therefore, the zeta function will be:

Where and

Proof. Remember that:

Hence, from case 1) in subsection 3.1, along with Remark 3.8, we obtain:

From case 1) in subsection 3.1, along with Remark 3.7, we obtain:

From case 1) in subsection 3.1, along with Remark 3.6, we obtain:

From case 1) in subsection 3.1, along with Remark 3.5, we obtain:

From case 2) in subsection 3.1, along with Remark 3.7, we obtain:

From case 2) in subsection 3.1, along with Remark 3.6, we obtain:

From case 2) in subsection 3.1, along with Remark 3.5, we obtain:

From case 3) in subsection 3.1, along with Remark 3.7, we obtain:

From case 3) in subsection 3.1, along with Remark 3.6, we obtain:

From case 3) in subsection 3.1, along with Remark 3.5, we obtain:

From case 4) in subsection 3.1, along with Remark 3.7, we obtain:

From case 4) in subsection 3.1, along with Remark 3.6, we obtain:

From case 4) in subsection 3.1, along with Remark 3.5, we obtain:

From case 5) in subsection 3.1, along with Remark 3.6, we obtain:

From case 5) in subsection 3.1, along with Remark 3.5, we obtain:

From case 6) in subsection 3.1, along with Remark 3.7, we obtain:

From case 6) in subsection 3.1, along with Remark 3.6, we obtain:

From case 6) in subsection 3.1, along with Remark 3.5, we obtain:

From case 7) in subsection 3.1, along with Remark 3.6, we obtain:

From case 7) in subsection 3.1, along with Remark 3.5, we obtain:

From case 8) in subsection 3.1, along with Remark 3.7, we obtain:

From case 8) in subsection 3.1, along with Remark 3.6, we obtain:

From case 8) in subsection 3.1, along with Remark 3.5, we obtain:

From case 9) in subsection 3.1, along with Remark 3.6, we obtain:

From case 9) in subsection 3.1, along with Remark 3.5, we obtain:

From the 97 partial zeta functions above, we obtain that:

and finally, from Remarks 3.5 to 3.8, we obtain that

The Global Zeta Function for B Cp3 .

By Corollary 3.4, it follows that where we have that and

3.3. Some relations for ZB (Mi; s)

Lastly, we will study a couple of relations that satisfy the zeta functions ZB (Mi; s) .

Let τ be the mapping such that

We will denote by

a). For each

we have that {Mi : B} = p, p2, p3, p3 ℤ4p which satisfy . Thus

Hence, according to the functional equation given in [¹², Theorem 2.3] the following relations are fulfilled:

Where 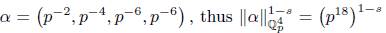besides, 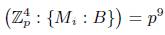therefore, from Eq. (14) we obtain

For example .

b). For each i ∈ {9, ..., 18, 27, ..., 33, 39, ..., 42, 51, ..., 58, 65, 66, 74, ..., 76, 81, 87, ..., 90, 94} we have that 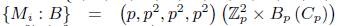from which we obtain 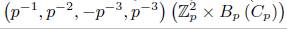thus

Hence, for 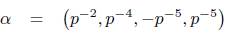we have that 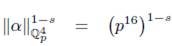, besides, 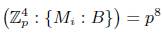, therefore, from Eq. (14) we obtain

For example

c). For each i ∈ {2, ..., 8, 25, 26, 37, 38, 49, 50, 73, 86} we have that

from which we obtain 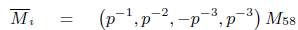therefore, the condition required in functional equation given in [¹², Theorem 2.3], is not fulfilled.

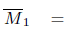
d). Finally, for i = 1 we have that {M₁: B} = B which satisfies 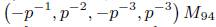therefore, the condition required in the functional equation given in [¹², Theorem 2.3], is not fulfilled.

Acknowledgements:

The authors would like to thank the referees for their valuable sugges-tions and comments.

References

[1] Carlsson G., “Equivariant stable homotopy and Segal’s Burnside ring conjecture”, Ann. Math. 120 (1984), No. 2, 189-224. doi: 10.2307/2006940 [ Links ]

[2] Carlsson G., “Segal’s Burnside ring conjecture and related problems in topology”, Proceed-ings of the International Congress of Mathematicians, Berkeley, USA, 1, 574-579, August 3-11, 1986. [ Links ]

[3] Bouc S., “Burnside rings”, in Handbook of Algebra (Hazewinkel M.), Elsevier, vol. 2 (2000), 739-804. doi: 10.1016/S1570-7954(00)80043-1 [ Links ]

[4] Bushnell C.J. and Reiner I., “Zeta functions of arithmetic orders and Solomon’s conjec-tures”, Math. Z. 173 (1980), No. 2 135-161. doi: 10.1007/BF01159955 [ Links ]

[5] Bushnell C. J. and Reiner I., “New asymptotic formulas for the distribution of left ideals of orders”, Math. 364 (1986), 149-170. doi: 10.1515/crll.1986.364.149 [ Links ]

[6] Ramírez-Contreras J. M. and Villa-Hernández D., “Solomon’s Zeta function of Bp Cp3 ”, Int. Electron. J. Algebra 20 (2016), 1-27. doi: 10.24330/ieja.266181 [ Links ]

[7] Reiner I., Maximal Orders, Academic Press, London-New York, 1975. [ Links ]

[8] Reiner I., “Zeta functions of integral representations”, Comm. in Algebra 8 (1980), No. 10, 911-925. doi: 10.1080/00927878008822498 [ Links ]

[9] Solomon L., “Zeta Functions and Integral Representation Theory”, Advances in Math. 26 (1977), No. 3, 306-326. doi: 10.1016/0001-8708(77)90044-5 [ Links ]

[10] Villa-Hernández D., “Zeta functions of Burnside rings of grups of order p and p2”, Communications in Algebra, 37 (2009), No 5, 1758-1786. doi: 10.1080/00927870802216404 [ Links ]

[11] Villa-Hernández D., “Zeta function of the Burnside ring for cyclic groups”, Int. J. Algebra 5 (2011), No 26, 1255-1266. [ Links ]

[12] Villa-Hernández D., “Functional Equations for Zeta functions of Burnside rings”, JP J. Algebra Number Theory Appl. 29 (2013), No 1, 1-16. [ Links ]

[13] Wolfram Research, Inc., Mathematica, Version 10.0, Champaign, IL, 2014. [ Links ]

To cite this article: J.M. Ramírez-Contreras, C. Vázquez-Rosas & D. Villa-Hernández, Zeta function of the Burnside ring for C_p3 , Rev. Integr. Temas Mat., 41 (2023), No. 1, 1-26. doi: 10.18273/revint.v41n1-2023001

Received: October 12, 2022; Accepted: March 19, 2023

This is an open-access article distributed under the terms of the Creative Commons Attribution License