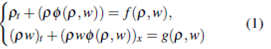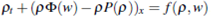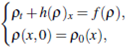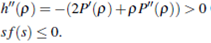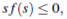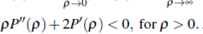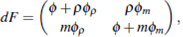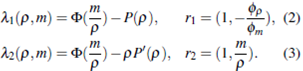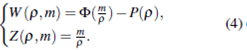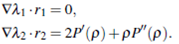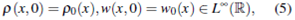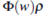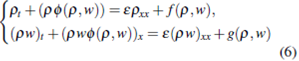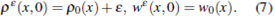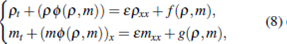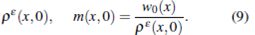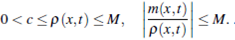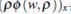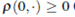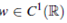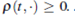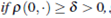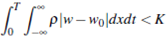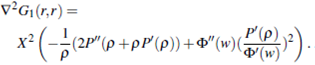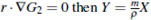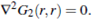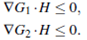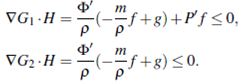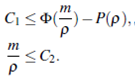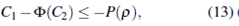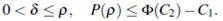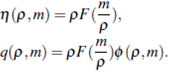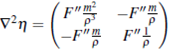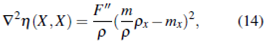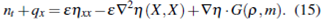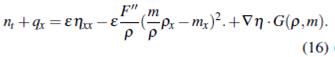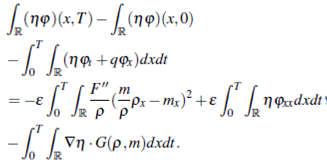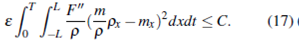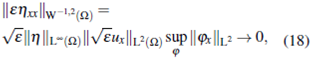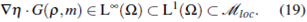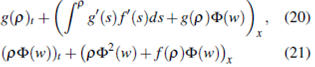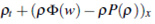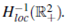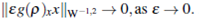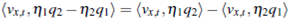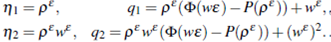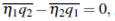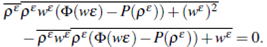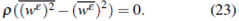1 Introduction

Many of the natural phenomena are modeled by partial differential equations. Some of the most common partial differential equations are the wave equation, the heat equation, the Schrõndinger equation and KdV equation. In this type of problems it is interesting to study the existence and uniqueness of solutions, which in many cases is not an easy task. For example, in the review article ¹ the author studies the existence and uniqueness of solutions for non-linear Schrõndinger equation. However, in the hyperbolic equations the existence and uniqueness of solutions are a open problems. The goal this paper show the existence of solutions for a hyperbolic system of conservation laws. We consider the balanced non-symmetric system

where 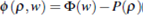, 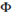a convex function. This system was considered in ² where the author showed the existence of global weak solution for the homogeneous system (1). Another system of the type (1) was considered in ³ as a generalization to the scalar Buckley-Leverett equations describing two phase flow in porous media. The system (1), recently, has been object of constant studies, in ⁴ the author considered the particular case in which 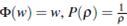, in this case the two characteristics of the system (1) are linear degenerate, solving the Riemann problem the existence and uniqueness of delta shock solution were established. 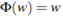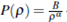In this line in (⁵) the authors considered the case and with 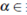(0,1), the existence and uniqueness of solutions to the Riemann problem was got by solving the Generalize Rankine-Hugoniot condition. In both cases, when 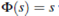the system (1) models vehicular traffic flow in a highway without entry neither exit of cars, in this case the source term represents the entry or exit of cars see ^6),(7),(8 and reference therein for more detailed description of source term.

Notice that when w is constant, the system (1) reduces to the scalar balance laws

,

and from the second equation in (1), g should be of the form

g = wf.

Moreover, if we make h(p) = 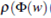- P(p)) the global weak solution of the Cauchy problem

there exists if h(p) is a convex function and the source term is dissipative i.e.,

For details on the above result see [9], [10] and references therein. In this work we assume the following conditions,

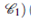f, g are Lipschitz functions such that

wf (P, w)= g(p, w), f (0,0)= 0.

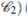There exist a constant M > 0 such that

for [S]> M.

The function P(p) satisfies

P(0) = 0, lim pP'(p) = 0, lim P(p) = ∞,

Remark 1.1. By example if f (p, w) = p, then g(p, w) = pw. In this case we have the non-symmetric system with lineal damping.

Making m = pw, system (1) can be transformed in a symmetric system

For this system, making F (p, m) = 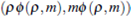, we obtain

so the eigenvalues and eigenvector of dF are given by

From (2) and (3), the k-Riemann invariants are given by

Moreover,

By 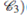condition, the system (6) is linear degenerate in the first characteristic field, non linear degenerate in the second characteristic field and non strictly hyperbolic. In this paper we obtain the main following theorem

Theorem 1.2. If the initial data

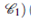
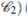
with.The total variation of the Riemann invariants W0(x) be bounded, and the conditions 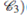^{_{holds, then the Cauchy problem (1), (5) has a global bounded weak entropy solution and wx(x, t) is bounded in L1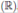}} . Moreover for w constant, P is the global weak solution of the scalar balance laws

Pt + h(P )x = f (P),

where h(p) = - pP(p), f (p) = f (p, w).

2 A priori bounds and existence

In order to get weak solutions, in this section we investigate the problem of the existence of the solutions for the parabolic regularization to the system (1)

with initial data

We consider the transformation m = pw, replacing in (6) we have

with initial data

Proposition 2.1. _{^{Let £ > 0. The Cauchy problem (6)-(7) has a unique solution for any (p0, w0). Moreover, if (p0, w0) 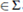their solutions (p£, m£) satisfies}}

The proof of this theorem is postponed at the end of the section. We begin with some lemmas that will be useful afterward.

Let ^{_{U = (p,m)T, H(U) = (f (U),g(U))}} and M = DF where F(p,m) =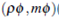.Then the system (8) can be written in the form

_{^{Ut = £Uxx + MUx + H.}}

For any ^{_{C1, C2}} constants let

G ₁ = C ₁ - W, (10)

G ₂ = Z ^- C₂, (11)

where W, Z are the Riemann invariants given in (4). We proof that the region

= {(p,m) : G ₁ 0, G ₂ 0} (12)

is an invariant region.

Lemma 2.2. If P satisfies

^_Pt + = f (p, w),

with and then Moreover;

with K constant, then p(x, t) 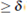(£, T) > 0 in (0, T).

For the proof of this lemma see [11, Lemma 2.2].

Lemma 2.3. ^{_{The functions G1 and G2 defined in (10) and (11), are quasi-convex}} .

Proof. Let r = (X, Y) be a vector. If 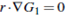then 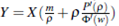thus

If , thus we have

Lemma 2.4. If the condition 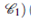holds, then G ₁ , G ₂ satisfy

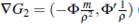Proof. From (10) and (11), we have that G1 = and then

From the Theorem 14.7 in [12], the region 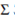defined in (12) is an invariant region for the system (8). It follows from (10), (11) that

Therefore,

we appropriately choose C ₁ , C ₂ such that

By (13) we have the proof of Proposition 2.1.

3 Weak convergence

In this section we show that the sequence (p ^£, m ^£) has a subsequence that converges the weak solutions to the system (8). For this we consider the following entropy-entropy flux pairs construct in ² by the author

The Hessian matrix of n is given by

then we have that

where X = ( ^{_{px, mx}} ). If 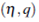is an entropy-entropy flux pair, multiplying in (8) by 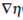(p, m) we have

Replacing the equation (14) in (15) we have

Chose a function 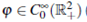satisfying 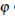= 1 on [- L, L ] x [0, T]. Multiplying (16) by 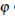and integrate the result in 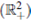we have

From the Proposition 2.1 we have

As a consequence of the inequality (17) we have the following lemma.

Lemma 3.1. For any £ > 0, if(p, m) is a solutions to the Cauchy problem (8), (9), then 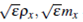are bounded in .

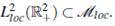We denote by 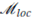the space of Radon Measures. For any bounded set 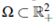we have

when £ -> 0.

Thereby,

Lemma 3.2.

are compact inParticularly

are compact in l

Proof. The proof of (21) is a consequence of the Lemma 3.1 and the inequalities (18), (19) and the Murat's Lemma. Multiplying in (20) by g' we have

By a similar argument in the inequality (18) we have

From the Lemma 3.1 the last term in (22) is in. 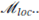By the Murat's Lemma we conclude the proof of (20).

Now we can stablish the proof of the main theorem. According to the Young's measures, there exists a probability measure ^_vxt associated with the bounded sequence ( ^{_{p£, w£}} ) such that for almost (x, t), ^_vxt satisfies the following Tartar equation,

for any entropy-entropy flux pair, where 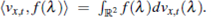We consider the following entropy-entropy flux pairs

Notice that = 0, then we have that

where 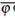denotes the weak-star limit w * - lim 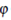( ^_u£ ). Therefore

Using the strong convergence of ^_p£ and Ф ( ^_w£ ) we have

The relation (23) includes the pointwise convergence of ^_w£ if p > 0.

4 Conclusion

This paper deals with a 2 x 2 inhomogeneous system of conservation laws of non-symmetric type, we extended the results of Lu ² under adecuate conditions on the source term.