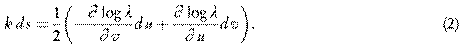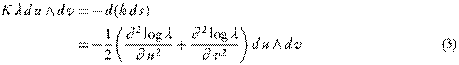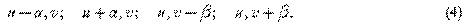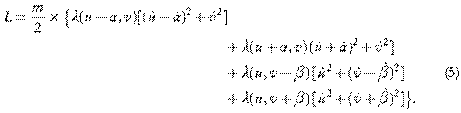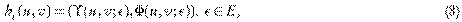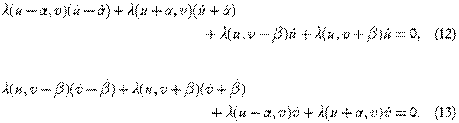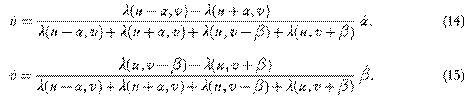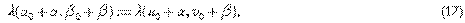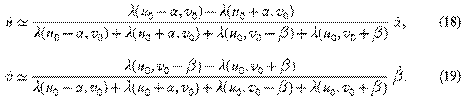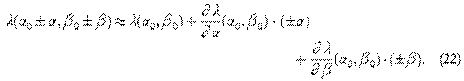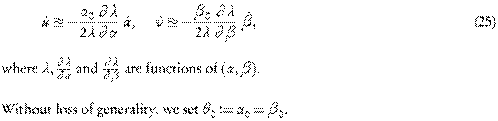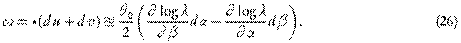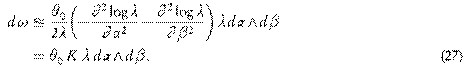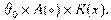Introduction

In a Euclidean inertial plane a body initially at rest remains at rest until a open access disturbing force makes it leave the rest. Nonetheless, in a curved surface, a deformable object can experience a finite amount of translation as a result of cyclic deformations arising from internal forces caused by muscles, motors o other means of shape alteration (^{Wisdom 2003}). In brief, the body is said to swim in the curved surface. The effective motion does not violate the law of conservation of linear momentum but, rather, is a genuine generalization of this law. The phenomenon reveals the existence of certain gauge fields on the configuration space for such an object or system (^{Shapere & Wilczek 1989}, ^{Littlejohn & Reinsch 1997}). The net translation does not depend on the speed with which the shape changes but on the non-null Gauss curvature of the surface. That is to say, the swimming phenomenon becomes ultimately geometric (^{Avron & Kenneth 2006}).

Although the existence of net translation from rest has been rigorously established in different contexts, its dependency on the Gauss curvature demands supplementary research efforts. Doubtlessly, the swimming motion “depends on the intrinsic curvature of the manifold” (^{Wisdom 2003}). In ^{Avron & Kenneth (2006)} it has en passant been showed that “for a small swimmer, the swimming distance in one stroke is determined by the Riemann curvature times certain moments of the swimmer”. This suggests that, in the best possible scenario, the effective translation would be directly proportional to the surface curvature and that is certainly what happens in a 2-sphere (^{Blau 2003}). However, this is not true for more general 2-manifolds (Avron & Kenneth 2006, Blau 2003). Does the direct proportionality hold at least within a reasonable approximation? We will below prove that this question has an affirmative answer.

Diverse mathematical machinery has been employed to describe the swimming phenomenon: embedded submanifolds of a real coordinate space Rⁿ (Shapere & Wilczek, ^{Blau 2003}), complex variables techniques (^{Cherman et al. 2000}), generalized coordinates or abstract Riemannian n-manifolds (^{Avron & Kenneth 2006}), among others. Here we limit ourselves to the simplest situation: a Riemannian surface, i.e., a real 2-manifold furnished with a smooth metric tensor or first fundamental form. Besides, our approach is intrinsic, meaning that the surface is not considered as lying in a Euclidean space or other ambient space. Arguments from physics should, into the bargain, be crystal clear for the mathematician. The variational principle of least action becomes here, without difficulties, a postulate. Noether’s Theorem matches a mathematical theorem. We shall assume no further knowledge on Lagrangian mechanics. Notions such as configuration space, shape space, gauge potential, gauge convention and others constitute legitimate definitions.

First we define an appropriate configuration space in a curved surface, a deformable body (lizard) and a shape space, that is, a set of shape coordinates (^{Littlejohn & Reinsch 1997}) for our problem. We also compute the velocities of the particles forming the lizard as it performs certain cyclic motion. Then, we obtain the Lagrangian of the dynamical system and deal with the consequences of the principle of least action. Needless to say, Noether’s Theorem provides a mighty tool for exploiting the local symmetries of the configuration space. The first integrals (quantities conserved in time) of the Euler-Lagrange system supply a system of differential equations relating the configuration coordinates with the shape coordinates. The core of this paper is reached later, when we introduce some infinitesimal approximations and make explicit the gauge convention. Certainly, in order to separate the configuration variables from the shape variables in the differential system, it is necessary to make simplifications, including linear approximations to the coefficients of the first fundamental form.

With these elements, we are ready to present our findings. First of all, we give yet another proof of the existence of a finite net translation resulting from a cyclic deformation of the shape, for our particular system (lizard). Our principal result is, howbeit, the affirmative answer to the previous question concerning the dependency of such finite amount of translation on the Gaussian curvature of the surface at the point of interest. Videlicet, under reachable simplifications, the translation is directly proportional to the intrinsic curvature.

Gauge kinematics

A configuration space is, for our purposes, a way to visualize the “state” of a special system as a point in a Riemannian 2-manifold. In such manner, our configuration space will specifically be an open subset of V of a Riemannian 2-manifold equipped with isothermal coordinates (u, v). ^{Gauss (1822)} proved the existence of such a local coordinate system within the frame of a general theory on the the conformal representation of one surface upon other. Dealing with a “small swimmer” only involves local coordinates in a “small” neighborhood of a point in the surface.

Isothermal coordinates on the Riemannian surface V are local coordinates whose metric is conformal to the Euclidean metric. The metric form is thus given by

where λ= λ (u, v) > 0 is the sole metric coefficient. Given a curve y in V that makes constant angle with the u - (and the v -) axis, its geodesic curvature k is the intrinsic geometric quantity yielding Liouville’s formula

The value of k measures how far is y from being a geodesic. The celebrated Theorema Egregium assures then that the Gauss curvature K of a surface also remains invariant under local isometries. Furthermore, the Gauss-Bonnet differential relation builds a walkable bridge between the geodesic curvature and the Gauss curvature. For the proofs and details of these theorems on Riemannian surfaces, we refer the interested reader to Stoker (1969).

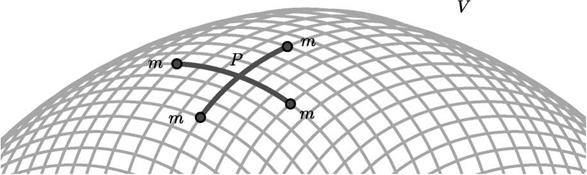
The system or lizard consists of four massless deformable limbs connected at one end in a common point P € V as it is illustrated in Fig. 1 The figure resembles a plus sign or a cross. Two limbs or legs extend sideways in opposite directions from P along a u-curve, that is, a curve for which v is a constant. The remaining limbs (neck and tail) spread out perpendicularly in opposite directions from P over a v-curve, a curve with constant u. These limbs have equal masses m attached to their free ends. The whole system is contained in V at all times, i.e. the lizard is a small swimmer. The four right angles at P are rigid (incapable of being deformed).

Figure 1 Lizard in configuration space u, v. 

We conceive muscles or other agents acting within the lizard to change the length of the limbs. While the configuration of the system is specified by the coordinates u, v, the lengths of the limbs can be regarded as shape coordinates. The shape space will constitute, for the time being, certain 2-manifold with coordinates a, (5, where a denotes the common length to the legs and ¡3 is the common length to neck and tail.

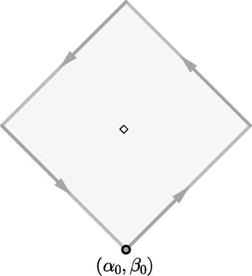
The possible values of α and β guarantee that no point of the lizard goes beyond the boundary of V. Their initial values are respectively α, β ₀ - The lizard performs a cyclic motion due exclusively to internal forces, stretching and shrinking its limbs, just like delineating a rhombus (or any other Jordan curve) in the shape space, returning finally back to the initial point. Cf.Fig. 2

Figure 2 Rhombus ❖ in shape space α, β. 

The configuration coordinates of the four masses, as functions of time, are

Gauge dynamics

A straightforward calculation of the corresponding squared velocities in terms of the metric coefficient yields the classical Lagrangian of our system or lizard:

Configuration and shape mix together in this expression. We do not have yet an explicit relation between configuration coordinates and shape coordinates (gauge convention) and so, we have yet no way to check whether or not L is a gauge invariant.

In order to determine the dynamical trajectory of a point in the lizard, we resort to the principle of least action. This is a powerful variational principle of particle and continuum systems. The Hamilton action is the real-valued functional of the trajectories from an initial to a final configuration in a specified time interval. Among all possible trajectories that the system could conceivably take, one selects (the) one that minimizes the action. Briefly, actual trajectories are those having least action. The true trajectory must, in this case, satisfy a system of differential equations, called the Euler-Lagrange equations.

Instead of attempting to solve directly these equations, we profit from some families of infinitesimal transformations on V which leave invariant the Lagrangian L. Each of such families produces a quantity whose values are conserved in time or, more precisely, a first integral of the Euler-Lagrange system. Noether’s Theorem assures the legitimacy of these “conservation laws”. Since the Lagrangian may be written in the form L = L(p,Wp), where p = (u,v) and W _p = (u,v) ∈ T _p (V) (the tangent space to V at point p), it defines a fiberwise function L : TV → M on the tangent bundle TV, into the set of real numbers M. A diffeomorphism h : V → V leaves L invariant if

where dh : TV → TV stands for the fiberwise derivative of h. Moreover, let E be an interval of real numbers containing zero, and {h _∈: ∈ ∈ E} an indexed system of diffeomorphisms of V leaving L invariant. This system is called a one-parameter family of diffeomorphisms of V when (i) the expression h _e is differentiable with respect to ∈ and (ii) h_o is the identity map on V. Under these circumstances, once we write conveniently

Noether’s Theorem ensures that the quantity

is a first integral of motion.

Now, the usual laws of conservation of energy and the angular momentum of classical mechanics are translated into relativity by postulating the existence of Killing vector fields. This will be the approach we will embrace. A Killing vector field is a vector field on the surface V that preserves the metric. That is, the Lie derivative of the metric with respect to a Killing vector field vanishes. By well-known results in ^{Atkins (2011)}, the Lie algebra of local Killing fields for a regular Riemannian surface may be one of five possible choices, including the trivial algebra. Riemannian surfaces V of non-vanishing constant Gaussian curvature, for example, are (locally) symmetric and admit three of such fields. Meaningfully, every differentiable Killing field in the neighborhood V determines a one-parameter family of diffeomorphims of V leaving the above Lagrangian invariant. Specifically, each infinitesimal transformation arising from a Killing field (ξ, ζ) implies the conservation of

Since the lizard lies initially at rest, we have that P = 0.

Assumption 1.

We assume that there are two independent Killings fields on V so that

The classification of regular Riemannian surfaces in ^{Atkins (2011}) guarantees that Assumption 1 holds whenever V is -locally- isometric to a surface of constant (positive, negative or zero) Gaussian curvature. Since these surfaces comprise parts of spheres, pseudospheres and developable surfaces, the application of our results is wide. The remaining cases demand further research. It is also important to keep in mind that we are dealing only with local, but not global, properties of differential surfaces.

In this way,

Or, put differently,

As there is no apparent general method to separate the variables, we try now to approximate this system of ordinary differential equations.

Gauge convention

A gauge convention is a manner for attaching a shape frame to a flexible body. In the case of the lizard we have implicitly supposed the existence of a bijective coordinate transformation

This will be our gauge convention. By the way, it also furnishes a Riemannian structure to the shape space via

where (u₀, v₀) ∈ V. This relation also defines an isometry between a neighborhood of (u₀, v₀) in the configuration space onto a neighborhood of (α₀, β₀) in the shape space. (u₀, v₀) may be, for instance, the point P where the lizard’s limbs join themselves together at rest before the deformation takes place.

With this in mind, we proceed to write the approximation of our nonlinear system.

Assumption 2.

There would be no harm in fixing the points (u, v) at (u₀, v₀) in such a way that

By means of the previous isometry, we are now able to separate the shape coordinates on the right side of the equations:

Immediately after that, we linearize or give a linear form to the metric coefficients through the customary first-order Taylor polynomial

We are, in such a manner, left with

Finally, we release back the shape coordinates to their possible values, i.e., we allow them to vary in the whole range by exchanging the roles of (α₀, β₀) and (α, β).

Assumption 3.

The estimated equations turn out to be

We now take advantage of the appealing language of differential forms to establish the sought amount of translation. First, we need to choose a convenient 1-form on V, that is, a smooth section of the cotangent bundle. Although for some surfaces (spheres, for example) the simple 1-form du (or dv) suffices, as an easy calculation shows, in our general setting we might be interested in the 1-form du + dv, or something of its sort. After careful consideration, we make use of the Hodge star operator to obtain an approximation to the form

The (approximation to the) exterior derivative of co can be normalized by the metric coefficient λ, that is,

By virtue of Stokes Theorem, the net finite amount of translation resulting from a deformation cycle -one stroke along a rhombus ❖ in the shape space- can then be assimilated to the integral

We summarize the main results in the following

Theorem. Let the Gauss curvature K be non-vanishing on V. Under the assumptions 1, 2 and 3, a cyclic change or stroke in the shape of the lizard (as described above) leads to a net translation. The amount of translation equals the product of θ ₀ , the area A(◊) of rhombus ◊ (in the Riemannian shape space) and the Gaussian curvature K(x) of the surface at a point x ∈ V:

The last statement is just but a forthright consequence of the Mean Value Theorem for integrals together with the fact that the configuration space and the shape space are (locally) isometric.

Concluding remarks

While there are still many questions left unanswered about the dependence of the net translation on the Gauss curvature, our aim in this paper was to establish one thing: that at least under suitable simplifying assumptions, the proportionality is direct. Moreover, we contend that studious examination of infinitesimal transformations arising from Killing fields as well as plausible isometries between the configuration space and the shape space should be carried out in order to elucidate such a dependence.

A makeshift notion of inertial frame makes sense in the cadre of intrinsic Geometry. Instead of a fixed Euclidean frame of reference attached to an origin point, it is here convenient to consider a moving frame or repère mobile on the surface. In a moving frame the linear or translational momentum retains a great deal of its significance.