1. Introduction
These definitions are needed in what follows and may or may not be familiar to everyone. A continuum X is a compact, connected metric space. A continuum X is indecomposable provided that whenever A and B are proper subcontinua of X, A ∪ B is a proper subset of X; X is hereditarily indecomposable if, and only if, every subcontinuum of X is indecomposable. A map is a continuous function. A map f from a continuum X to a continuum Y is weakly confluent provided that given any continuum M ⊆ Y there exists a continuum W ⊆ X such that f (W) = M. When X is a continuum, C(X) is the hyperspace of subcontinua of X. If a and b are points in ℝn with a ≠ b, [a,b] denotes the line segment from a to b. Let Sn denote the n dimensional sphere. An arc A ⊆ S3 is tame if and only if there is a homeomorphism h: S3 → S3 such that h(A) is an arc of a great circle in S 3 .
In [4] J. W. Rogers, Jr. asked whether every continuum is a continuous image of some indecomposable continuum. The author [1] gave an affirmative answer to this question.
Sometime later, in conversation, Rogers asked whether every continuum is a continuous image of some hereditarily indecomposable continuum. This article provides a proof that the answer to this question is also yes.
The author first announced this result in [1] but has not published it previously. It has come to my attention that in [4] this result has been extended to the non-metric case, building on the metric result.
2. Necessary Lemmas
Lemma 2.1. Let X and Y be continua. Then f: X → Y is weakly confluent if, and only if, the hyperspace map induced by f, C(f): C(X) → C(Y), is surjective.
Proof. This is just a restatement of the definition of weakly confluent.
Lemma 2.2. There exists a hereditarily indecomposable subcontinuum of ℝ 4 which separates ℝ4 .
Remark on proof. R. H. Bing [2] proved this not just for n = 4, but for every n > 1.
Lemma 2.3. Each homotopically essential map from a continuum X to the three sphere, S3 , is weakly confluent.
Proof. This was essentially proven, although in a different context, by S. Mazurkiewicz in [5, Theoreme I, p. 328]. This argument gives the necessary details. Let X be a continuum, and suppose g: X → S3 be a homotopically essential map. To prove that g is weakly confluent, it suffices to prove that every tame arc in S3 is equal to g(M) for some continuum M ⊆ X. This follows from Lemma 2.1 because the set of tame arcs is dense in C(S 3 ).
First, set up some machinery and notation, as follows. Let J be a tame arc in S3; let D n be the closed disk in the complex plane with radius (1/n) centered at 0. Let E n be the corresponding open disk, and let T n be the circle Dn \ En. Let Cn be the solid cylinder Dn x [0,1]. Since J is a tame, there exists an embedding h of C into S3 such that h({0} x [0,1]) = J. Consider Cn as a subset of S3 by identifying C1 with h(C 1 ), and for each t ∈ [0,1] let t denote the point h(0, t) ∈ J.
Let F n denote the manifold boundary of Cn , that is, F n = (Dn x {0,1}) ∪ (Tn x [0,1]). Note that given any n and any a, b ∈ J there is an isotopy H: Cn x [0,1] → Cn satisfying the following:
(i) for each s ∈ [0,1], H(J x {s}) = J;
(ii) for each x ∈ Fn and each t ∈ [0,1], H(x,t) = x;
(iii) for every x ∈ Cn, H(x, 0) = x; and
(iv) H(b, 1) = a.
By setting H(x, t) = x for every x ∈ S3 \ Cn, and every t ∈ [0, 1], H can be considered to be a function (hence an isotopy) from S3 x [0, 1] to S3.
Now, suppose X is a continuum and let g: X → S3 be a homotopically essential map. To prove that g is weakly confluent, it suffices to prove that there exists a continum M ⊆ X such that g(M) = J.
Proceed by contradiction; assume there is no such M. Then no component of g-1(J) intersects both g-1(0) and g-1(1). By compactness, there is a separation, R0 ∪ R1 of g-1(J) satisfying g-1(0) ⊆ R0 and g-1(1) ⊆ R1. Since R0 and R1 are disjoint closed sets in X, there exist open subsets S0 and S1 of X such that R0 ⊆ S0 and R1 ⊆ S1 and Cl(S0) ∩ Cl(S1) = ∅. There exists n such that g-1(Cn) ⊆ S0 ∪ S1. Let p = inf g(R1) and let q = sup g(R0), and let a, b ∈ J be such that 0 < a < p and q < b < 1. If p > q, then g is not surjective and hence not essential, so 0 <a<p ≤ q<b< 1. ∪sing the number n and the points a and b just chosen, let H: S3 x [0, 1] → S3 be the isotopy described above. Define a homotopy G: X x [0,1] → S3 by G(x, t) = g(x) if x ∈ X \ S0 and G(x,t) = H(g(x),t) if x ∈ Cl(S0). Define f: X → S3 by f (x) = G(x, 1).
Then, note that if y ∈ J and a < y < p, then there does not exist z ∈ X such that f (z) = y, so f is nonsurjective. Hence, f is inessential. Since g is homotopic to f, g is inessential also, a contradiction, which completes the proof.
Lemma 2.4. A continuum X ⊆ R4 admits a homotopically essential map onto S3 if, and only if, R4 X is not connected S3.
Remark on Proof. This is a special case of the Borsuk separation theorem. I do not have a reference to the original proof, but a proof can be found in almost any advanced topology or algebraic topology book.
Lemma 2.5. Given any continuum Y, there is a continuum X ⊆ S3 that admits a continuous surjection f : X → Y.
Proof. Let Y be a continuum and let C and D be Cantor sets in R3 such that C and D lie on lines skew to each other. Then, whenever a, p ∈ C and b, q ∈ D, and a, p, b, and q are all different, the line segments [a, b] and [p, q] are disjoint. Let g: C ∪ D → Y be a map such that g|C: C → Y and g|D: D → Y are both onto. Such a g exists since a Cantor set can be mapped onto every compact metric space. Define
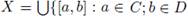 and g(a) = g(b)}. Then X is a continuum in R3. For each x ∈ X, let [a(x), b(x)] be a segment in X satisfying a(x) ∈ C; b(x) ∈ D, and x ∈ [a(x), b(x)]. (This segment is unique unless x = a(x) or x = b(x).) Define f: X → Y by f (x) = g(a(x)) = g(b(x)). It is straightforward to verify that f : X → Y is continuous and onto. Since for any point p ∈ S3, S3 \ {p} is a copy of R3, X can be treated as a subcontinuum of S3.
and g(a) = g(b)}. Then X is a continuum in R3. For each x ∈ X, let [a(x), b(x)] be a segment in X satisfying a(x) ∈ C; b(x) ∈ D, and x ∈ [a(x), b(x)]. (This segment is unique unless x = a(x) or x = b(x).) Define f: X → Y by f (x) = g(a(x)) = g(b(x)). It is straightforward to verify that f : X → Y is continuous and onto. Since for any point p ∈ S3, S3 \ {p} is a copy of R3, X can be treated as a subcontinuum of S3.
3. Main Result
Theorem 3.1. Let Y be an arbitrary continuum. There exists a hereditarily indecomposable continuum K that admits a surjective map f: K → Y.
Proof. Let Y be a continuum. By Lemma 2.5, there is a continuum T ⊆ S3 and an onto map g : T → Y. By Lemma 2.2, there exists a hereditarily indecomposable continuum L ⊆ R4 that separates R4. Thus by Lemma 2.4, there is a homotopically essential map h: L → S3. By Lemma 2.3, h is weakly confluent, so there exists a continuum K C L such that h(K) = T. Let f = g o (h|K). Then f: K → Y is the desired map; K is hereditarily indecomposable since it is a subcontinuum of L.















