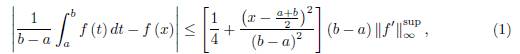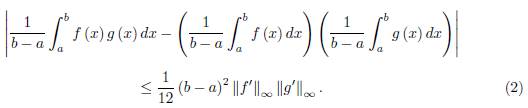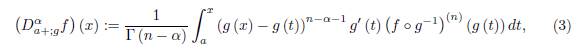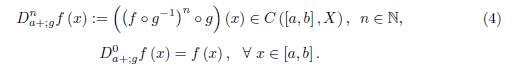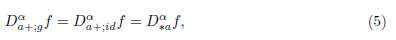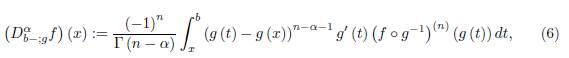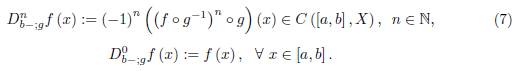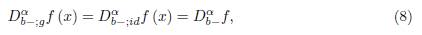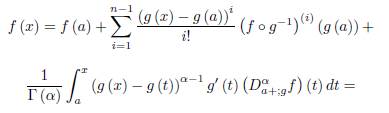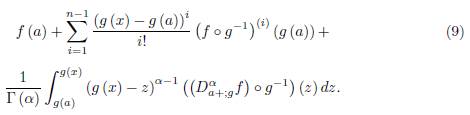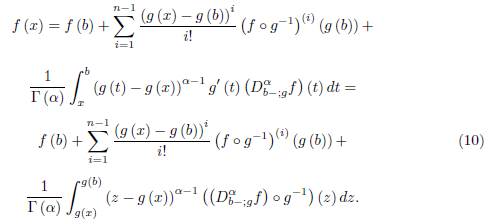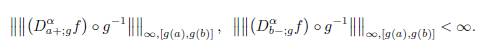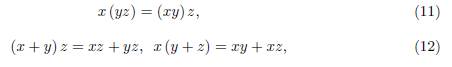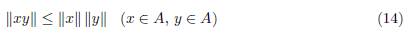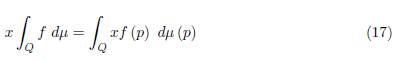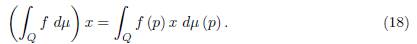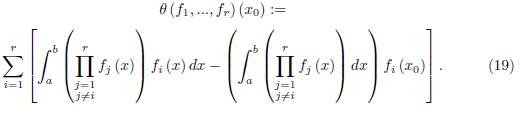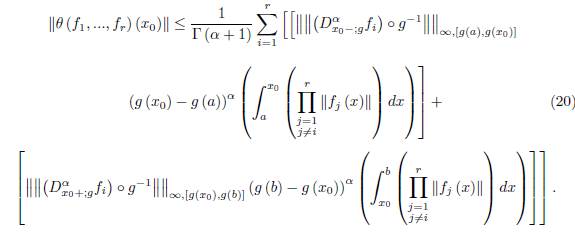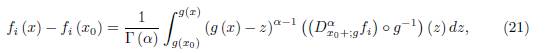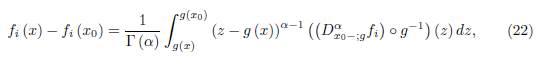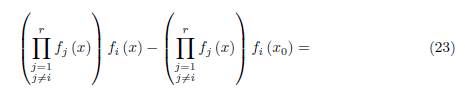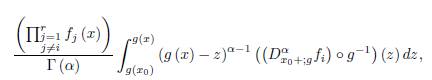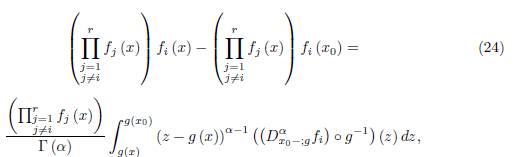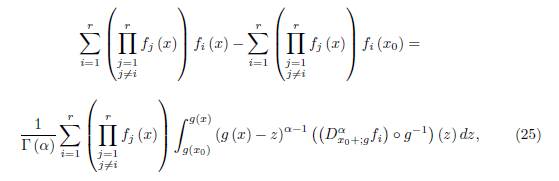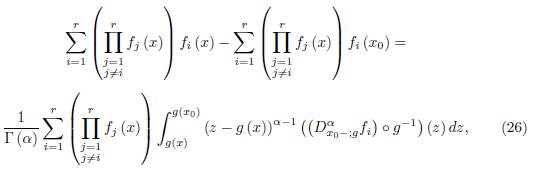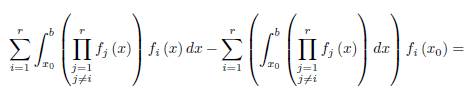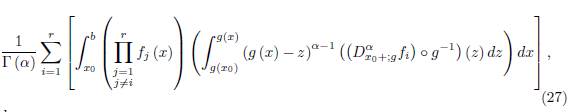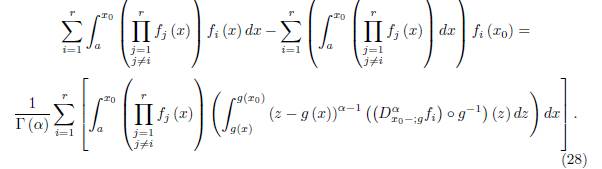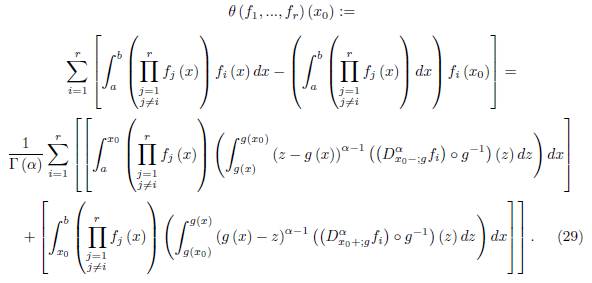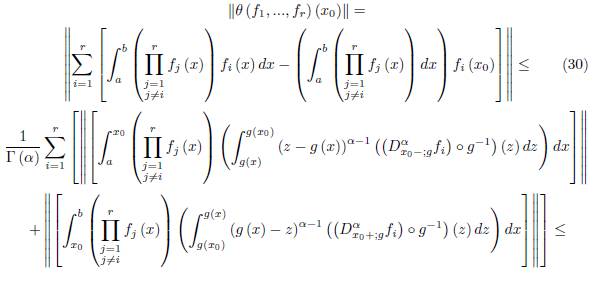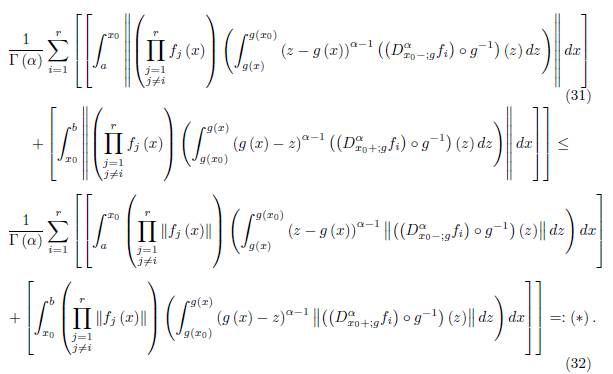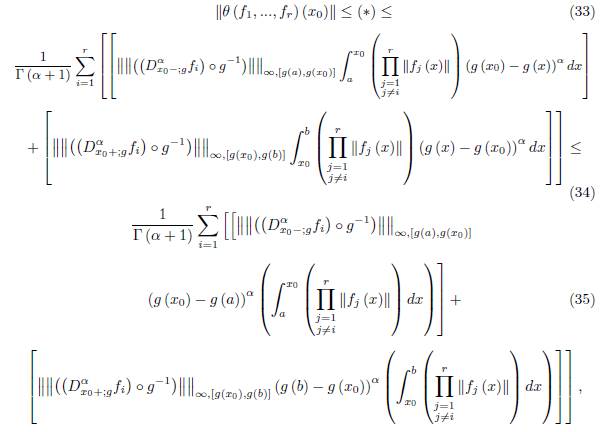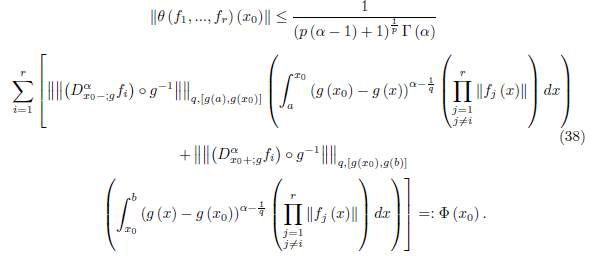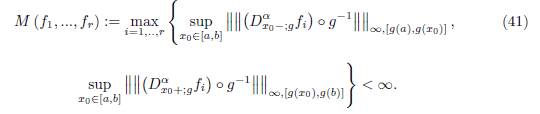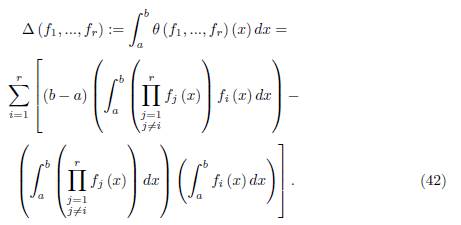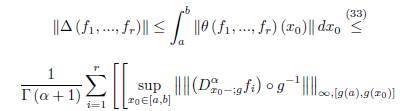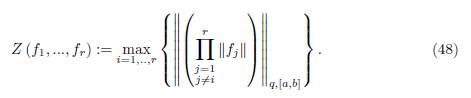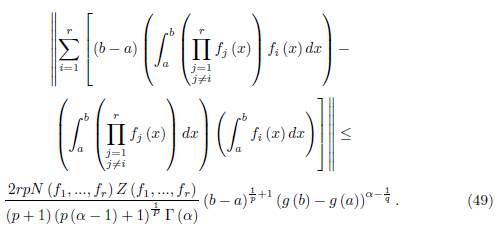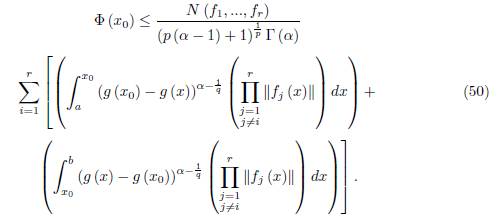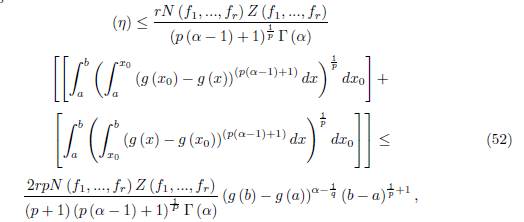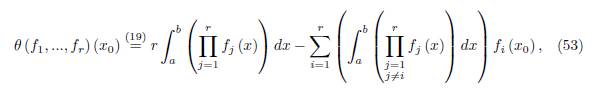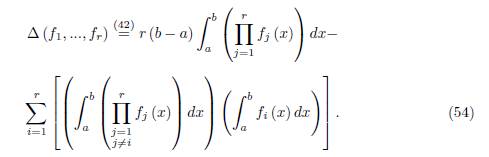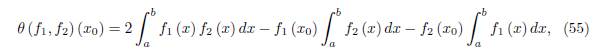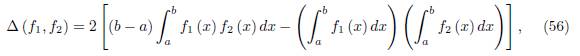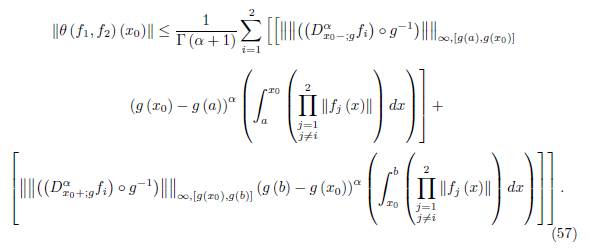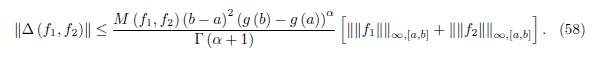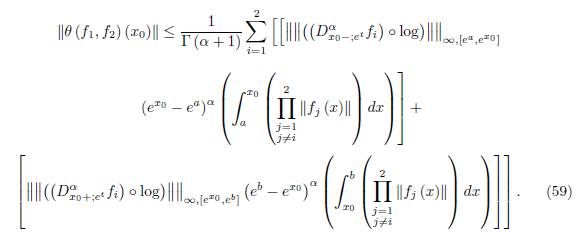1. Introduction

The following results motivate our work.

Theorem 1.1 (1938, Ostrowski [¹⁰]). Let f : [a, b] → ℝ be continuous on [a, b] and differentiable on (a, b) whose derivative f′ : (a, b) → ℝ is bounded on (a, b), i.e., 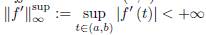. Then

for any x ∈ [a, b]. The constant 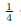is the best possible.

Ostrowski type inequalities have great applications to integral approximations in Numerical Analysis.

Theorem 1.2 (1882, Čebyšev [⁶]). Let f, g : [a, b] → ℝ be absolutely continuous functions with f^′, g^′ ∈ L_∞ ([a, b]). Then

The above integrals are assumed to exist.

The related Grüss type inequalities have many applications to Probability Theory. We presented also ([⁴], Ch. 8,9) mixed fractional Ostrowski and Grüss-Cebysev type inequalities for several functions, acting to all possible directions. The estimates involve the left and right Caputo fractional derivatives. See also the monographs written by the author [²], Chapters 24-26 and [³], Chapters 2-6.

In this article we generalize [⁴], Ch. 8,9 for several Banach algebra valued functions. Now our left and right Caputo fractional derivatives are for Banach space valued functions and our integrals are of Bochner type. Several applications finish this article. Inspiration came also from [⁷], [⁸].

2. Vectorial background fractional calculus

Here all come from [⁵]. We need:

Definition 2.1 ([⁵], p. 106). Let α > 0, ⌈α⌉ = n, ⌈·⌉ the ceiling of the number. Let f ∈ Cⁿ ([a, b] , X), where [a, b] ⊂ ℝ, and (X, ∥·∥) is a Banach space. Also let g ∈ C¹ ([a, b]) , strictly increasing such that g⁻¹ ∈ Cⁿ ([g (a) , g (b)]) .

We define the left generalized g-fractional derivative X-valued of f of order α as follows:

∀ x ∈ [a, b], where Γ is the gamma function. The last integral is of Bochner type ([¹], pp. 422-428; [⁹]).

If α , by Theorem 4.10 ([⁵], p. 98), we have that .

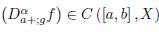We set

When g = id, then

the usual left X-valued Caputo fractional derivative, see [⁵], Ch. 1.

We also need:

Definition 2.2 ([⁵], p. 107). Let α > 0, ⌈α⌉ = n, · ⌈・⌉ the ceiling of the number. Let f ∈ Cⁿ ([a, b] , X), where [a, b] ⊂ ℝ, and (X, ∥・∥) a Banach space. Let g ∈ C¹ ([a, b]) , strictly increasing such that g⁻¹ ∈ Cⁿ ([g (a) , g (b)]) .

We define the right generalized g-fractional derivative X-valued of f of order α as follows:

∀ x ∈ [a, b]. The last integral is of Bochner type.

If α , by Theorem 4.11 ([⁵], p. 101), we have that

We set

When g = id, then

the usual right X-valued Caputo fractional derivative, see [⁵], Ch. 2.

We mention the following generalized fractional Taylor formulae with integral remainders over Banach spaces.

Theorem 2.3 ([⁵], p. 107). Let α > 0, n = ⌈α⌉, and f ∈ C ⁿ ([a, b] , X), where [a, b] ⊂ ℝ and (X, · ) a Banach space. Let g ∈ C¹ ([a, b]), strictly increasing such that g⁻¹ ∈ Cⁿ ([g (a) , g (b)]), a ≤ x ≤ b. Then

We also mention:

Theorem 2.4 ([⁵], p. 108). Let α > 0, n = ⌈α⌉, and f ∈ C ⁿ ([a, b] , X), where [a, b] ⊂ ℝ and (X, ∥·∥) a Banach space. Let g ∈ C¹ ([a, b]), strictly increasing such that g⁻¹ ∈ Cⁿ ([g (a) , g (b)]), a ≤ x ≤ b. Then

If 0 < α ≤ 1, then the sums in (9), (10) disappear.

Also in (9), (10), we have that

3. Banach Algebras background

All here come from [¹¹].

We need:

Definition 3.1 ([¹¹], p. 245). A complex algebra is a vector space A over the complex filed C in which a multiplication is defined that satisfies

and

for all x, y and z in A and for all scalars α.

Additionally if A is a Banach space with respect to a norm that satisfies the multiplicative inequality

and if A contains a unit element e such that

and

then A is called a Banach algebra.

A is commutative iff xy = yx for all x, y ∈ A.

Remark 3.2. Commutativity of A will be explicited stated when needed.

There exists at most one e ∈ A that satisfies (15).

Inequality (14) makes multiplication to be continuous, more precisely left and right continuous, see [¹¹], p. 246.

Multiplication in A is not necessarily the numerical multiplication, it is something more general and it is defined abstractly, that is for x, y ∈ A we have xy ∈ A, e.g. composition or convolution, etc.

For nice examples about Banach algebras see [¹¹], p. 247-248, S (Λ) 10.3.

Remark 3.3. Next we mention about integration of A-valued functions, see [¹¹], p. 259, S (Λ) 10.22:

If A is a Banach algebra and f is a continuous A-valued function on some compact Hausdorff space Q on which a complex Borel measure µ is defined, then f dµ exists and has all the properties that were discussed in Chapter 3 of [¹¹], simply because A is a Banach space. However, an additional property can be added to these, namely: If x ∈ A, then

and

The Bochner integrals we will involve in our article follow (17) and (18). Also, let f ∈ C ([a, b] , X), where [a, b] ⊂ ℝ, (X, · ) is a Banach space. By [⁵], p. 3, f is Bochner integrable.

4. Main Results

We start with mixed generalized fractional Ostrowski type inequalities for several functions over a Banach algebra. A uniform estimate follows.

Theorem 4.1. Let (A, ∥・∥) be a Banach algebra, x₀ ∈ [a, b] ⊂ ℝ, α > 0, n = ⌈α⌉, fi ∈ Cⁿ ([a, b] ,A), i = 1, ..., r ∈ ℕ − {1}; g ∈ C¹ ([a, b]), strictly increasing such that g⁻¹ ∈ Cⁿ ([g (a) , g (b)]), with (fi ◦ g⁻¹ ) ^(k) (g (x₀)) = 0, k = 1, ..., n − 1; i = 1, ..., r.

Denote by

Then

Proof. Since (f_i ◦ g⁻¹) ^(k) (g (x₀)) = 0, k = 1, ..., n − 1; i = 1, ..., r, we have by Theorem 2.3 that

∀ x ∈ [x₀, b] ,

and by Theorem 2.4 that

∀ x ∈ [a, x₀]

for all i = 1, ..., r.

Left multiplying (21) and (22) with 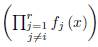we get, respectively,

∀ x ∈ [x₀, b] ,

and

∀ x ∈ [a, x₀] ;

for all i = 1, ..., r.

∀ x ∈ [x₀, b] ,

and

∀ x ∈ [a, x₀] .

Next we integrate (25) and (26) with respect to x ∈ [a, b]. We have

and

Finally, adding (27) and (28) we obtain the useful identity

Therefore we get that

Hence it holds

proving (20).

Next comes an L₁ estimate.

Theorem 4.2. All as in Theorem 4.1, with α ≥ 1. Then

proving Theorem 4.2.

An Lp estimate follows.

Theorem 4.3. All as in Theorem 4.1. Let now p, q > 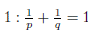, with α > 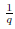. Then

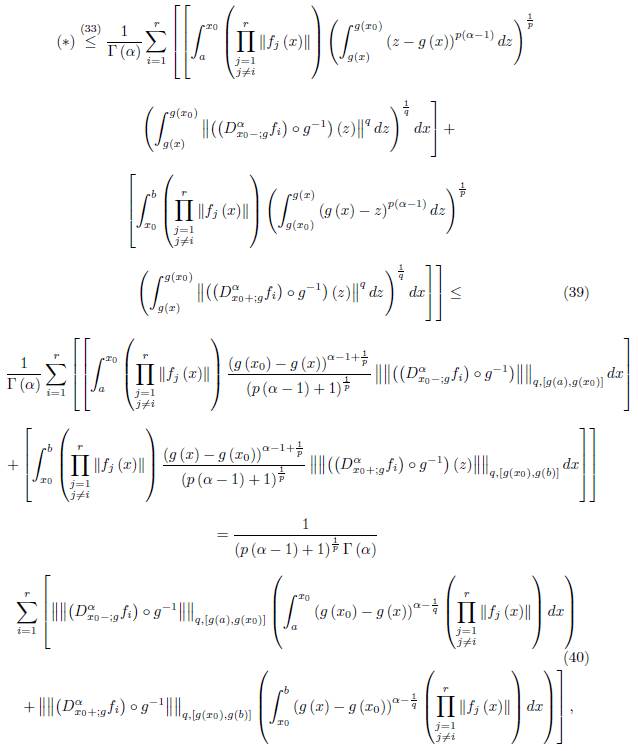
Proof. We have that

proving Theorem 4.3.

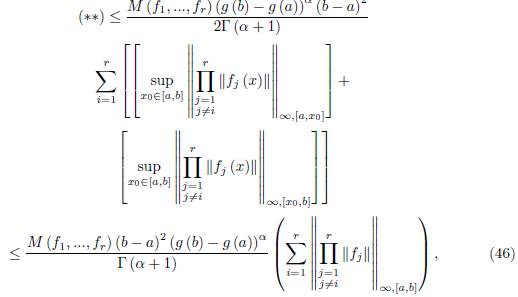
We continue with generalized fractional Grüss-Cebysev type inequalities for several functions over a Banach algebra. A uniform estimate follows.

Theorem 4.4. Let (A, ∥·∥) be a Banach algebra, 0 < α ≤ 1, f_i 1, ..., r ∈ ℕ − {1}; g ∈ C¹ ([a, b]), strictly increasing such that g⁻¹ x₀ ∈ [a, b] ⊂ ℝ and θ (f₁, ..., f_r) (x₀) as in (19). Assume that

Denote by

Then it holds

Proof. We have that

Hence it holds

proving (43).

Next comes an L_p estimate

Theorem 4.5. Let (A, ∥·∥) be a Banach algebra, 0 < α ≤ 1, f_i 1, ..., r ∈ ℕ − {1}; g ∈ C¹ ([a, b]), strictly increasing such that g⁻¹ Let p, q > 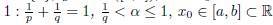. Assume that

and set

Then

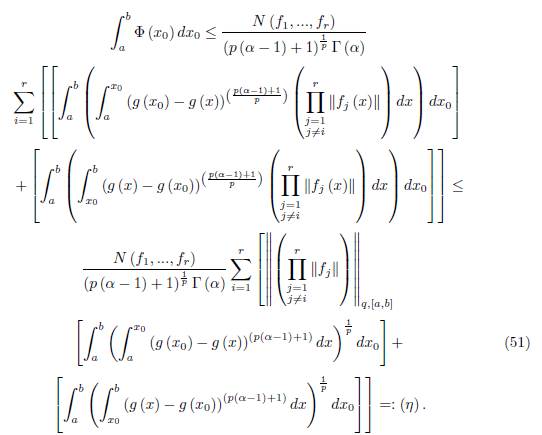
Proof. Here Φ (x₀) is as in (38).

Clearly then it holds

Therefore we obtain

Hence we get

proving (49).

5. Applications

Remark 5.1. Assume from now on that (A, ∥·∥) is a commutative Banach algebra. Then, we get that

x₀ ∈ [a, b] .

Furthermore, it holds (0 < α ≤ 1 case)

When r = 2, we get that

x₀ ∈ [a, b] ,

and

0<α≤1.

Corollary 5.2. All as in Theorem 4.1, A is a commutative Banach algebra, r = 2. Then

Proof. By Theorem 4.1.

We continue with

Corollary 5.3. All as in Theorem 4.4, A is a commutative Banach algebra, r = 2, 0 < α≤ 1, M (f₁, f₂) as in (41). Then

Proof. By Theorem 4.4.

Finally we derive:

Corollary 5.4. All as in Corollary 5.2, for g (t) = e^t. Then

Proof. By Corollary 5.2.